Pulley project of mine requiring help

[kieyard]

text is same as on pic.

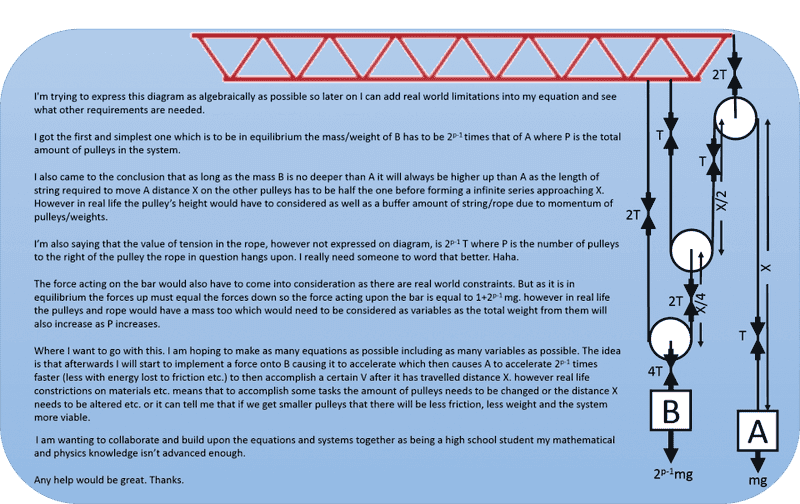
I'm trying to express this diagram as algebraically as possible so later on I can add real world limitations into my equation and see what other requirements are needed.

I got the first and simplest one which is to be in equilibrium the mass/weight of B has to be 2p-1 times that of A where P is the total amount of pulleys in the system.

I also came to the conclusion that as long as the mass B is no deeper than A it will always be higher up than A as the length of string required to move A distance X on the other pulleys has to be half the one before forming a infinite series approaching X.

However in real life the pulley’s height would have to considered as well as a buffer amount of string/rope due to momentum of pulleys/weights.

I’m also saying that the value of tension in the rope, however not expressed on diagram, is 2p-1 T where P is the number of pulleys to the right of the pulley the rope in question hangs upon. I really need someone to word that better. Haha.

The force acting on the bar would also have to come into consideration as there are real world constraints. But as it is in equilibrium the forces up must equal the forces down so the force acting upon the bar is equal to 1+2p-1 mg. however in real life the pulleys and rope would have a mass too which would need to be considered as variables as the total weight from them will also increase as P increases.

Where I want to go with this. I am hoping to make as many equations as possible including as many variables as possible. The idea is that afterwards I will start to implement a force onto B causing it to accelerate which then causes A to accelerate 2p-1 times faster (less with energy lost to friction etc.) to then accomplish a certain V after it has traveled distance X. however real life constrictions on materials etc. means that to accomplish some tasks the amount of pulleys needs to be changed or the distance X needs to be altered etc. or it can tell me that if we get smaller pulleys that there will be less friction, less weight and the system more viable.

I am wanting to collaborate and build upon the equations and systems together as being a high school student my mathematical and physics knowledge isn’t advanced enough.

Any help would be great. Thanks.

 

[eljose79]

Hello! It sounds like you are trying to develop a mathematical model for a system involving multiple pulleys and masses. This is a very interesting and challenging problem, and I would be happy to offer some suggestions and insights.

First, it is important to understand that in order to accurately model a real-world system, we must consider all of the relevant forces and constraints. This means that in addition to the weight of the masses and the tension in the rope, we must also consider the mass and dimensions of the pulleys, as well as any frictional forces that may be present.

Based on your description, it seems like you have already identified some of these factors and incorporated them into your equations. However, I would caution against assuming that the tension in the rope is always equal to 2p-1 T. This may be true in certain situations, but it could also vary depending on the specific configuration of the system and the forces acting on it. It may be more accurate to consider the tension as a variable that is dependent on the other factors in the system.

You also mentioned the concept of equilibrium, which is crucial in understanding how the forces in this system interact. In order for the system to be in equilibrium, the forces acting on each pulley and mass must be balanced. This means that the net force in each direction (up and down) must be equal. This can be expressed mathematically as:

ΣFup = ΣFdown

Where ΣF represents the sum of all the forces in each direction. This equation can be used to solve for the tension in the rope, as well as the acceleration of the masses.

In terms of incorporating real-world limitations, one approach could be to introduce a factor for efficiency or energy loss into your equations. This could account for the friction and other factors that may affect the performance of the system. You could also consider adding variables for the material properties of the pulleys and rope, such as their coefficients of friction or elasticity.

Overall, it seems like you have a solid understanding of the basic principles involved in this system and are on the right track in terms of developing a mathematical model. I would recommend continuing to refine and expand upon your equations, and perhaps seeking guidance from a teacher or mentor who has experience with this type of problem. Good luck with your project!