member 731016
- Homework Statement
- Pls see below
- Relevant Equations
- Pls see below
For this problem,
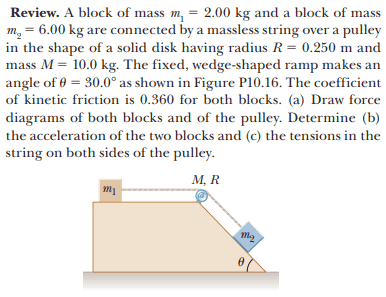
Why is the tension on each side not equal?
For this problem I think the only assumption is that the string is inextensible so the accelerations of the masses are equal.
Many thanks!
Why is the tension on each side not equal?
For this problem I think the only assumption is that the string is inextensible so the accelerations of the masses are equal.
Many thanks!