Q： Why is it a reversible adiabatic process but not isentropic?

[tracker890 Source h]

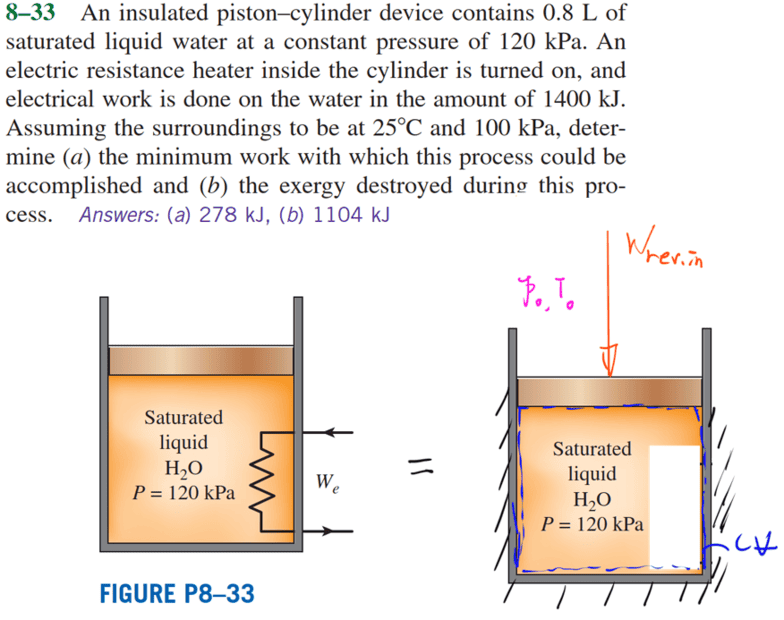
Homework Statement

Puzzled by why it's a reversible adiabatic process but not isentropic.

Relevant Equations

Wb,rev equation

Q1: Why can't set $Q_{in,net}=0$ and use equation (2) to obtain $W_{act,in}=\left( \bigtriangleup U \right) _{cv}$?
Q2: If assume it's a reversible process, why can't equation (3) determine (△S)sys=0?
ref1.
ref2.

$$
W_{rev,in}=W_{act,in}-W_{surr}=W_{act,in}+P_0\left( V_2-V_1 \right) \cdots \text{(1)}
$$
$$
\ \ Q_{in,net}+W_{act,in}=\left( \bigtriangleup U \right) _{cv}\cdots \text{(2)}
$$
$$
\ \ T_0\left( \bigtriangleup S \right) _{sys}=\sum{\left( \frac{T_0}{T_k} \right)}Q_k\cdots \text{(3)}
$$
$$
\ let\ \ Q_{in,net}=Q_k
$$
$$
\left( 2 \right) +\left( 3 \right) :\
$$
$$
\ \ W_{act,in}=\sum{\left( -1+\frac{T_0}{T_k} \right)}\cancel{Q_k}+\left( \bigtriangleup U \right) _{cv}-\ T_0\left( \bigtriangleup S \right) _{sys}
$$
$$
\ \ \ \ \ \ \ \ =\left( U_2-U_1 \right) -T_0\left( S_2-S_1 \right) _{sys}\cdots \text{(4)}
$$
$$
Substitute\left( 4 \right) into\left( 1 \right)
$$
$$
W_{rev,in}=\left( U_2-U_1 \right) -T_0\left( S_2-S_1 \right) _{sys}+P_0\left( V_2-V_1 \right)
$$
$$
=m\left[ \left( u_2-u_1 \right) -T_0\left( s_2-s_1 \right) _{sys}+P_0\left( v_2-v_1 \right) \right]
$$
$$
=-m\left[ \left( u_1-u_2 \right) -T_0\left( s_1-s_2 \right) _{sys}+P_0\left( v_1-v_2 \right) \right] \cdots \text{(5)}
$$

 

[Chestermiller]

I don't understand your notation or what you did.

Here is my take on this: If the surroundings are at 100 kPa, and the saturated liquid water is at 120 kPa, that must mean that the weight of the piston must contribute 20 kPa. At 120 kPa, the specific volume of water from the steam tables is 1.047 liters/kg. So you have 0.8/1.047=0.764 kg of water in your system. The heat of vaporization at 120 kPa is 2247 kJ/kg. So it would take 1717 kJ to vaporize all the water. You are only adding 1400 kj, so that would vaporize 81.5% of the water, so, in the final state, you would have (0.764)(0.815)=0.623 kg water vapor and 0.141 kg saturated liquid water in the cylinder at 120 kPa and about 105 C.

I don't understand what they mean by "the minimum amount of work with which this process could be accomplished."

 

[tracker890 Source h]

I don't understand your notation or what you did.

Here is my take on this: If the surroundings are at 100 kPa, and the saturated liquid water is at 120 kPa, that must mean that the weight of the piston must contribute 20 kPa. At 120 kPa, the specific volume of water from the steam tables is 1.047 liters/kg. So you have 0.8/1.047=0.764 kg of water in your system. The heat of vaporization at 120 kPa is 2247 kJ/kg. So it would take 1717 kJ to vaporize all the water. You are only adding 1400 kj, so that would vaporize 81.5% of the water, so, in the final state, you would have (0.764)(0.815)=0.623 kg water vapor and 0.141 kg saturated liquid water in the cylinder at 120 kPa and about 105 C.

I don't understand what they mean by "the minimum amount of work with which this process could be accomplished."

My question is quite simple, why is the entropy change not zero for this reversible adiabatic process?
$\left( s_1-s_2 \right) _{sys}\ne 0$

 

[Chestermiller]

My question is quite simple, why is the entropy change not zero for this reversible adiabatic process?
$\left( s_1-s_2 \right) _{sys}\ne 0$

Oh, that's easy. If your system includes the heater plus the water, then entropy is generated in the heater (resistance). If your system includes the water only, then the water is not adiabatic, but the entropy change is equal to the heat from the heater divided by the constant water temperature. Basically, the entropy generated in the heater is transferred to the water.

So for the combination of water plus heater, the process is adiabatic but irreversible. For the water alone, it undergoes a non-adiabatic reversible change. For the heater alone, it generates entropy that is transferred to the water.

 

[tracker890 Source h]

Oh, that's easy. If your system includes the heater plus the water, then entropy is generated in the heater (resistance). If your system includes the water only, then the water is not adiabatic, but the entropy change is equal to the heat from the heater divided by the constant water temperature. Basically, the entropy generated in the heater is transferred to the water.

So for the combination of water plus heater, the process is adiabatic but irreversible. For the water alone, it undergoes a non-adiabatic reversible change. For the heater alone, it generates entropy that is transferred to the water.

$Q_k=0$, but why is $\left( \bigtriangleup S \right) _{sys}$ not equal to zero in equation (3)?

 

[Chestermiller]

$Q_k=0$, but why is $\left( \bigtriangleup S \right) _{sys}$ not equal to zero in equation (3)?

Are you aware that entropy can change not only by heat flow at the boundaries of a system, but also by entropy generation due to irreversibility? Eqn. 3 does not include entropy generation.

$$\Delta S_{heater}=-\frac{Q}{378}+\sigma=0$$where Q is the heat transferred from the heater to the water and $\sigma$ is the entropy generated within the heater as a results of irreversibility (ohmic resistance).
$$\Delta S_{water}=+\frac{Q}{378}$$
$$\Delta S_{system}=\Delta S_{heater}+\Delta S_{water}=\sigma=\frac{Q}{378}>0$$

 

[tracker890 Source h]

Are you aware that entropy can change not only by heat flow at the boundaries of a system, but also by entropy generation due to irreversibility? Eqn. 3 does not include entropy generation.

$$\Delta S_{heater}=-\frac{Q}{378}+\sigma=0$$where Q is the heat transferred from the heater to the water and $\sigma$ is the entropy generated within the heater as a results of irreversibility (ohmic resistance).
$$\Delta S_{water}=+\frac{Q}{378}$$
$$\Delta S_{system}=\Delta S_{heater}+\Delta S_{water}=\sigma=\frac{Q}{378}>0$$

But isn't the question asking for reversible work? So, doesn't that mean we should assume $S_{gen}=0$, and when $S_{gen}=0,Q_k=0$, which implies that $\left( \bigtriangleup S \right) _{sys}$ should also become 0?

 

[Chestermiller]

But isn't the question asking for reversible work? So, doesn't that mean we should assume $S_{gen}=0$, and when $S_{gen}=0,Q_k=0$, which implies that $\left( \bigtriangleup S \right) _{sys}$ should also become 0?

I don't understand what the question is asking for. All I know is that the process as described in the problem statement is irreversible (for the overall system), as you yourself correctly ascertained by the fact that the entropy change is positive while no heat is transferred to or from the system.

 

[tracker890 Source h]

I don't understand what the question is asking for. All I know is that the process as described in the problem statement is irreversible (for the overall system), as you yourself correctly ascertained by the fact that the entropy change is positive while no heat is transferred to or from the system.

Thank you for your patient and detailed explanation. I will try to understand it first.

 

[Chestermiller]

Thank you for your patient and detailed explanation. I will try to understand it first.

There are two ways that the Exergy destruction can be determined for the system (water and heater) involved in this process.

Method 1:

1. Calculate the Exergy change of the system $\Delta E$
2. Recognize that, since the system is insulated, the exergy transfer between system and surroundings due to heat flow is zero
3. Calculate the exergy transfer due to work: $-[W-p_0(V_2-V_1)$
4. Subtract the exergy transfer due to work from the overall exergy change of the system. This gives minus the exergy destruction.

Method 2:

1. Determine the entropy change of the combined system (heater + water). This is equal to the entropy change of the water alone, since the entropy change of the heater is zero. The entropy change of the water is equal to the heat supplied by the heater divided by the system temperature (since the water experiences an internally reversible change).
$$\Delta S_{water}=\frac{1400}{378}=3.70\ kJ/K$$

2. Recognize that this is also equal to the entropy generated in the combined system, since the combined system is isolated: $\sigma=\Delta S_{water}$

3. Calculate the exergy destruction form $destruction=T_0\sigma$