Inqur
- 1
- 0
- TL;DR Summary
- Can the quantum nature of reality be based on a spacial matrix function rather than the probability function of QED/Quantum Mechanics?
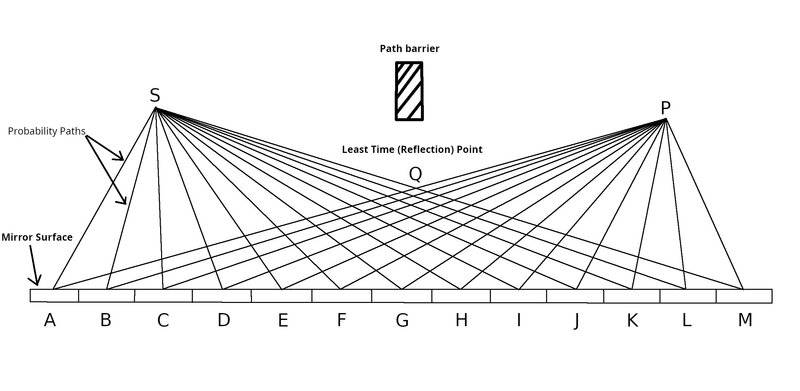
In "QED, The Strange Theory of Light And Matter" Richard Feynman describes the probability path of a single photon emitted from a source, reflected from a mirror surface, and finally reflected to a probe detector. This path is the least time path relative to the probe/viewer, determined by the phasor angle of the time/probability path. See diagram below. Notations added.
As we all know from experience, the detection point is determined wherever the probe/viewer is stationed. Therefore, in principle, the number of possible detection points are infinite in spacial locations, viz., an infinite number of the least time paths are possible. The orthodox particle theory of light would negate such a possibility, but the wave nature of light would seem to allow it. Of course, such detections by eye are a result of many emissions of photons.
But a question arises that, in theory, if we could set up an infinite number of different probes would we detect the same "one" photon at all the infinite probe locations "simultaneously" (ignoring the spatial distance of time delay of the different locations), or is this detection dependent on the finite quantum packet of the photon "particle" and therefore detectable by only one probe? Presumably then, the shortest least action path would be that detection point. Although, this could also possibly be a wave function of a finite quantum packet of the photon.
Has a similar experiment ever been conducted?
However, it is odd that the experiment is conducted under the assumption that reflection occurs as a wave function phenomenon, given that a mirror surface is a reflector of light waves, which can therefore be predictably determined by the least time path, and disregarding a particle probability consideration. The least action path, relative to reflection paths, seems contradictory to the particle paradigm. It also seems to unnecessarily complicate the understanding of Nature by probability functions. Feynman himself concedes this Strange conundrum in the very title of his book. A more sensible paradigm would be that a Spacial matrix is the actual function of "motion", energy, and matter themselves, albeit, one that cannot presently be defined by orthodox science. It is true that the equations in the QED and Quantum Mechanics models are highly precise in their predictions, but this would be expected, given that the elements of their equations are based on known experimental constants and extrapolated therefrom; viz. that such predictions are simply mathematical logical extensions. The same can be said of particle theory creation via collider experiments, thereby creating mathematical "phantom" particles that normally have no reality in Nature, except as logical extensions of synthetic conditions. Similarly, in Quantum Mechanics, the Spacial Matrix concept would eliminate the paradox of an interference pattern creation in the two slit experiment created by a series of single shot electrons. And the use of probes to detect which slit the electron emerges from is nonsensical, since it introduces a type of "nodal" point in the wave pattern of the wave/particle as a function of space, thus creating a new wave pattern that disrupts the interference path. Indeed, a whole host of paradoxes would be eliminated by this Spatial Matrix concept.
Other concepts would be required to make full sense of this Matrix concept, but in the interest of keeping this simple and short, it is left as is here.
As we all know from experience, the detection point is determined wherever the probe/viewer is stationed. Therefore, in principle, the number of possible detection points are infinite in spacial locations, viz., an infinite number of the least time paths are possible. The orthodox particle theory of light would negate such a possibility, but the wave nature of light would seem to allow it. Of course, such detections by eye are a result of many emissions of photons.
But a question arises that, in theory, if we could set up an infinite number of different probes would we detect the same "one" photon at all the infinite probe locations "simultaneously" (ignoring the spatial distance of time delay of the different locations), or is this detection dependent on the finite quantum packet of the photon "particle" and therefore detectable by only one probe? Presumably then, the shortest least action path would be that detection point. Although, this could also possibly be a wave function of a finite quantum packet of the photon.
Has a similar experiment ever been conducted?
However, it is odd that the experiment is conducted under the assumption that reflection occurs as a wave function phenomenon, given that a mirror surface is a reflector of light waves, which can therefore be predictably determined by the least time path, and disregarding a particle probability consideration. The least action path, relative to reflection paths, seems contradictory to the particle paradigm. It also seems to unnecessarily complicate the understanding of Nature by probability functions. Feynman himself concedes this Strange conundrum in the very title of his book. A more sensible paradigm would be that a Spacial matrix is the actual function of "motion", energy, and matter themselves, albeit, one that cannot presently be defined by orthodox science. It is true that the equations in the QED and Quantum Mechanics models are highly precise in their predictions, but this would be expected, given that the elements of their equations are based on known experimental constants and extrapolated therefrom; viz. that such predictions are simply mathematical logical extensions. The same can be said of particle theory creation via collider experiments, thereby creating mathematical "phantom" particles that normally have no reality in Nature, except as logical extensions of synthetic conditions. Similarly, in Quantum Mechanics, the Spacial Matrix concept would eliminate the paradox of an interference pattern creation in the two slit experiment created by a series of single shot electrons. And the use of probes to detect which slit the electron emerges from is nonsensical, since it introduces a type of "nodal" point in the wave pattern of the wave/particle as a function of space, thus creating a new wave pattern that disrupts the interference path. Indeed, a whole host of paradoxes would be eliminated by this Spatial Matrix concept.
Other concepts would be required to make full sense of this Matrix concept, but in the interest of keeping this simple and short, it is left as is here.
