Quadratic functions: [Diagram Included] Football game scenario

[Swan]

Homework Statement

You are the quarterback for the Quinte Saints Football team. You are in the middle of the COSSA gold medal game and you see your receiver is wide open down the field beside the sideline. If he catches the ball, you win the game. However, the biggest guy Joey from the opposing team who is 190 cm tall is running towards you. You decide to throw the ball so that the highest part of the path is 1.5 m over Joey's head to avoid him reaching it if he jumps. You throw the ball at your receiver releasing the football at your head level when Joey is 5 m away from you.

The goal of this task is to figure out if your teammate can catch the ball and win the game.

Assumption variable: Your Height (to the nearest centimeter): 150 cm. **NOTE**: We had to assume what the quarterback's height was and in this my height is 150 cm.

a) Draw a sketch of the situation including the path of the ball (assume no wind). Fill in all information you know at this time.​

b) Determine the equation representing the path of the football.​

c) Your receivers typically catch the ball 1 m from the ground. If your player is 9.5 m away, and can run within 2 m of his initial location to catch the ball, does he catch the ball to win the game? Justify.​

Homework Equations

None

The Attempt at a Solution

a)

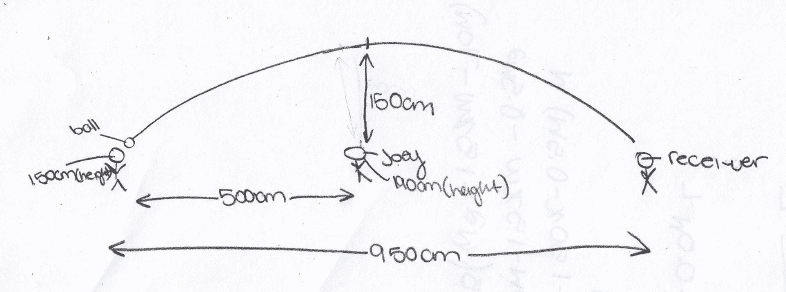
b) I have no idea how to go about this.​

c) I say he would catch the ball to win the game because the receiver can cover 2 m of horizontal distance if needed.​

 

[tia89]

Ok for a) (even if you could be a little clearer and for example show the difference in height of Joey, you and the fact that the receiver capture the ball at 1m height).

As for b), decompose the motion in an horizontal one (x(t), given by a usual uniform rectilinear motion) and a vertical one (y(t), uniformly accelerated motion in the gravity field). Then solve y(x) and you have the equation for the path.

Finally for c) using the path just found, check at what distance the ball fall from the receiver and you're done