Quadratic with simple meaningful intuitive constants

[PaulDiddams]

TL;DR Summary

A simple quadratic rearrangement that uses the intercept and the values of x and y that define the maxima or minima, in place of a, b and c, to drive a quadratic function. (demo Excel sheet attached).

Ever made a simple model that fits a quadratic function?

Tweaking the a, b and c constants to fit new observed data is a bit of a pain.

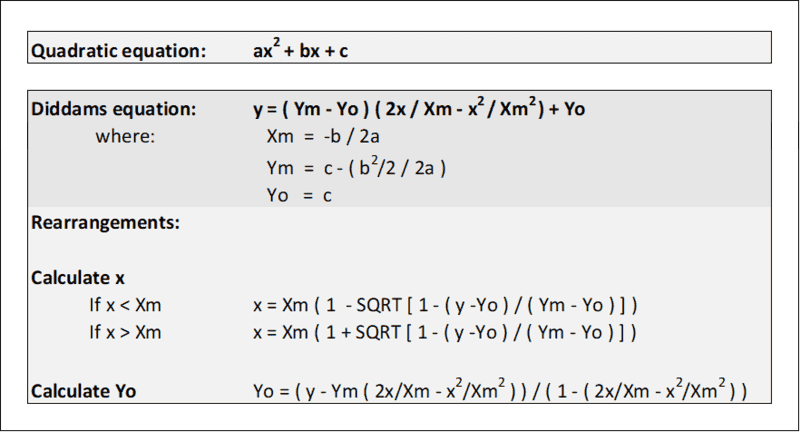
When I was a grad. student I came up with the following simple quadratic rearrangement that uses the intercept (Yo) and the values of x and y that define the maxima or minima (Xm, Ym) in place of non-intuitive a, b and c constants to drive the quadratic function. I also include rearrangements to calculate x from y, or Yo which I often find very useful too.

I would appreciate being credited "Diddams equation" if you choose to use my rearrangement. I think it's really neat and very powerful.

Enjoy.

 

[mfb]

That's a simple rearrangement exercise for high school, done millions of times before.
Naming things after yourself is frowned upon by the way. Even if it were something new.

 

[PaulDiddams]

That's a simple rearrangement exercise for high school, done millions of times before.
Naming things after yourself is frowned upon by the way. Even if it were something new.

I've been using this rearrangement for over 30 years now and neither I nor anyone I know has ever seen it done before, and my colleagues have been referring to it by that name for point of reference. If you have references where it's been done and shared before I'm interested to see them.