Quantum Entanglement information transmission idea to knock down....

[LozD]

TL;DR Summary

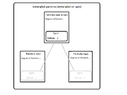
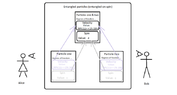
Many entangled particle pairs are prepared with property A (1 or 0) at 99% probability of 1, and property B (1 or 0) at 1% probability of 1. The pairs are separated with 2 observers, X and Y, each having a particle of each pair. They pre-arrange that at a certain time: Y will "send a 0" by NOT measuring anything on his particles or Y will "send a 1" by measuring property B on every one of his particles. X will measure property A on her particles: If 99% = 1, Y sent a 1; if 50%, Y sent a 0.

I work in IT and am a layman in the quantum world. I have obviously misunderstood something in my amateur reading of quantum, but if someone could explain my mistake in the above scenario it might be very insightful for me! Forgive me if the terminology is not correct - or if indeed lay folks' enquiries on this forum are inappropriate then please ignore me!

Here's the above again with a bit more detail and the concepts that lead me to assume the main steps ...

1. I take a pair of particles with two properties (spin, velocity, whatever) A and B with values 1 or 0 and prepare them (entangle them): 99% A is 1, 1% B is 1.
2. Send one of the particles with another observer to another place.
3. Tell them that at a pre-arranged time they must either (a) not observe their particle if they wish to send a 0, or, (b) observe (measure) the B property if they wish to send a 1.
4. At the pre-arranged time (or just after!) I then observe my particle's A value.
5. If the sender *didn't* observe her particle then the A property of my particle will be 1 with the original prepared 99% probability, because I am the first observer of any property of the entangled pair. (Btw, thereafter, property B, if examined by me or the other observer, would only have a 50% chance, not 1%, of being 1, due to "complementarity" and the Heisenberg Uncertainty Principle, but this is not directly relevant here). So, per the pre-arranged plan, the remote observer has "sent a 0" if the probability of property A being 1 is 99%.
6. If the sender *did* observe her particle's B property then the A property of my particle will be 1 with only a 50% probability, because the sender has observed property B and due to "complementarity" and the Heisenberg's Uncertainty Principle the probability of property A being 1 has collapsed to only 50%.
7. By using multiple pairs of such entangled particles the probability difference of 99% vs 50% for 0 or 1 respectively will allow me to derive a 0 was sent if the number of 1's on A of my particles is near 99%, or a 1 was sent if the number of 1's is near 50%, with good accuracy.
   

This appears not to require any comparison value of A to be sent from the remote observer back to me after the above events which would necessarily happen at equal to or less than the speed of light via another communication method. Rather, the information arrives instantaneously - so long as the synchronous nature of the pre-arrangement protocol is reliable.

My reasoning around the "complementarity" and Heisenberg Uncertainty Principle pieces came from the narrative in the section entitled "Bohm's variant" in this link ...

https://en.m.wikipedia.org/wiki/EPR_paradox

Whatever axis their spins are measured along, they are always found to be opposite. In quantum mechanics, the x-spin and z-spin are "incompatible observables", meaning the Heisenberg uncertainty principle applies to alternating measurements of them: a quantum state cannot possesses a definite value for both of these variables. Suppose Alice measures the z-spin and obtains +z, so that the quantum state collapses into state I. Now, instead of measuring the z-spin as well, Bob measures the x-spin. According to quantum mechanics, when the system is in state I, Bob's x-spin measurement will have a 50% probability of producing +x and a 50% probability of -x. It is impossible to predict which outcome will appear until Bob actually performs the measurement. Therefore, Bob's positron will have a definite spin when measured along the same axis as Alice's electron, but when measured in the perpendicular axis its spin will be uniformly random. It seems as if information has propagated (faster than light) from Alice's apparatus to make Bob's positron assume a definite spin in the appropriate axis.

... and from the narrative in this excellent layman's explanation of Quantum Entanglement ...

https://www.quantamagazine.org/entanglement-made-simple-20160428/

Upon deeper reflection, the paradox dissolves further. Indeed, let us consider again the state of the second system, given that the first has been measured to be red. If we choose to measure the second q-on’s color, we will surely get red. But as we discussed earlier, when introducing complementarity, if we choose to measure a q-on’s shape, when it is in the “red” state, we will have equal probability to find a square or a circle. Thus, far from introducing a paradox, the EPR outcome is logically forced. It is, in essence, simply a repackaging of complementarity.

If anyone who knows what they're talking about has got a spare moment to put me straight, please fire away!

 

[LozD]

Just in case it's not clear what my issue is: as far as I have read, using Quantum Entanglement to communicate information, i.e. like data, e.g. 1's and 0's, is not possible with QE without the transmission of a comparison copy of the information making its way from sender to recipient via another non-QE route in parallel, that necessarily, due to Special Relativity, transmits at equal to or less than the speed of light. My above thought experiment scenario purports to contradict that limitation and does allow information transmission over the purely QE system instantaneously with no parallel, sub- speed-of-light, back-channel required.

 

[PeroK]

Summary:: Many entangled particle pairs are prepared with property A (1 or 0) at 99% probability of 1, and property B (1 or 0) at 1% probability of 1. The pairs are separated with 2 observers, X and Y, each having a particle of each pair. They pre-arrange that at a certain time: Y will "send a 0" by NOT measuring anything on his particles or Y will "send a 1" by measuring property B on every one of his particles. X will measure property A on her particles: If 99% = 1, Y sent a 1; if 50%, Y sent a 0.

The distribution of the results of X's measurements is independent of what Y does. There's no way for X to know whether Y undertook any measurements or not.

So, yes, you've fundamentally misundersood quantum entanglement.

 

[LozD]

OK, I thought entanglement meant the two particles share the same state? So if A measures the state of his particle whatever it is will be the state of B's particle, even though they won't know they've measured it without transmitting that by other means. So A could say to himself on measuring the state of his particle, "Aha, it's a 1. I've no idea if B has read it yet, but if she does, it will be a 1 also". However, as soon as that "first read" is done, all bets are off in terms of the other prepared states (velocity, spin, etc.) on the particle. They collapse to being 50% (if the possible values are binary for example) and are no longer the original probabilities when the particles were prepared and both observers informed of those probabilities.

 

[PeroK]

OK, I thought entanglement meant the two particles share the same state? So if A measures the state of his particle whatever it is will be the state of B's particle, even though they won't know they've measured it without transmitting that by other means. So A could say to himself on measuring the state of his particle, "Aha, it's a 1. I've no idea if B has read it yet, but if she does, it will be a 1 also".

Yes, but that's a not a message from A to B (or from B to A) as A and B have no control over the results of their own or the other's measurements.

 

[LozD]

Quite so, it's not a message. It's not on that B "channel" that the message is really being sent. The B state is merely there for Y to "measure or not at midnight" (or whatever time) and make the first "read" of this connected system. It doesn't matter what value is discovered on B by Y, it's irrelevant. And X will never even look at B either, doesn't need to. Instead X will look at A at 1 minute past midnight, which Y never touched at her end. If X discovers A is 99% chance 1, then X will know that Y didn't measure anything at midnight, specifically, Y didn't measure B. If X discovers A is 50% chance 1, then X will know that Y did measure B at midnight. A 1 or 0 has passed from X to Y through their pre-arrangement of midnight and 1 minute past midnight synchronous protocol.

 

[Nugatory]

OK, I thought entanglement meant the two particles share the same state?

Yes, they do have the same state. However, if we prepare the particles in the state such that A has a 99% chance of getting a 1 and a 1% chance of getting a zero and (for the sake of argument the entanglement is such that) B’s measurement on the same axis will have the same probabilities, then any measurement on any axis performed by B will be consistent with that state - no matter what A has done or will do.

As an aside, although the mathematical formalism allows me to write down and reason about that state, I know of no process that could produce it in the real world. Real experiments are done with the physically realizable state (the “singlet state” when we’re working with spin) in which A and B both have a 50% chance of getting either result.

 

[PeroK]

Quite so, it's not a message. It's not on that B "channel" that the message is really being sent. The B state is merely there for Y to "measure or not at midnight" (or whatever time) and make the first "read" of this connected system. It doesn't matter what value is discovered on B by Y, it's irrelevant. And X will never even look at B either, doesn't need to. Instead X will look at A at 1 minute past midnight, which Y never touched at her end. If X discovers A is 99% chance 1, then X will know that Y didn't measure anything at midnight, specifically, Y didn't measure B.

I've already said that is a fundamental misunderstanding. X will get A 99% of the time regardless of what Y does or does not do.

 

[DrChinese]

1. I take a pair of particles with two properties (spin, velocity, whatever) A and B with values 1 or 0 and prepare them (entangle them): 99% A is 1, 1% B is 1.

This is not an entangled state. In an entangled state, the particles' entangled properties do not have a specific value until they are measured. So you would actually have something more like:

Before measurement:
50% (A=1 + B=0) + 50% (A=0 + B=1)

After measurement:
100% (A=1, B=0) [or vice versa 100% (A=0, B=1)]

Note that this assumes you are measuring the 2 particles in the same manner. The order of measurement is not important to the statistical results.

 

[LozD]

According to the following paragraphs from the links I gave, they appear to contradict you - see my bold ...

Wiki link: -

Whatever axis their spins are measured along, they are always found to be opposite. In quantum mechanics, the x-spin and z-spin are "incompatible observables", meaning the Heisenberg uncertainty principle applies to alternating measurements of them: a quantum state cannot possesses a definite value for both of these variables. Suppose Alice measures the z-spin and obtains +z, so that the quantum state collapses into state I. Now, instead of measuring the z-spin as well, Bob measures the x-spin. According to quantum mechanics, when the system is in state I, Bob's x-spin measurement will have a 50% probability of producing +x and a 50% probability of -x. It is impossible to predict which outcome will appear until Bob actually performs the measurement. Therefore, Bob's positron will have a definite spin when measured along the same axis as Alice's electron, but when measured in the perpendicular axis its spin will be uniformly random. It seems as if information has propagated (faster than light) from Alice's apparatus to make Bob's positron assume a definite spin in the appropriate axis.

Quanta Magazine link: -

Upon deeper reflection, the paradox dissolves further. Indeed, let us consider again the state of the second system, given that the first has been measured to be red. If we choose to measure the second q-on’s color, we will surely get red. But as we discussed earlier, when introducing complementarity, if we choose to measure a q-on’s shape, when it is in the “red” state, we will have equal probability to find a square or a circle.

 

[PeterDonis]

According to the following paragraphs from the links I gave

Neither of which are valid references. You need to be looking at textbooks and peer-reviewed papers, not Wikipedia and pop science magazines.

 

[DrChinese]

Suppose Alice measures the z-spin and obtains +z, so that the quantum state collapses into state I. Now, instead of measuring the z-spin as well, Bob measures the x-spin. According to quantum mechanics, when the system is in state I, Bob's x-spin measurement will have a 50% probability of producing +x and a 50% probability of -x.

Of course, this is correct as written. If Alice measures the z spin and gets +z (a 50-50 likelihood), Bob can measure on the x, y or z planes and his will result will ALSO be completely random (regardless of which plane he chooses). If he chooses z to measure, his result will be random but correlated (as expected) with Alice's result.

 

[PeroK]

It seems as if information has propagated (faster than light) from Alice's apparatus to make Bob's positron assume a definite spin in the appropriate axis.

That's nonsense. Although, I wonder whether the article you are quoting goes on to say as much, and is playing devil's advocate at this point.

Quanta Magazine link: -

Upon deeper reflection, the paradox dissolves further. Indeed, let us consider again the state of the second system, given that the first has been measured to be red. If we choose to measure the second q-on’s color, we will surely get red. But as we discussed earlier, when introducing complementarity, if we choose to measure a q-on’s shape, when it is in the “red” state, we will have equal probability to find a square or a circle.

Again, q will get square or circle with 50% probability regardless of what p does or does not do.

 

[LozD]

This is not an entangled state. In an entangled state, the particles' entangled properties do not have a specific value until they are measured. So you would actually have something more like:

Before measurement:
50% (A=1 + B=0) + 50% (A=0 + B=1)

After measurement:
100% (A=1, B=0) [or vice versa 100% (A=0, B=1)]

Note that this assumes you are measuring the 2 particles in the same manner. The order of measurement is not important to the statistical results.

Aha, now you're talkin'!

OK, my supposition that the probabilities could be set across two properties of the state in preparing the entanglement might be an issue. I think this more technical / mathematical discussion might be the same thing. From skim reading it, it appears the "correct" answerer is saying the preparing can set the probabilities but you lose something else in the maths or something that spoils it. Let's read it a bit more slowly ...

https://physics.stackexchange.com/q...pare-and-reset-probabilities-to-send-informat

 

[Irishdoug]

Many entangled particle pairs are prepared with property A (1 or 0) at 99% probability of 1, and property B (1 or 0) at 1% probability of 1.

I don't believe such a state would really be considered entangled.

 

[Nugatory]

Many entangled particle pairs are prepared with property A (1 or 0) at 99% probability of 1, and property B (1 or 0) at 1% probability of 1.

I don't believe such a state would really be considered entangled.

The state $\sqrt{.99}|11\rangle+\sqrt{.01}|00\rangle$ would, I think, generally be considered to be entangled. (I also have no idea how to prepare it).

 

[LozD]

To be honest, the B initial B state doesn't matter. That was an unnecessary constraint I applied. B can just be 50% with no preparing. All that's needed is for A to be able to be shaped to something that isn't 50%. Does that make for an entanglement now then, with only A "nudged"?

 

[Nugatory]

To be honest, the B initial B state doesn't matter.

On the contrary, what you are calling “the B state” is essential to calculating the results of B’s measurement. It is also an essential part of the overall system state because, as you said in #4 above, the two particles share the same state: there is a single quantum state of the entire two-particle system, it includes all the information needed to calculate the result of measurements by A and B, and because it cannot be separated into an “A state” and a “B state” (formally this property is called “non-factorizable” and it what makes the state entangled) it makes no sense to talk about either particle’s state in isolation.

 

[LozD]

On the contrary, what you are calling “the B state” is essential to calculating the results of B’s measurement. It is also an essential part of the overall system state because, as you said in #4 above, the two particles share the same state: there is a single quantum state of the entire two-particle system, it includes all the information needed to calculate the result of measurements by A and B, and because it cannot be separated into an “A state” and a “B state” (formally this property is called “non-factorizable” and it what makes the state entangled) it makes no sense to talk about either particle’s state in isolation.

But we don't care about the results of B's measurement. We only care that its has been measured or not.

Whenever I refer to measuring at one end or the other I am assuming that that action is measuring the shared state. There is no local state if they are entangled, I get that, I misled you if I made you think I was referring to either particle in isolation.

I think my misunderstanding might be to do around state and what I'm calling "properties". The state is the "wholeness" of the shared characteristics of the pair; the state has sub-components like axis, velocity, location, etc. All those properties make up the state. Or am I wrong there?

Hence why the Quanta Magazine article refers to the two systems (the two particles in my parlance) each having shape and colour as two properties that make up the state. And the Wiki refers to x and y-axis spin making up the shared state of the pair in their example.

 

[PeroK]

I think my misunderstanding might be to do around state and what I'm calling "properties". The state is the "wholeness" of the shared characteristics of the pair; the state has sub-components like axis, velocity, location, etc. All those properties make up the state. Or am I wrong there?

Look at it this way: I have a particle and I am about to measure some quantity associated with that particle. Let's say spin about the x-axis to be precise. And, let's say, that the probabililty is 50-50 that it is spin-up or spin-down.

Now, you tell me that something about another particle has been measured. That cannot change the 50-50. You might say: someone measured the x-spin of another particle. Okay, but it's still 50-50 on my particle.

Then, however, you tell me the result of that measurement. You say: the other particle was measured and it was spin-up. That changes the probability. Now I know that my particle is x spin-up.

Your mistake is to think that the probabilities change by knowing that another measurement has been made, without knowing the outcome.

That's fundamentally wrong, whatever you think you've read in Quanta magazine.

 

[LozD]

Look at it this way: I have a particle and I am about to measure some quantity associated with that particle. Let's say spin about the x-axis to be precise. And, let's say, that the probabililty is 50-50 that it is spin-up or spin-down.

Now, you tell me that something about another particle has been measured. That cannot change the 50-50. You might say: someone measured the x-spin of another particle. Okay, but it's still 50-50 on my particle.

Then, however, you tell me the result of that measurement. You say: the other particle was measured and it was spin-up. That changes the probability. Now I know that my particle is x spin-up.

Your mistake is to think that the probabilities change by knowing that another measurement has been made, without knowing the outcome.

That's fundamentally wrong, whatever you think you've read in Quanta magazine.

Your mistake is to think that the probabilities change by knowing that another measurement has been made, without knowing the outcome.

I'm not saying the probabilities change by knowing that another measurement has changed. I'm saying that the probability on, say, the x-axis spin change when the y-axis spin is measured, whether or not anyone knows about that measuring happening or not.

 

[Nugatory]

I think my misunderstanding might be to do around state and what I'm calling "properties".

There’s no way of responding without at least sniffing around the underlying math. If you’re not up for taking on an intro textbook you might give Giancarlo Girardi’s book “Sneaking a look at god’s cards” a try.

However I can try a very abbreviated corner-cutting explanation. In post #16 I wrote down an entangled state $|\psi\rangle$ that seems close to what you were trying to describe in your first post: $|\psi\rangle=\sqrt{.99}|11\rangle+\sqrt{.01}|00\rangle$

The things in $|\rangle$ brackets are called ”kets”; they are abstract mathematical objects that work like vectors - the sum of two kets is a ket, and a ket can be multiplied by a number to yield a “bigger” or “smaller” ket that “points in the same direction”.

The ket $|11\rangle$ corresponds to the state “If A measures the spin on a specific chosen in advance axis there is a 100% probability that they will get a 1 and if B measures the state on that same axis there is a 100% probability that they will get a 1”. The ket $|00\rangle$ is the same thing for 0 results.

The ket $|\psi\rangle$ is the state of the system and it is a sum of two kets, called a superposition. If we measure the spin of either particle on the specified axis the wave function will collapse to either $|00\rangle$ or $|11\rangle$; the probabilities are given by the square of the coefficients, in this case 99% and 1%.

So let’s say that A performs a measurement. 99% of the time the wave function collapses to $|11\rangle$ and 1% of the time to $|00\rangle$. Thus, after A has performed their measurement if B measures any property of their particle they will get results consistent with their particle being in the 1 state 99% of the time and consistent with their particle being in the 0 state 1% of the time. (And note that that is “any property” - if they measure something other than spin on the designated axis we’ll need to do some math to calculate the probabilities of those results from the 1 state and the 0 state, but we know how to do that).

But suppose A doesn’t perform any measurement so the wave function is uncollapsed when it gets to B? B does something to their particle, that collapses the wave function with the same 99% $|11\rangle$ and 1% $|00\rangle$ probabilities, and we are in the exact same situation as if A had made a measurement.

And that is why nothing A does can affect anything B observes, and therefore why A cannot send a signal to B by manipulating their side of the entanglement.

 

[LozD]

Aha, now you're talkin'!

OK, my supposition that the probabilities could be set across two properties of the state in preparing the entanglement might be an issue. I think this more technical / mathematical discussion might be the same thing. From skim reading it, it appears the "correct" answerer is saying the preparing can set the probabilities but you lose something else in the maths or something that spoils it. Let's read it a bit more slowly ...

https://physics.stackexchange.com/q...pare-and-reset-probabilities-to-send-informat

OK, the maths in that link I don't fully get, but the gist of the text description around it I have some inkling I think.

The answerer's answer to 1. acknowledges that you can "shape" (my terminology, sorry!) the probabilities on the particles and retain the entanglement. So 75/25% instead of 50/50% and they are entangled.

In questioner's 2 and 3 I can see now he is trying to "transmit" on just one property, like my property B, say x-axis spin or whatever. He's simply saying, if he shapes B to 75/25% and the remote observer measures enough of the particles and finds they're in the ration 75/25 then interpret a 1, else if he shapes B to 50/50% then the observer can interpret a 0. That simpler than my dual property approach and I never even thought of it.

The answerer demolishes it through the maths I don't understand, but I think basically says "No, the probability reverts to 50/50% no matter what you shape because the diagonal filter is a measurement and that collapses the entanglement.

But interestingly, in 4 the answerer talks about the internal entanglement between different properties of the state. So entanglement is possible between A and B (x-spin and y-spin) within the state, not just A (x-spin) to A (x-spin) and / or B (y-spin) to B (y-spin) between two particles that share a state.

Here's the paragraph ...

4. Each independent degree of freedom can sustain correlations with other degrees of freedom, on the same particle or other particles, in principle. In this case, all of the entanglement that the system had, aside from correlated uncertainties in their momenta (which you implicitly perform a weak measurement on, just by virtue of making a polarization measurement at a particular location after it was emitted), are in the polarizations. As the polarization of each beam is entirely involved in what entanglement there is (in a multi-particle scenario, you can consider entanglement spread across the system in a way that some particles are more involved in the entangled state than others), any single measurement will destroy all entanglement present.

"Any measurement will destroy all entanglement present". That's exactly what I am saying, is it not? That means if property A was shaped 99/1% (1/0) as I proposed, the measurement of B by the remote particle observer Y collapses the whole entanglement shooting match. So A becomes 50/50%. And that signifies a 1 was sent by Y (she measured B), whereas if A remains 99/1% that signifies a 1 was sent by Y (she didn't measure B).

 

[LozD]

When the entanglement collapses, I wonder what the speed of action is between the measured property that triggers the collapse and the other different properties with the same state (answerer's 4 above) to reset them - like if x-spin is measured how long does it take for y-spin to register that and go back to 50/50% if it was 99/1%? We know it's instantaneous for a property (say x-spin) between the two particles in the entangled state. But is it instantaneous between the measured property and the other properties in the state when collapse occurs?

Let's say my theory is true - the non-measured properties revert to 50/50% (1,0) from 99/1% (1,0) or whatever they were when entangled. Then the inter-property disentanglement transmission speed can't be instantaneous because then special relativity would be contradicted and causality would be transmittable faster than the speed of light. But if the inter-property disentanglement transmission speed was slower such that it exactly compensated for the instantaneousness of the inter-particle transmission then special relativity and the casualty faster than the speed of light problem would disappear.

So if the particles were separated by a distance such that the speed of light would take 1 minute to get between them, then even though the inter-particle collapse is instant the inter-property collapse would take 1 minute. So any event (the legendary cat with its poison trigger for example) hanging off the back of the switch back to 50/50% from 99/1% would still not be triggered before light would reach it from the remote particle and observer, because the delay has been shifted to the inter-property transmission.

That's testable, but not easy!

So while we wouldn't have a system that transmits information faster than the speed of light, we do have one that doesn't use light, electromagnetics, etc. mechanisms between its end points. So no-one could spy on it. Without access to one of the particles in the entanglement the communication would be secure (unless someone discovers what the transmission mechanism is for disentanglement). That'd be worth a bob or two if someone could get it working.

 

[StevieTNZ]

When the entanglement collapses, I wonder what the speed of action is between the measured property that triggers the collapse and the other different properties with the same state (answerer's 4 above) to reset them - like if x-spin is measured how long does it take for y-spin to register that and go back to 50/50% if it was 99/1%? We know it's instantaneous for a property (say x-spin) between the two particles in the entangled state. But is it instantaneous between the measured property and the other properties in the state when collapse occurs?

Let's say my theory is true - the non-measured properties revert to 50/50% (1,0) from 99/1% (1,0) or whatever they were when entangled.

I think you really need to read "Sneaking a Look at God's Cards" as suggested in post #22. Or https://mitpress.mit.edu/books/quantum-entanglement

 

[LozD]

I think you really need to read "Sneaking a Look at God's Cards" as suggested in post #22. Or https://mitpress.mit.edu/books/quantum-entanglement

For the shear brilliance of the name "Sneaking a Look at God's Cards" my order is on its way!

 

[Nugatory]

I'm saying that the probability on, say, the x-axis spin change when the y-axis spin is measured, whether or not anyone knows about that measuring happening or not.

They don’t, if we’re measuring x on one particle and y on the other.

The probability of getting spin-up on x given that a y measurement has been made on the other particle is the sum of the probability of getting spin-up on x if y was measured up times the probability that y would be measured spin up and the probability of spin-up on x if y was measured down times the probability of y would be measured spin-down - and that is equal to the probability of getting spin-up on x if no measurement has been made on y.

 

[DrChinese]

1. When the entanglement collapses, I wonder what the speed of action is between the measured property that triggers the collapse... That's testable, but not easy!

2. Without access to one of the particles in the entanglement the communication would be secure (unless someone discovers what the transmission mechanism is for disentanglement). That'd be worth a bob or two if someone could get it working.

The mystery here is why you are speculating about nuances of entanglement when you don't know the basics. Many of the questions you ask would be answered if you knew more about the subject from a scientific perspective (rather than reading highly abbreviated summaries). You are obviously interested and intelligent, so you should take time to follow some of the paths that have already been tread by others. Some basics are in the following paper, which is intended for college undergrads. It in turn references 3 key papers (and more of course): its references 4 (Einstein et al, 1935), 25 (Bell, 1964) and 30 (Aspect et al, 1982) - are considered essential to any sort of understanding of entanglement. And yes, it would take some of your time to learn about these and why they are important. But considering that there have been over 30,000 papers written on entanglement in just the past 20 years since the one below was written in 2002, reading these I mention will be a good jump start.

https://arxiv.org/abs/quant-ph/0205171
"We use polarization-entangled photon pairs to demonstrate quantum nonlocality in an experiment suitable for advanced undergraduates. The photons are produced by spontaneous parametric down conversion using a violet diode laser and two nonlinear crystals. The polarization state of the photons is tunable. Using an entangled state analogous to that described in the Einstein-Podolsky-Rosen “paradox,” we demonstrate strong polarization correlations of the entangled photons. Bell’s idea of a hidden variable theory is presented by way of an example and compared to the quantum prediction. A test of the Clauser, Horne, Shimony and Holt version of the Bell inequality finds S = 2.307±0.035, in clear contradiction of hidden variable theories. The experiments described can be performed in an afternoon."

----------------------------------------

Further, as you might imagine, many facets of entanglement have been studied quite deeply - including many around what you are speculating (although these studies relate to known theory and experiment).

1. Although not exactly the same as your 1 (per my labeling): the minimum experimentally determined speed at which collapse occurs between entangled particles is 10,000 times the speed of light (10^4 c, or 4 orders of magnitude). It is probably instantaneous, but we cannot be absolutely certain. :)

https://arxiv.org/abs/0808.3316

2. And secure communication is in fact one of the objectives in quantum cryptography:

https://en.wikipedia.org/wiki/Quantum_cryptography

Good luck!

 

[PeroK]

Your mistake is to think that the probabilities change by knowing that another measurement has been made, without knowing the outcome.

I'm not saying the probabilities change by knowing that another measurement has changed. I'm saying that the probability on, say, the x-axis spin change when the y-axis spin is measured, whether or not anyone knows about that measuring happening or not.

Okay, I think I understand your model of entanglement. We have entangled particles A and B:

Particle A is definitely x-spin-up (or 99% if you prefer); particle B is definitely/99% x-spin-down.

You measure z-spin of particle A. Now particle A is 50% x-spin-up; and, particle B is now 50% x-spin-up.

Therefore: if you measure particle B before particle A has been measured you almost certainly get x-spin-down; but, if you wait until particle A has been measured (in the z-direction), then the state of particle B changes from 99% x-spin-down to 50% spin-down.

This allows someone to send a message FTL by choosing to measure the z-spin of particle A or not.

I think that's what you're saying.

The only problem with this is that this is not QM; this is LozD mechanics (LM), which allows FTL signalling. There is very little, if anything, in common between QM and LM. They are two entirely different physical theories! And, critically, the experimental evidence supports QM and not LM.

 

[LozD]

Okay, I think I understand your model of entanglement. We have entangled particles A and B:

Particle A is definitely x-spin-up (or 99% if you prefer); particle B is definitely/99% x-spin-down.

You measure z-spin of particle A. Now particle A is 50% x-spin-up; and, particle B is now 50% x-spin-up.

Therefore: if you measure particle B before particle A has been measured you almost certainly get x-spin-down; but, if you wait until particle A has been measured (in the z-direction), then the state of particle B changes from 99% x-spin-down to 50% spin-down.

This allows someone to send a message FTL by choosing to measure the z-spin of particle A or not.

I think that's what you're saying.

The only problem with this is that this is not QM; this is LozD mechanics (LM), which allows FTL signalling. There is very little, if anything, in common between QM and LM. They are two entirely different physical theories! And, critically, the experimental evidence supports QM and not LM.

LM rocks!

Yes, that's it exactly! My poor usage of terminology confused everyone.

So my question is: why is LM not QM? What am I doing wrong in the LM? Now that I've cleared up my confused attempt to pose a thought experiment and been understood, what specifically is actually wrong in the LM trail of logic?

 

[PeroK]

LM rocks!

Yes, that's it exactly! My poor usage of terminology confused everyone.

So my question is: why is LM not QM? What am I doing wrong in the LM? Now that I've cleared up my confused attempt to pose a thought experiment and been understood, what specifically is actually wrong in the LM trail of logic?

Your thought experiment reveals several misconceptions about QM. First, I suggest, we need to look at quantum spin compared to classical spin.

For a classical object we can find an axis of rotation. E.g. the Earth has an axis of rotation, and so would a spinning basketball. And, the spin of either object is not of fixed magnitude: it may be increased or decreased if a torque is applied.

The spin on an electron is fundamentally different in a number of ways (which leads to the picture of a classically spinning object not being a good mental model):

The total amount of spin is fixed. The measured value is always $\dfrac {\sqrt 3 \hbar}{2}$. This cannot be increased or decreased. Moreover, the spin component when measured about any axis is always fixed in magnitude and only varies in direction. It's always $\dfrac{\hbar}{2}$.

This is completely non-classical and means we can never find an axis of rotation for the electron. Whatever axis we choose, the measured spin component is always effectively a third of the total spin.

These two characeristics lead to the electron's spin being described by a spin state. A study of the nature of electron spin and of electron spin states is a good place to start with QM.

Finally, if we measure the spin about a given axis, the state of the electron after the measurement correpsonds to a state where further measurements about the same axis will yield the same result. This is called an eigenstate.

For example, if we measure the spin about the z-axis and get $+\dfrac \hbar 2$, then the state of the electron after the measurement is z-spin-up.

If we prepare an ensemble of electrons in the z-spin-up state and measure spin about the x-axis or y-axis, then in each case we get a 50-50 split of x-spin-up and x-spin-down or y-spin-up and y-spin-down respectively. And, if we measure spin about an axis that makes an angle $\theta$ with the z-axis, then we get a measurement of spin-up with probability $\cos^2 theta$ (and spin-down with probability $\sin^2 \theta$).

Now, let's describe an experiment that does obey QM:

Two particles are created with zero total spin. This means that their spins must be opposite (if measured about any axis). This could be explained by each particle having a definite but opposite spin about each axis. This would be a so-called hidden variables theory. There's a long story here about how tests of Bell's inequality have ruled out such local hidden variable theories.

Instead, QM says that the spin of neither particle is determined until one particle is measured. This is called an entangled state and, indeed, the system may only be analysed as a two-particle system; not as two separate particles each with its own state.

If both particles are measured about the same axis, then they are always found to have opposite spins. QM is silent on how nature achieves this. QM simply says that is the what nature serves up when the particles are measured.

Now, we prepare an ensemble of such entangled pairs and carry out some spin measurements about different axes.

Let's say that one particle is always measured about the z-axis. We get a 50-50 split of z-spin-up and z-spin-down. If the other particle is measured about the x-axis, then we get a 50-50 split of x-spin-up and x-spin down. If the results are compared, we find no correlation between the results. I.e. 25% of the time we will get z-up, x-up; 25% of the time z-up, x-down; 25% z-down, x-up; and 25% z-down, x-down.

Note that measuring one particle about the z-axis has no effect on the 50-50 distribution of measurements about the x-axis.

Now, suppose we measure one particle about an axis an angle $\theta$ from the z-axis, where $\cos^2 \theta = 0.9$, say. Let's call this axis $w$.

As always, we will get 50% w-spin-up and 50% w-spin-down.

Finally, suppose we do the w-axis measurement after the first particle has been measured about the z-axis. We still get 50-50, but thse results are correleted with the random results that of the z-measurement. I.e.:

45% of the time we get z-up and w-down
5% of the time we get z-up and w-up
45% of the time we get z-down and w-up
5% of the time we get z-down and w-down

If the experimenter could force a result of z-up, then they could influence the distribution of w-axis measurements. But, they can't force the electron to be z-spin-up and thereby signal this to the second experimenter. The first measurement is random 50-50 of up or down. We have no control over this.

In conclusion, we have known correlation between measurement results; but no control over either set of measurement results, hence no signalling capability.

 

[LozD]

Your thought experiment reveals several misconceptions about QM. First, I suggest, we need to look at quantum spin compared to classical spin.

For a classical object we can find an axis of rotation. E.g. the Earth has an axis of rotation, and so would a spinning basketball. And, the spin of either object is not of fixed magnitude: it may be increased or decreased if a torque is applied.

The spin on an electron is fundamentally different in a number of ways (which leads to the picture of a classically spinning object not being a good mental model):

The total amount of spin is fixed. The measured value is always $\dfrac {\sqrt 3 \hbar}{2}$. This cannot be increased or decreased. Moreover, the spin component when measured about any axis is always fixed in magnitude and only varies in direction. It's always $\dfrac{\hbar}{2}$.

This is completely non-classical and means we can never find an axis of rotation for the electron. Whatever axis we choose, the measured spin component is always effectively a third of the total spin.

These two characeristics lead to the electron's spin being described by a spin state. A study of the nature of electron spin and of electron spin states is a good place to start with QM.

Finally, if we measure the spin about a given axis, the state of the electron after the measurement correpsonds to a state where further measurements about the same axis will yield the same result. This is called an eigenstate.

For example, if we measure the spin about the z-axis and get $+\dfrac \hbar 2$, then the state of the electron after the measurement is z-spin-up.

If we prepare an ensemble of electrons in the z-spin-up state and measure spin about the x-axis or y-axis, then in each case we get a 50-50 split of x-spin-up and x-spin-down or y-spin-up and y-spin-down respectively. And, if we measure spin about an axis that makes an angle $\theta$ with the z-axis, then we get a measurement of spin-up with probability $\cos^2 theta$ (and spin-down with probability $\sin^2 \theta$).

Now, let's describe an experiment that does obey QM:

Two particles are created with zero total spin. This means that their spins must be opposite (if measured about any axis). This could be explained by each particle having a definite but opposite spin about each axis. This would be a so-called hidden variables theory. There's a long story here about how tests of Bell's inequality have ruled out such local hidden variable theories.

Instead, QM says that the spin of neither particle is determined until one particle is measured. This is called an entangled state and, indeed, the system may only be analysed as a two-particle system; not as two separate particles each with its own state.

If both particles are measured about the same axis, then they are always found to have opposite spins. QM is silent on how nature achieves this. QM simply says that is the what nature serves up when the particles are measured.

Now, we prepare an ensemble of such entangled pairs and carry out some spin measurements about different axes.

Let's say that one particle is always measured about the z-axis. We get a 50-50 split of z-spin-up and z-spin-down. If the other particle is measured about the x-axis, then we get a 50-50 split of x-spin-up and x-spin down. If the results are compared, we find no correlation between the results. I.e. 25% of the time we will get z-up, x-up; 25% of the time z-up, x-down; 25% z-down, x-up; and 25% z-down, x-down.

Note that measuring one particle about the z-axis has no effect on the 50-50 distribution of measurements about the x-axis.

Now, suppose we measure one particle about an axis an angle $\theta$ from the z-axis, where $\cos^2 \theta = 0.9$, say. Let's call this axis $w$.

As always, we will get 50% w-spin-up and 50% w-spin-down.

Finally, suppose we do the w-axis measurement after the first particle has been measured about the z-axis. We still get 50-50, but thse results are correleted with the random results that of the z-measurement. I.e.:

45% of the time we get z-up and w-down
5% of the time we get z-up and w-up
45% of the time we get z-down and w-up
5% of the time we get z-down and w-down

If the experimenter could force a result of z-up, then they could influence the distribution of w-axis measurements. But, they can't force the electron to be z-spin-up and thereby signal this to the second experimenter. The first measurement is random 50-50 of up or down. We have no control over this.

In conclusion, we have known correlation between measurement results; but no control over either set of measurement results, hence no signalling capability.

That's a very cool "deeper dive", so-to-speak, explanation of the quantum phenomenon as described in a the shallower manner in the Quanta Magazine article - thank you!

It also helps me frame my question yet again, hopefully slightly more accurately than even the last attempt. This is rather like a heat seeking missile that shoots off to the left of target, corrects, but overshoots to the right of target (but not quite as far as it did to the left), corrects, and so on. We might hit the target eventually or I might not be brainy enough in which case we won't!

So spin - and its x, y, z ... axes and attendant rules regarding amount of spin, magnitude of spin and such - is one, correct me if I'm wrong, "degree of freedom" of the particle, in this case an electron. A "degree of freedom" is what I was previously calling a property of the particle. Degrees of freedom, like spin, location, velocity, are the top level characteristics of the particle. Each comes with its own payload of rules and constraints, like the ones you described for spin.

I think I get entanglement between a degree of freedom, like spin, between two particles now.

So here's two independent (unentangled particles). The spin value on particle One is a, and the spin on particle Two is b. There is no correlation or "sharedness" of the two results. They are both local, independent. Knowing the value of one shines no light on the value of the other.

In software development terms, these are two "variables by value", each has its own storage where the value is contained. (In contrast to two "variables by reference", see next) ...

Now, here's two entangled particles, entangled by their spin degree of freedom ...

The spin value for particles One and Two is maintained in one shared place - in the top box in the diagram entitled "Particle one & two" - and the value is c. Since the value of c is shared between both entangled particles I have greyed out the local c value of spin in each particle as a visual cue that the value is not really there but rather in the shared location above.

In software development terms the spin in each particle is a "variable by reference". That means the spin variable in each particle doesn't hold the value of the spin in storage but rather a pointer to the shared memory location where the value resides, in this case in the Spin value storage location in the "Particle one & two" box. Hence also the arrow heads on the grey lines pointing from the local particles to the shared box: quite literally software pointers in the programming analogy.

So by the great big gaps in each of those boxes representing the particles you can maybe see where I am going with this, but didn't explain myself very well previously.

I'm going to post this and then come back ...

 

[PeroK]

I'm not sure how far the programming analogy can be pushed, but to implement quantum entanglement in a program:

You would wait until a "measure spin about axis $\hat n$" command was received. Randomly select $\pm \dfrac \hbar 2$ for the measured particle, return this value; set the state of that particle to $\hat n$ up; set the state of the second particle to $\hat n$ down; and, break the entanglement.

Where $\hat n$ is some axis in 3D space.

There are complications brought about by SR and the relatively of which measurement was first, but that's the gist of it.

 

[LozD]

That's a very cool "deeper dive", so-to-speak, explanation of the quantum phenomenon as described in a the shallower manner in the Quanta Magazine article - thank you!

It also helps me frame my question yet again, hopefully slightly more accurately than even the last attempt. This is rather like a heat seeking missile that shoots off to the left of target, corrects, but overshoots to the right of target (but not quite as far as it did to the left), corrects, and so on. We might hit the target eventually or I might not be brainy enough in which case we won't!

So spin - and its x, y, z ... axes and attendant rules regarding amount of spin, magnitude of spin and such - is one, correct me if I'm wrong, "degree of freedom" of the particle, in this case an electron. A "degree of freedom" is what I was previously calling a property of the particle. Degrees of freedom, like spin, location, velocity, are the top level characteristics of the particle. Each comes with its own payload of rules and constraints, like the ones you described for spin.

I think I get entanglement between a degree of freedom, like spin, between two particles now.

So here's two independent (unentangled particles). The spin value on particle One is a, and the spin on particle Two is b. There is no correlation or "sharedness" of the two results. They are both local, independent. Knowing the value of one shines no light on the value of the other.

In software development terms, these are two "variables by value", each has its own storage where the value is contained. (In contrast to two "variables by reference", see next) ...

View attachment 295631

Now, here's two entangled particles, entangled by their spin degree of freedom ...

View attachment 295633
The spin value for particles One and Two is maintained in one shared place - in the top box in the diagram entitled "Particle one & two" - and the value is c. Since the value of c is shared between both entangled particles I have greyed out the local c value of spin in each particle as a visual cue that the value is not really there but rather in the shared location above.

In software development terms the spin in each particle is a "variable by reference". That means the spin variable in each particle doesn't hold the value of the spin in storage but rather a pointer to the shared memory location where the value resides, in this case in the Spin value storage location in the "Particle one & two" box. Hence also the arrow heads on the grey lines pointing from the local particles to the shared box: quite literally software pointers in the programming analogy.

So by the great big gaps in each of those boxes representing the particles you can maybe see where I am going with this, but didn't explain myself very well previously.

I'm going to post this and then come back ...

Now, here I'm introducing another degree of freedom (my aka "property") of the particle: velocity. And that's entangled between the two particles too ...

Again I've shown the local value boxes greyed out to signify the value is not really there but rather in the shared place above, attendant arrows pointing to the shared place too. (Note I've put a slight purple tinge on this greyed-ness for Velocity to distinguish it from Spin, which I'll use later).

So, we now have two entangled particles, One and Two, with their Spin and Velocity degrees of freedom entangled.

Next, let's say the Velocity can be some value between 0 and 1. When I prepare the particles, I set the probability like this: |v> = 99% |11> + 1% |00> . (This is LozD using ket-speak for the first time so beware more confusion by me, feel free to critique me, and hat-tip @ Nugatory!) In plain English I'm saying "I'm preparing the velocity degree of freedom of the entangled particles to 99% probability it is 1, 1% it is 0.

We introduce Alice and Bob and give them each a particle; Alice has One and Bob has Two, and we separate them. Here they are with the prepared pair of particles ...

We want Bob to communicate a 1 or 0 in the following manner ...

At midnight, if Bob wants to send a 0 he must not measure anything on his particle. Not Spin, not Velocity, nada. On the other hand, if he wants to send a 1 then he will examine the Spin. This protocol is pre-arranged with Alice and Bob.

At one minute past midnight, Alice will measure Velocity. If Bob didn't measure anything at midnight, then Alice's measuring of Velocity will have the probability |v> = 99% |11> + 1% |00> . She can interpret this as a zero being been sent by Bob. On the other hand, if Bob did measure Spin he will have collapsed the entanglement, then Alice's measuring of Velocity will have the probability |v> = 50% |11> + 50% |00> . She can interpret this as a one being been sent by Bob. Hence we have information communication between the two observers at a distance, and this will be happening instantaneously (or however many thousands of times faster than the speed of light someone posted that disentanglement happens at). And no subluminal back channel is required to send the information for comparison.

Now it might be said (someone did on this thread) that the entanglement collapse will only happen for the Spin, not the Velocity. So the spin will remain |v> = 99% |11> + 1% |00> as it was originally prepared and not revert to |v> = 50% |11> + 50% |00> .

Well, this is where the "degree-of-freedom to degree-of-freedom" entanglement comes in. If in addition to the particles being entangled, the Spin and Velocity are also entangled, then Bob's entanglement collapse of Spin will cascade to Velocity. See this diagram ...

The entanglement is shown by greying with a butterscotch tinge the two degrees of freedom, Spin and Velocity. (Perhaps I should have shown the entanglement in the shared place in black to be consistent with the notion that this is where the real values are and not on the particles?). But the entanglement collapse path if Bob transmits a 1 is: Bob measures Particle Two's Spin, that collapses the entanglement in the notional shared values Particle One & Two (right hand side butterscotch arrow), which in turn collapses the entanglement between Spin and Velocity in the shared notional particle (curved lines on either side of the shared notional particle One & Two), which in turn collapses the Spin and Velocity in the Particle One (left hand side butterscotch arrow), which means that when Alice measures Particle One's Velocity at a minute past midnight it will be |v> = 50% |11> + 50% |00> - Bob sent a 1. And if Bob doesn't measure Spin on Particle Two at midnight none of that cascading collapse will happen, and Alice's Velocity probability will be |v> = 99% |11> + 1% |00> - Bob sent a 0.

Is that clear now?

 

[PeroK]

That feels more like Alice in Wonderland, following Bob down a rabbit hole!