Qubits state calculation

[Hennessy]

TL;DR Summary

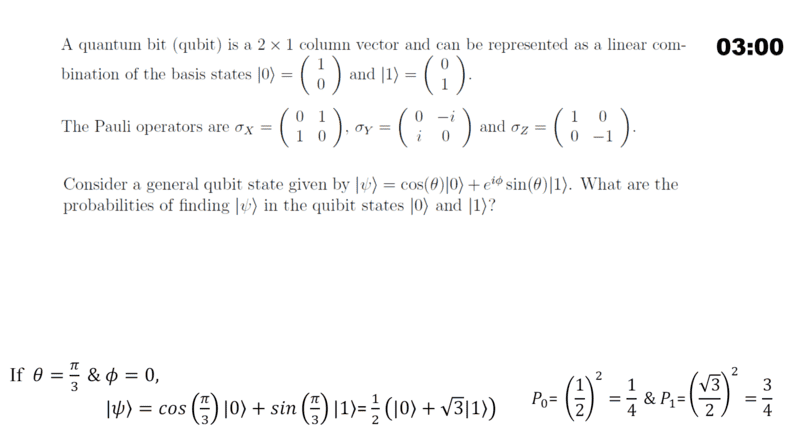
A question regarding calculating a Qubits state

Hello All, 2nd year undergrad taking my first course in modern physics. We have been given this question in a mock exam and at the bottom is the solution. When looking at a general cubit it seems the argument of both sin and cos functions should be (pi/2) not (pi/3). I have figured out a different question by obtaining the complex conjugate of this wavefunction however we were not given an argument we were just given variables. So could someone please explain the solution for this , i don't see how any matrix multiplication brings out a factor of a half at any point. I can see for P_1 that Sin(pi/3) = sqrt3/2 but that's about it. I also understand the a linear combination of a given wavefunctions' probability amplitudes should add to 1. Any advice would be appreciated, thanks guys <3 p.s why has he chosen theta = pi/3 and pfi = 0 ?

 

[Hill]

TL;DR Summary: A question regarding calculating a Qubits state

i don't see how ... brings out a factor of a half at any point. I can see for P_1 that Sin(pi/3) = sqrt3/2 ...

$\cos(\frac {\pi} 3)=\frac 1 2$. Does it help?

 

[Hennessy]

Hi Hill,

Okay this makes more sense now and i see that we can pull out the 1/2 for sqr3/2 aswell so thank you for clarifying this and helping me be able to answer it appreciate it :).

 

[DrClaude]

p.s why has he chosen theta = pi/3 and pfi = 0 ?

No idea. Were I asking such a question, I would want an answer in terms of θ and φ.

 

[Hennessy]

No idea. Were I asking such a question, I would want an answer in terms of θ and φ.

yeah unfortunately my lecturer didn't give any reasoning , we asked why and he just said it was a "standard result" so i understood that as just " just take it as a fact" :/, not the best for learning but i've learnt to take some things on the chin on my degree when i don't understand them deeply enough for me to connect the dots.

 

[cianfa72]

Yes, the general qubit state $\ket{\psi}$ in the $\{\ket{\uparrow}, \ket{\downarrow} \}$ basis is $$\ket {\psi}=\cos(\theta/2) \ket{\uparrow}+e^{i\varphi}\sin(\theta/2) \ket{\downarrow}$$ it is a unit vector (i.e. with norm 1) in the complex Hilbert space of dimension 2.

You can also think of it as a point in the projective line space built over the above Hilbert space.

 

[Nugatory]

p.s why has he chosen theta = pi/3 and pfi = 0 ?

Unless there are more parts to the problem (the otherwise irrelevant Pauli matrices suggest that there might be) it looks as if these numbers were chosen arbitrarily to make the problem definite and the trig functions easily calculated.

Sort like when the elementary school teacher says "Jill has five apples and Jane has three apples. How many apples total?".... Why "five" and "three"? No reason, the teacher needed two numbers to pose an addition problem and those are what they chose.