- #1
ajovcu
- 4
- 0
Hello all, I'm a new member. I've decided banging my forehead until its red will not make me any more able to solve my practice problems. I hope I haven't posted this in the wrong forum but here is the question in concern:
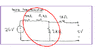
Select R in the circuit so that VL= 5 V
(see circuit in attachment below)
Hopefully you will be able to read this, I wrote it in OneNote- here is my attempt at a solution:
Select R in the circuit so that VL= 5 V
(see circuit in attachment below)
Hopefully you will be able to read this, I wrote it in OneNote- here is my attempt at a solution: