Question about arc length and the condition dx/dt > 0

[songoku]

Homework Statement

Please see below

Relevant Equations

none

This is not homework

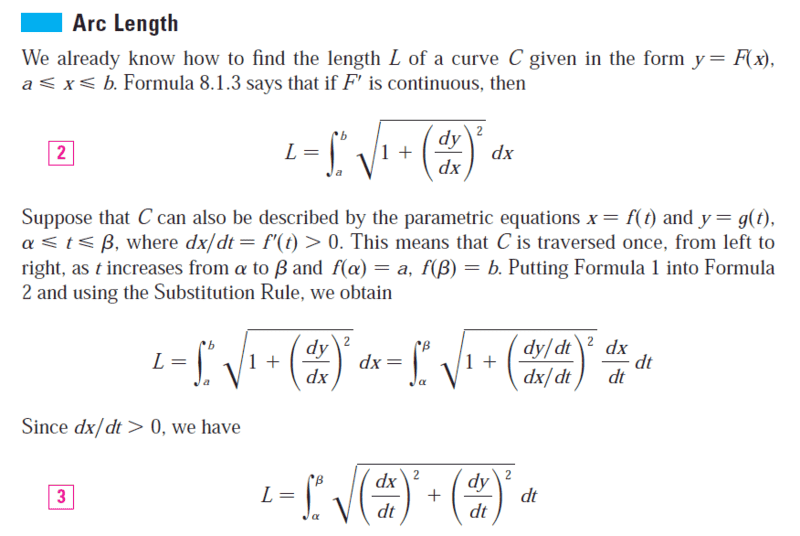
That passage is from James Stewart (Multivariable Calculus). I want to ask about the condition dx/dt > 0. If dx / dt < 0, the formula can't be used?

Thanks

 

[BvU]

Why not ? Can you find a counter-example ?

 

[songoku]

Why not ? Can you find a counter-example ?

In my opinion, it can because from the derivation I don't see the need for dx/dt to be positive.

I just don't understand why dx / dt > 0 is written there in the text.

Thanks

 

[Mark44]

I just don't understand why dx / dt > 0 is written there in the text.

If dx/dt > 0 then then x is increasing. Conversely, if dx/dt < 0 then x is decreasing.

Consider the parametric curve $x = \sin(t), y = 1$ for $t \in [0, \pi]$. Graph this simple "curve" and determine its arc length from your graph. What does the integral formula for arc length of this curve produce?

 

[pasmith]

The length of a curve should be the same, whether you start measuring it from the left end ($dx/dt > 0$) or the right end ($dx/dt < 0$). Some details of the derivation will change if $dx/dt < 0$; in particular the assumption that $f(\alpha) = a < b = f(\beta)$ must be replaced by $f(\alpha) = b > a = f(\beta)$.

 

[songoku]

If dx/dt > 0 then then x is increasing. Conversely, if dx/dt < 0 then x is decreasing.

Consider the parametric curve $x = \sin(t), y = 1$ for $t \in [0, \pi]$. Graph this simple "curve" and determine its arc length from your graph. What does the integral formula for arc length of this curve produce?

The graph is horizontal line y = 1 and 0 ≤ x ≤ 1.

I think the arc length should be 2 because the curve is traversed twice, once from left to right for 0 ≤ t ≤ π/2 and then from right to left for π/2 ≤ t ≤ π.

From integration, I get zero

The length of a curve should be the same, whether you start measuring it from the left end ($dx/dt > 0$) or the right end ($dx/dt < 0$). Some details of the derivation will change if $dx/dt < 0$; in particular the assumption that $f(\alpha) = a < b = f(\beta)$ must be replaced by $f(\alpha) = b > a = f(\beta)$.

I think I understand more clearly now from your post and Mark's example. The condition dx / dt > 0 is to ensure the curve is traversed once, only from left to right. If I want to use integration to find the arc length, I need to divide it into two cases, for 0 ≤ t ≤ π/2 and π/2 ≤ t ≤ π then subtract.

Thank you very much for the help and explanation BvU, Mark44, pasmith