Question about Coloumb's law notation and math in two different textbooks

[Selectron09]

TL;DR Summary

Trying to understand mathematically how Jackson Classical Electrodynamics and Griffiths both describe coloumb's law equation

I am currently taking Electricity and Magnetism I for Graduate school and we are of course using Jackson Classical Electrodynamics 3e. I am used to Griffiths from undergrad and intro physics in that they describe it:

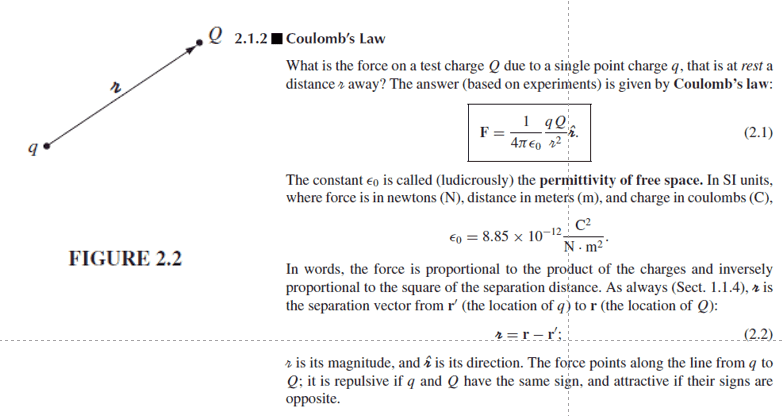
But Jackson modifies the notation to include a cube of the magnitude in the denominator:

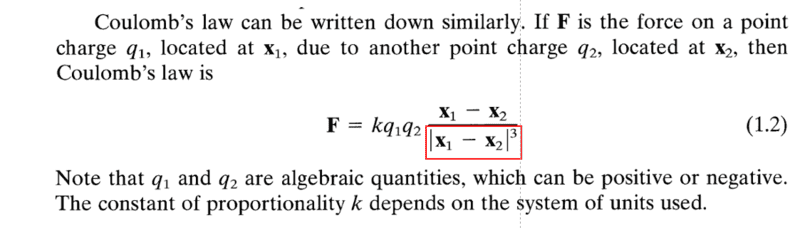
I have tried to wrap my head around it. My professor just said it makes it "less mathematically akward" which is fine. Can someone take me stepwise line by line why these two are the same? I would really appreciate it. I want to be sure that I am getting used to the notation early of Jackson as I hear that's the trickiest part. It's not "new" physics!

 

[vanhees71]

Of course, Jackson has it in the clearest way. It's just giving the force between two point charges at given positions. Of course it's the same as in Griffiths's book. You only have to look up the definition of $\vec{r}$. That becomes clear by rewriting the Jackson formula in the following way
$$\vec{F}=\frac{k q_1 q_2}{|\vec{x}_1-\vec{x}_2|^2} \frac{\vec{x}_1-\vec{x}_2}{|\vec{x}_1-\vec{x}_2} \equiv \frac{k q_1 q_2}{r^2} \hat{r},$$
where $\vec{r}=vec{x}_1-\vec{x}_2$ and $\hat{r}=\vec{r}/|\vec{r}|$.

I'd also have written $\vec{F}_1$ for the force, because it's the force on charge 1 due to the presence of charge 2. Of course, you get $\vec{F}_2=-\vec{F}_1$ as it should be for static fields.

 

[Selectron09]

Thankyou very much. That was amazingly helpful and gets me on the right track now as I continue through the reading. Much gratitude and I shall not hesitate to come back again after I've struggled through it.

 

[Meir Achuz]

The form ${\bf r}/r^3$ is more convenient for taking vector derivatives like grad, div, curl.

 

[vanhees71]

You can also work in, e.g., spherical coordinates. Then you work with vector components wrt. the according (position-dependent) vectors $\vec{e}_r$, $\vec{e}_{\vartheta}$, and $\vec{e}_{\varphi}$.