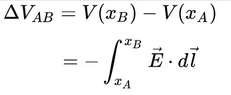Question about the integral used to calculate the voltage between two points

[lys04]

Homework Statement

If the voltage between two points is the negative integral from A to B of Edl, then in a given problem where it asks me to find the voltage between two points, how do I know which is A and which is B? Will both a negative and positive answer be accepted since its relative, like for example point A is +2V compared to B but B might be -2V compared to A?

Relevant Equations

negative integral from A to B of Edl

^^

 

[haruspex]

"between" is symmetric. It does not imply a direction, so the answer would be a magnitude. But is that the word used in the question?

 

[kuruman]

My usage of the word voltage, with which some PF users disagree, is to denote the absolute value of the potential difference between two points. If I want to refer to a quantity that could be positive or negative, i use "the electrostatic potential difference between from A to B".

When one writes $Q=CV$ for a capacitor, $V$ is called the voltage across the capacitor and is assumed to be always positive.

 

[haruspex]

"the electrostatic potential difference between from A to B"

Between? Did you mean "the electrostatic potential difference from A to B"?

 

[lys04]

"between" is symmetric. It does not imply a direction, so the answer would be a magnitude. But is that the word used in the question?

No, I am just asking generally, it hasn't come up in a question. It just confused me tho. Does magnitude mean it's always positive?

 

[lys04]

Between? Did you mean "the electrostatic potential difference from A to B"?

Idk, I saw a question online that was like find the potential difference between A and B so I got confused.

 

[lys04]

My usage of the word voltage, with which some PF users disagree, is to denote the absolute value of the potential difference between two points. If I want to refer to a quantity that could be positive or negative, i use "the electrostatic potential difference between from A to B".

When one writes $Q=CV$ for a capacitor, $V$ is called the voltage across the capacitor and is assumed to be always positive.

That makes sense. Thanks.

 

[kuruman]

Between? Did you mean "the electrostatic potential difference from A to B"?

That's what I meant. I crossed out the superfluous "between". Thanks for the catch.

No, I am just asking generally, it hasn't come up in a question. It just confused me tho. Does magnitude mean it's always positive?

The question in @haruspex's post #4 was addressed to me. It was a typo that I fixed. Sorry about the confusion.

 

[vanhees71]

But in circuit analysis you have to get the sign of the potential difference right. All these sign problems usually go away when you remember, how the corresponding quantities are derived from Maxwell's equations.