Question about this equation for the expectation value

[docnet]

TL;DR Summary

please see below

Hi all,

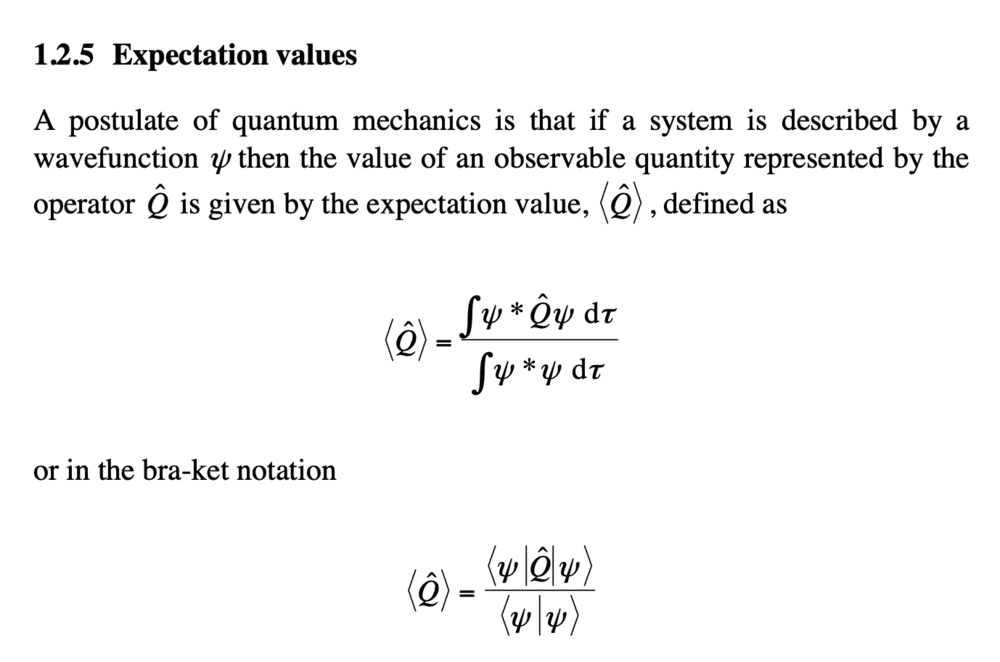
I found this notation of expectation values in a NMR text.

In class, I learned that expectation values are given by
$$<\hat{X}>=\int_{-\infty}^\infty\psi^*x\psi dx$$

why does this textbook divide by the integral of probability density $\int \psi^*\psi dx$?

 

[PeterDonis]

a NMR text.

Which textbook?

why does this textbook divide by the integral of probability density $\int \psi^*\psi dx$?

Because it is not assuming that $\psi$ is normalized. The formulas you are used to seeing are based on the assumption that $\psi$ is normalized, which is a typical assumption in QM textbooks; but that just means the integral in the denominator is $1$ so the textbooks often leave it out. A strictly correct formula keeps it in.

 

[docnet]

Which textbook?

University of Cambridge published lectures of NMR under Introduction to NMR on this website

Link: http://www-keeler.ch.cam.ac.uk/lectures/

Because it is not assuming that $\psi$ is normalized. The formulas you are used to seeing are based on the assumption that $\psi$ is normalized, which is a typical assumption in QM textbooks; but that just means the integral in the denominator is $1$ so the textbooks often leave it out. A strictly correct formula keeps it in.

Ah, that makes so much sense. Thank you I am truly glad for to have your knowledge as a resource for studying.

edited for grammar

 

[PeterDonis]

Thank you I am truly glad for to have your knowledge as a resource for studying.

You're welcome! Glad I could help.