Question about Waves -- "frequency" versus "angular frequency"

[sinus]

TL;DR Summary

The difference between f and ω as the resonant frequency in oscillation

I've been reading many references that said "frequency" and "angular frequency" are two different things. I'm writing a report about damped oscillations experiments (that's a task from a subject in my college).
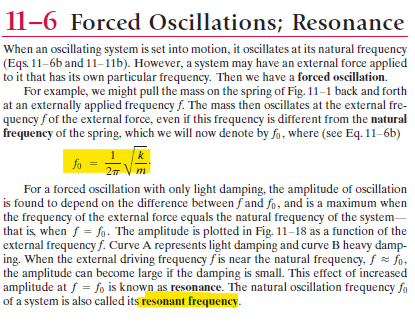
Can someone tell me which one is the resonant frequency (natural frequency)? f or ω? In Giancoli's book "Physics Principles with Application 7th Ed (2014)", it said that [f][/0] is the resonant frequency

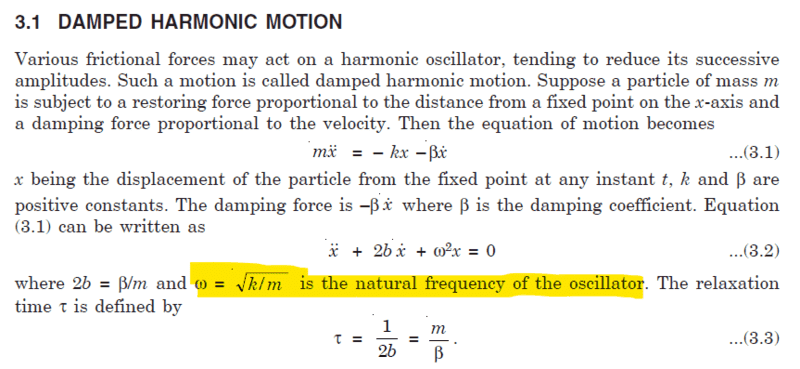
But in other references, said that [ω][/0] is the resonant frequency. This one from Chaudhuri "Waves and Oscillations (2010)"

But, f isn't equal to ω right? Their relation is showed by ω=2πf. Well, as you can see the topic of the screenshot one above is "Forced Oscillation" not "Damped Oscillation". Then, I want to ask, so if it is damped oscilations, resonant frequency is [ω][/0] and if it is forced oscillations resonant frequency is [f][/0]? Please correct me if I'm misconception.

 

[malawi_glenn]

It's just semantics. Just as when people use velocity but means speed and vice versa

 

[Ibix]

The frequency is the number of complete revolutions per unit time, and the angular frequency is the number of radians turned per unit time. It's really a matter of choice which one you use to characterise a system. It's similar to 5mph and 8kph - they're both the same speed and it doesn't matter which you use as long as you do it consistently. In the case of frequency I suspect less advanced texts will prefer to use $f$ because it's a slightly simpler concept, and more advanced texts will prefer $\omega$ since it tends to lead to fewer factors of $2\pi$ in the maths. But it makes no real difference which choice a source uses as long as they use it consistently.

For your paper I would advise making a decision over whether you prefer to use $f$ or $\omega$ and translating formulae you find in sources to your convention where necessary. You might want to note that you are doing that, at least the first time you do it, so whoever is marking it knows that you didn't drop/add the $2\pi$ factors by accident.

 

[anorlunda]

In addition to cycles per second, and radians per second, one could also use degrees per second.
But degrees per second is an inconvenient unit in most science and engineering. The only exception I can think of is rate of turn in airplane cockpits where the "standard rate of turn" is 3 degrees per second.

So everything @Ibix said is correct, but there are even more arbitrary units of angle the could be convenient for special purposes.

 

[vanhees71]

Why not gons per second? You can always add unnecessary complications to a simple issue by choosing units that are inappropriate for a given problem. In theoretical physics one uses usually $\omega=2 \pi f$ to save to write even more factors of $2 \pi$ than you have to do anyway. That's also the reason, why in modern textbooks on QT nobody uses $h$ but always $\hbar$ ;-)).