- #1
gharkness
- 1
- 0
I have a question regarding world lines and Minkowski diagrams. I have been looking everywhere for an answer and can't seem to find one, and I would just like some clarification.
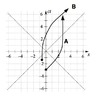
So if we have a Minkowski Diagram, with a particular world line drawn out for, let's say a particle, is the world line required to respect the asymptotes that are in the plot on the line when ct/x = 1, or is the world line simply a path through space-time, where every single point on the line is an event?
If every single line is an event, would there be an individual set of asymptotes for each, with each event having its own hyperbola?
So, is the hyperbola only for an event, and any point falling on the hyperbola for that event can be achieved by a Lorentz Transformation?
Also, if what I thought about events was true, is it possible for different events on the same world line to be both space like and time like?
I have attached a picture with a world line doing what I am asking about, in case what I said was too hard to follow.
So if we have a Minkowski Diagram, with a particular world line drawn out for, let's say a particle, is the world line required to respect the asymptotes that are in the plot on the line when ct/x = 1, or is the world line simply a path through space-time, where every single point on the line is an event?
If every single line is an event, would there be an individual set of asymptotes for each, with each event having its own hyperbola?
So, is the hyperbola only for an event, and any point falling on the hyperbola for that event can be achieved by a Lorentz Transformation?
Also, if what I thought about events was true, is it possible for different events on the same world line to be both space like and time like?
I have attached a picture with a world line doing what I am asking about, in case what I said was too hard to follow.