Question on Zeno time derivation

[Mainframes]

Hi,

I'm trying to follow the derivation of the Zeno time from two sources and am struggling. I think I'm missing some sort of algebraic trick and any tips would be appreciated. A bit more detail below.

In the attached paper \citep{Facchi_2008}, the Zeno time (equation (6)) is derived from equation (4) and equation (5), but I don't see how.

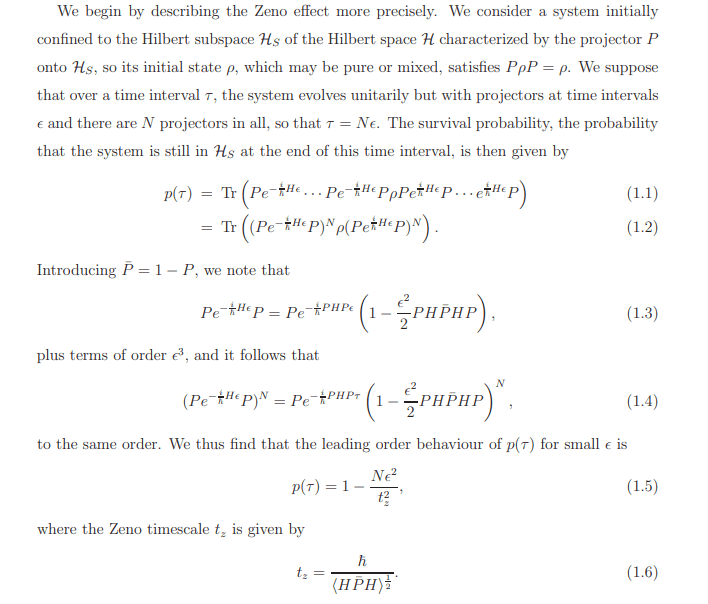
In the second attached paper \citep{PhysRevA.89.042116}, the Zeno time is derived in equation (1.6) though I cannot even see how how equation (1.3) is derived (let alone the Zeno time).

REFERENCES

@article{Facchi_2008,
doi = {10.1088/1751-8113/41/49/493001},
url = {https://dx.doi.org/10.1088/1751-8113/41/49/493001},
year = {2008},
month = {oct},
publisher = {},
volume = {41},
number = {49},
pages = {493001},
author = {P Facchi and S Pascazio},
title = {Quantum Zeno dynamics: mathematical and physical aspects},
journal = {Journal of Physics A: Mathematical and Theoretical},
}

@article{PhysRevA.89.042116,
title = {Classical limit of the quantum Zeno effect by environmental decoherence},
author = {Bedingham, D. and Halliwell, J. J.},
journal = {Phys. Rev. A},
volume = {89},
issue = {4},
pages = {042116},
numpages = {17},
year = {2014},
month = {Apr},
publisher = {American Physical Society},
doi = {10.1103/PhysRevA.89.042116},
url = {https://link.aps.org/doi/10.1103/PhysRevA.89.042116}
}

 

[Demystifier]

Hint: $P$ and $Q$ are projectors, i.e. $P^2=P$, $Q^2=Q$, $PQ=QP=0$.

 

[Mainframes]

Hint: $P$ and $Q$ are projectors, i.e. $P^2=P$, $Q^2=Q$, $PQ=QP=0$.

Thank you very much for your reply. I did try using those relationships (and I understand why they are true), however, I still could not get the algebra to work.

Furthermore, in equation (1.3) of the below extract frrom \citep{PhysRevA.89.042116}, I cannot even see how the $\epsilon^2$ term on the RHS of the equation (1.3) is possible. The reason for this is that I believe any $\epsilon^2$ term on the LHS would be of the form $\frac{\epsilon^2}{\hbar^2}$.

This, together with my inability to get the algebra to match, led me to believe I am missing something else fundamental.

 

[Demystifier]

Thank you very much for your reply. I did try using those relationships (and I understand why they are true), however, I still could not get the algebra to work.

First observe that from $P=|\psi_0\rangle\langle\psi_0|$ we have
$$P|\psi_0\rangle = |\psi_0\rangle , \;\;\; Q|\psi_0\rangle = 0.$$
The goal is to compute
$$\tau_Z^{-2} = \langle\psi_0|H^2| \psi_0\rangle - \langle\psi_0|H| \psi_0\rangle^2.$$
The second term is proportional to
$$\langle\psi_0|H| \psi_0\rangle^2=
\langle\psi_0|H| \psi_0\rangle \langle\psi_0|H| \psi_0\rangle
= \langle\psi_0|HPH| \psi_0\rangle ,$$
while the first term is
$$\langle\psi_0|H^2| \psi_0\rangle = \langle\psi_0| PH(Q+P)HP | \psi_0\rangle$$
$$=\langle\psi_0| PHQHP | \psi_0\rangle + \langle\psi_0| PHPHP | \psi_0\rangle$$
$$=\langle\psi_0| PHQQHP | \psi_0\rangle + \langle\psi_0| HPH | \psi_0\rangle$$
$$=\langle\psi_0| H_{int}^2 | \psi_0\rangle + \langle\psi_0| H | \psi_0\rangle^2 .$$
Combining all this we get
$$\tau_Z^{-2} = \langle\psi_0| H_{int}^2 | \psi_0\rangle$$
which is Eq. (6).

 

[Mainframes]

First observe that from $P=|\psi_0\rangle\langle\psi_0|$ we have
$$P|\psi_0\rangle = |\psi_0\rangle , \;\;\; Q|\psi_0\rangle = 0.$$
The goal is to compute
$$\tau_Z^{-2} = \langle\psi_0|H^2| \psi_0\rangle - \langle\psi_0|H| \psi_0\rangle^2.$$
The second term is proportional to
$$\langle\psi_0|H| \psi_0\rangle^2=
\langle\psi_0|H| \psi_0\rangle \langle\psi_0|H| \psi_0\rangle
= \langle\psi_0|HPH| \psi_0\rangle ,$$
while the first terms is
$$\langle\psi_0|H^2| \psi_0\rangle = \langle\psi_0| PH(Q+P)HP | \psi_0\rangle$$
$$=\langle\psi_0| PHQHP | \psi_0\rangle + \langle\psi_0| PHPHP | \psi_0\rangle$$
$$=\langle\psi_0| PHQQHP | \psi_0\rangle + \langle\psi_0| HPH | \psi_0\rangle$$
$$=\langle\psi_0| H_{int}^2 | \psi_0\rangle + \langle\psi_0| H | \psi_0\rangle^2 .$$
Combining all this we get
$$\tau_Z^{-2} = \langle\psi_0| H_{int}^2 | \psi_0\rangle$$
which is Eq. (6).

This is brilliant. Thank you so much for this (it has certainly Demystified the result to me)