- #1
science_world
- 7
- 0
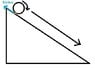
I found something interesting in the law of conservation of mechanical energy related with rotational kinetic energy. The law states that:
ME = KE(translational) + KE(rotational) + PE
*ME = Mechanical Energy
*KE = Kinetic Energy
*PE = Potential Energy
It was stated that the above law (conservation of ME) can be applied only if there was no external force (such as from friction). However, we also believe that the KE(rotational) exist because of friction (which is an external force).
So, are we contradicting ourselves? If we apply the above law, then there shouldn't be external force (friction). No friction means no KE(rotational). On the other hand, if we apply KE(rotational) law, then there exist the friction force. If friction occurs, then the above law can't be applied.
ME = KE(translational) + KE(rotational) + PE
*ME = Mechanical Energy
*KE = Kinetic Energy
*PE = Potential Energy
It was stated that the above law (conservation of ME) can be applied only if there was no external force (such as from friction). However, we also believe that the KE(rotational) exist because of friction (which is an external force).
So, are we contradicting ourselves? If we apply the above law, then there shouldn't be external force (friction). No friction means no KE(rotational). On the other hand, if we apply KE(rotational) law, then there exist the friction force. If friction occurs, then the above law can't be applied.