I Questions about a Conductor in an Electric Field

[Neitrino]

Dear PF,

I have a question regarding a conductor in electric filed. I have formulated my question in attached PDF file ... would please be so kind and advise me please...

Thanks you in advance ...

 

[willem2]

The field in area C is not zero. You argued yourself that a neutral conductor can't produce a field outside of itself, so the field in the conductor can't cancel the field of the charged plate outside of itself. This would only work with grounded conductor (wich wouldn't be neutral). You can also see this if you use gauss' law on the red and blue volumes. (which have the same enclosed charge, since the conductor is neutral)

 

[DaveE]

When charges in the conductor move to cancel the applied field they create charges on the conductor surface that reestablish an external field outside. So, if your induced field makes an electron move to the left in the conductor, that means there will be an excess of positive charge on the right. Seen externally from the right side, the conductor surface looks (sort of) like the original charged plate, with a bunch of positive charges that make a field on the right like the one on the left.

Lots of hand waving here, because the presence of the conductor does change the E-field lines nearby (boundary conditions and such), but it doesn't make it go away. When you get far away from the conductor, it should look like the conductor had no effect. Like this:

 

[Neitrino]

Dear willem2 and DaveE,

Let me ask another question...

"When you get far away from the conductor, it should look like the conductor had no effect. Like this:"

When an initial charged (with charge +Q) plate creates an electric filed it originates from the charged surface and penetrates into area A, then it penetrates into area B (inside the conductor thickness) where it is canceled by the induced filed - so if it is canceled inside the conductor how / why does it propagate onwards - into area C ?

Thank you so much for you kind explanations

 

[DaveE]

Dear willem2 and DaveE,

Let me ask another question...

"When you get far away from the conductor, it should look like the conductor had no effect. Like this:"

When an initial charged (with charge +Q) plate creates an electric filed it originates from the charged surface and penetrates into area A, then it penetrates into area B (inside the conductor thickness) where it is canceled by the induced filed - so if it is canceled inside the conductor how / why does it propagate onwards - into area C ?

Thank you so much for you kind explanations

The field inside the conductor, induced by charge displacement, can be thought of as originating at the + charges on the left surface and ending at the - charges at the right surface. This field cancels the externally generated field inside the conductor, but isn't (very) present outside of the conductor. From far away, the shift of charges inside the conductor isn't significant, an observer out there just sees + and - charges in a relatively small space far away that cancel each other.

 

[hutchphd]

When an initial charged (with charge +Q) plate creates an electric filed it originates from the charged surface and penetrates into area A, then it penetrates into area B (inside the conductor thickness) where it is canceled by the induced filed - so if it is canceled inside the conductor how / why does it propagate onwards - into area C ?

If the conductor is not infinite in extent then the induced charge distributions on the surface will not be uniform. I believe you are using the approximation that the conductor is finite and that it is not in the same problem.

 

[alan123hk]

From the sketch below, it can be seen that after the conductor is added, the electric field in all spaces does not change, and of course the electric field inside the conductor is also zero.

 

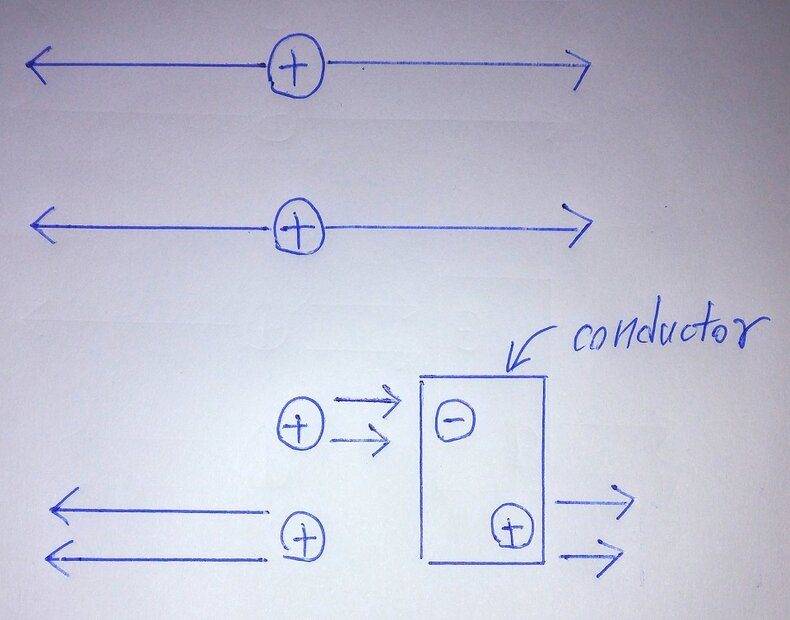
[SDL]

It actually does, don't forget you conductor sample is finite. The external electric field is always normal to a conductor's surface. If you insert a rectangular conductor into a uniform field, like it's shown on your drawing, what this field at the upper and the lower surfaces would be?

 

[vanhees71]

What happens is that when putting the conductor into the field, the conduction electrons rearrange such that the interior of the conductor gets field free (for electrostatics), and the conductor gets a corresponding surface-charge density distribution. This rearrangement of charges leads also to a change of the electrostatic field outside of the conductor. The most simple example, which can be analytically solved using the "image-charge method", is a conducting sphere in the field of a single point charge (outside of the sphere).

 

[alan123hk]

It actually does, don't forget you conductor sample is finite. The external electric field is always normal to a conductor's surface. If you insert a rectangular conductor into a uniform field, like it's shown on your drawing, what this field at the upper and the lower surfaces would be?

 

[SDL]

Ok, something like that. But what about this:

after the conductor is added, the electric field in all spaces does not change

?
The field can't stay unchanged: first, it becomes non-uniform to satisfy boundary conditions and, second, the exterior field is now the sum of the original one and the field produced by the induced charges.

 

[alan123hk]

What I mean of course is that when both the electric field and the conductor extend to infinity, the electric fields in all spaces A, B, and D have no change after adding the conductor. The electric field in space C inside an ideal conductor is always zero, so it doesn't change either.

If the conductor does not extend to infinity, then of course simply means that there is no change in the electric fields A, B, D near the middle position.

I believe that what the OP was mainly interested in studying was the electric field in spaces A, B, C and D, not other more complicated situations.

BTW, the diagram below is something I came up with, I believe it has never been seen before, I am very happy with this diagram.

 

[hutchphd]

BTW, the diagram below is something I came up with, I believe it has never been seen before, I am very happy with this diagram.

It is good that you are happy but the diagram is incorrect. Your supposition is true only for flat sheets that have no edges (are of infinite extent). The real world is considerably more complicated.... so much for happiness.

 

[alan123hk]

The question posed by the OP is now clearly explained graphically, the remaining question that may be asked is what is the net force acting on the conductor? Will it stay still, move left, or move right?