Dario56
- 289
- 48
Bragg's law is schematically shown on the picture:
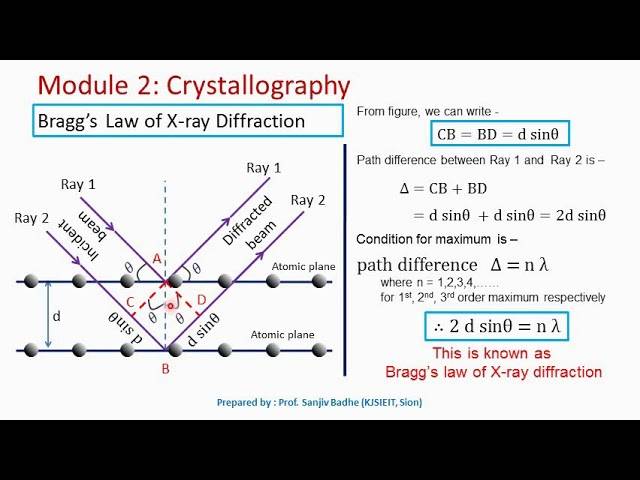 Two parallel and plane waves are shown which propagate towards the crystal. For plane waves, wave fronts are flat planes perpendicular to the wave propagation with infinite size. In reality, there are no plane waves. Nevertheless, they are used as they are often a good approximation.
Two parallel and plane waves are shown which propagate towards the crystal. For plane waves, wave fronts are flat planes perpendicular to the wave propagation with infinite size. In reality, there are no plane waves. Nevertheless, they are used as they are often a good approximation.
I have two questions:
1) How do we know that the interference of two waves hadn't happened before both waves interacted with the lattice? To be more precise, waves shouldn't interfere before the left ray reaches point D as we want that the left ray travels that extra distance (from C to D) to see what kind of interference result we get. Wave fronts of real waves extend in space as wave propagates and so interference may happen before both waves interacted with the lattice (before left wave reached point D) . If that happens, there is no much use in the Bragg's law.
2) Bragg's law is based on the interference of two parallel waves. Scattering of the x-rays happens because electrons absorb x-rays and re-emit them, but they do so randomly, in all directions. Constructive interference can happen if waves of different propagation directions interfere and so, if we see the bright or dark spot of the interference, how can we know that in fact two parallel waves actually interfered to give that spot?
I have two questions:
1) How do we know that the interference of two waves hadn't happened before both waves interacted with the lattice? To be more precise, waves shouldn't interfere before the left ray reaches point D as we want that the left ray travels that extra distance (from C to D) to see what kind of interference result we get. Wave fronts of real waves extend in space as wave propagates and so interference may happen before both waves interacted with the lattice (before left wave reached point D) . If that happens, there is no much use in the Bragg's law.
2) Bragg's law is based on the interference of two parallel waves. Scattering of the x-rays happens because electrons absorb x-rays and re-emit them, but they do so randomly, in all directions. Constructive interference can happen if waves of different propagation directions interfere and so, if we see the bright or dark spot of the interference, how can we know that in fact two parallel waves actually interfered to give that spot?