Quick spherical coordinate question

In summary, the speaker is asking for help calculating the inertia matrix for a given shape using spherical coordinates. They explain their chosen limits of integration for theta and phi, and ask for confirmation on their choice. The responder suggests different limits and the speaker agrees that it is working.
  • #1
Shaybay92
124
0
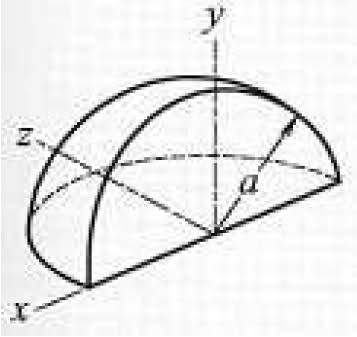
So I have the following shape for which I want to calculate the inertia matrix. Basically I just want to know what limits of integration I should use if I am using spherical coordinates. Assume the convention that phi is the angle from x to y in the xy plane and theta is from z to the xy plane. Thus the limits I chose were [theta, -pi/2,pi/2] and [phi,0,pi/2]. Do you agree with these? The reason I ask is I am getting zero for my calculation of Izz.

Thanks in advance.
9uox75.jpg

Homework Statement


Homework Equations


The Attempt at a Solution

 
Physics news on Phys.org
  • #2
if your defining theta from the positive z axis then i think theta should go from 0 to pi/2
and i think phi is from 0 to pi .
 
  • #3
Thanks so much! I just wasn't visualizing it properly, and I used those values and it seems to be working :D
 
  • #4
no problem
 

FAQ: Quick spherical coordinate question

What are spherical coordinates?

Spherical coordinates are a system of coordinates used to locate a point in three-dimensional space. They consist of a distance from the origin, an angle from the positive x-axis, and an angle from the positive z-axis.

How are spherical coordinates different from Cartesian coordinates?

Spherical coordinates use angles and a distance from the origin to locate a point, while Cartesian coordinates use x, y, and z coordinates. Spherical coordinates are useful for describing points on a sphere or in a three-dimensional space, while Cartesian coordinates are useful for describing points in a flat, two-dimensional space.

What is the formula for converting spherical coordinates to Cartesian coordinates?

The formula for converting spherical coordinates to Cartesian coordinates is x = r * sin(theta) * cos(phi), y = r * sin(theta) * sin(phi), and z = r * cos(theta), where r is the distance from the origin, theta is the angle from the positive z-axis, and phi is the angle from the positive x-axis.

How are spherical coordinates used in physics?

Spherical coordinates are often used in physics to describe the position, velocity, and acceleration of objects in three-dimensional space. They are also used in equations for electric and gravitational fields, as well as in fluid dynamics and quantum mechanics.

What are some real-world applications of spherical coordinates?

Spherical coordinates are used in many real-world applications, such as navigation systems, astronomy, and computer graphics. They are also used in engineering for designing and analyzing structures and in oceanography for mapping and studying ocean currents.

Similar threads

How to calculate a sink using spherical coordinates
Replies
7
Views
990
Center of mass of spherical shell inside of cone (Apostol Problem).
Replies
3
Views
1K
Surface area of a shifted sphere in spherical coordinates
Replies
3
Views
3K
Represent a 3d region and compute this triple integral
Replies
4
Views
1K
Line integral in spherical coordinates
Replies
4
Views
8K
How to find the curl of a vector field which points in the theta direction?
Replies
33
Views
4K
Surface Integral of a sphere
Replies
9
Views
769
Vector Field Transformation to Spherical Coordinates
Replies
5
Views
1K
Substituting spherical coordinates to evaluate an integral
Replies
1
Views
1K
Element of surface area in spherical coordinates
Replies
7
Views
4K

Hot Threads

Recent Insights

Back
Top