Radius of gyration for a flywheel

[NEGATIVE_40]

Homework Statement

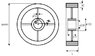
What is the radius of gyration (in meters) for the steel flywheel shown? The width of its rim, L, is as given below. The density of steel is 7500 kg/m3. The outside diameter (OD)for the wheel is 2000 mm, and the inside diameter (ID) is 1840 mm as shown in the figure. The spokes are of cylindirical cross section with the diameter 70 mm. The hub OD and ID are 400 mm and 200 mm, respectively. The hub width is half of the rim width.

*see attachment for diagram*

Homework Equations

$$k= \sqrt{\frac{I}{m}}$$, where k is the radius of gyration, I is the moment of inertia and m is the mass
$$I_x=\frac{1}{4} mR^2$$ for a thin disk (I used the formula for circular cylinder initially, but it just reduced to this anyway)
$$I_y= \frac{1}{12}m(3R^2+l^2) +m \bar{y}^2$$ for circular cylinder, in my case it is for the vertical spokes.

The Attempt at a Solution

I started by finding the total mass of the flywheel, and I am fairly sure I have that part correct

The part where I think I am making my mistake is in calculating the moments of inertia.
for the circular bits
$$I_x =\frac{m_{big~circular ~bit}}{4}(R_{BO}^2-R_{Bi}^2)+ \frac{m_{little~circular~bit}}{4}(R_{SO}^2-R_{Si}^2)$$
then for the horizontal spokes:
$$I_{x, horizontal spokes} = 2*\left [ \frac{1}{2}m_{one~spoke}R^2 \right ]$$ where R is the radius of the spoke.
for the vertical spokes using parallel axis theorem:
$$I_{x, vertical spokes} = 2*\left [ \frac{1}{12}m_{one~spoke}(3R^2+l^2) +m_{one~spoke} \bar{y}^2 \right ]$$

I've then added all of these moments of inertia together and used
$$k= \sqrt{\frac{I}{m}}$$
but my answer is wrong.

Have I missed anything in my working, or used the wrong inertia formula somewhere?
Am I supposed to take the moment of inertia about the x – axis in this case? (would it make a difference if I did it about another axis?)

This question has really got me confused, so any help would be appreciated!

 

[SteamKing]

I would expect the problem is looking for the gyradius of the flywheel about the axis of rotation (or z-axis from the attached diagram, the axis perpendicular to the sketch).

Your calculation of the MOI of the spokes is puzzling. I think you took the right approach with the vertical spokes, but you should have taken the same approach with the horizontal spokes as well. They are rotating about an axis which is located a certain distance away from the centroid of the individual spoke.

For the cylindrical portions of the flywheel (the hub and the outer rim), I think your calculation of the MOI uses an incorrect formula. The depth of the cylinders should figure into the MOI calculation in addition to the radii (and separately from the calculation of the mass). I think the formulas in the OP are for thin disks.

 

[NEGATIVE_40]

thanks for your reply.

for the cylindrical portions I'm now using the MOI of a circular cylinder of length L about z axis (as I have the axis set up, z coming out of the page)
$$I_z= \frac{1}{2}m(R_{Bo}^2-R_{Bi}^2)$$
which is actually the same as that of a thin disk. The difference would be in m, the mass.
and then I did the same for the inner hub.

I think I'm a bit confused about the spokes. Since they are both rotating about the z-axis, they would have the same MOI, right?
$$I_{z} = 4*\left [ \frac{1}{12}m_{one~spoke}(3R^2+l^2) +m_{one~spoke} \bar{y}^2 \right ]$$
where I could just say y bar is the distance from the centroid of the spoke to the centroid of the flywheel.

Does this approach look correct?

 

[SteamKing]

The spokes look OK.

If you check this link: http://en.wikipedia.org/wiki/List_of_moments_of_inertia

You'll see that the MOI calculation for the flywheel hub and rim in the OP are indeed incorrect.

 

[NEGATIVE_40]

Okay it all works out now. I had to add, not subtract, the radii for the MOI in the circular section.
Thanks.