Radius of Smallest Circle in 6 Circles Problem (10cm)

  • Thread starter Thread starter KKW
  • Start date Start date
  • Tags Tags
    Circles
KKW
Messages
3
Reaction score
0
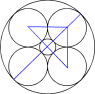
If you inscribe 4 circles inside a bigger circle and then add another smaller circle, touching the other 4 circles, what is the radius of the smallest circle (in the centre) if the radius of the biggest circle is 10cm?
 
Mathematics news on Phys.org
Is the radius of the four circles the same?
 
Assuming four circles are identical, that's everything you may need. Think how these are related to the radii of circles in question.
 

Attachments

  • 6_circles_question.png
    6_circles_question.png
    9.8 KB · Views: 492

Thread 'What Exactly is Dirac’s Delta Function? - Insight'

Insights auto threads is broken atm, so I'm manually creating these for new Insight articles. In Dirac’s Principles of Quantum Mechanics published in 1930 he introduced a “convenient notation” he referred to as a “delta function” which he treated as a continuum analog to the discrete Kronecker delta. The Kronecker delta is simply the indexed components of the identity operator in matrix algebra Source: https://www.physicsforums.com/insights/what-exactly-is-diracs-delta-function/ by...
View full post »

Thread 'Fermat's Last Theorem'

Fermat's Last Theorem has long been one of the most famous mathematical problems, and is now one of the most famous theorems. It simply states that the equation $$ a^n+b^n=c^n $$ has no solutions with positive integers if ##n>2.## It was named after Pierre de Fermat (1607-1665). The problem itself stems from the book Arithmetica by Diophantus of Alexandria. It gained popularity because Fermat noted in his copy "Cubum autem in duos cubos, aut quadratoquadratum in duos quadratoquadratos, et...
View full post »

Thread 'Useless continued fraction for 1'

I'm interested to know whether the equation $$1 = 2 - \frac{1}{2 - \frac{1}{2 - \cdots}}$$ is true or not. It can be shown easily that if the continued fraction converges, it cannot converge to anything else than 1. It seems that if the continued fraction converges, the convergence is very slow. The apparent slowness of the convergence makes it difficult to estimate the presence of true convergence numerically. At the moment I don't know whether this converges or not.
View full post »

Similar threads

I Geometry problem of interest with a 3-4-5 triangle
2
Replies
59
Views
1K
MHB Area of multiple circles inside a rectangle
Replies
3
Views
2K
B Inscribing a circle in an Oblique Square (Drafting)
Replies
9
Views
1K
I Equation for circle points in 3D
Replies
3
Views
3K
B The Pitcher's Mound
Replies
10
Views
2K
MHB AO+BO+CO≥6r where r is the radius of the inscribed circle
Replies
2
Views
1K
B Understanding why πr^2 works for different area calculations
Replies
7
Views
2K
MHB Calculating Geometric Probability on a Round Table
Replies
3
Views
2K
MHB Relationship between inner and outer radius of a two concentric circles
Replies
2
Views
2K
MHB Tangent line to circle making 30º with a second circle
Replies
2
Views
2K

Hot Threads

Recent Insights

Back
Top