- #1
Oliver321
- 59
- 5
I am starting to learn classical physics for my own. One exercise was, to calculate the vector r (see picture: 1.47 b). The vector r is r=z*z+p*p.
I don’t understand this solution. My problem is: in a vector space with n dimensions there are n basis vectors. In the case of cylindrical coordinates there have to be 3 basis vectors: z,p,phi. (How this basis vectors are defined, is listed in Wikipedia)Every vector in a vector space can be built from a minimum of 3 basis vectors. So we also need all three basis vectors to construct r. But why are we using online two of them? r could possibly point in any direction?
I appreciate every help! Thank you!
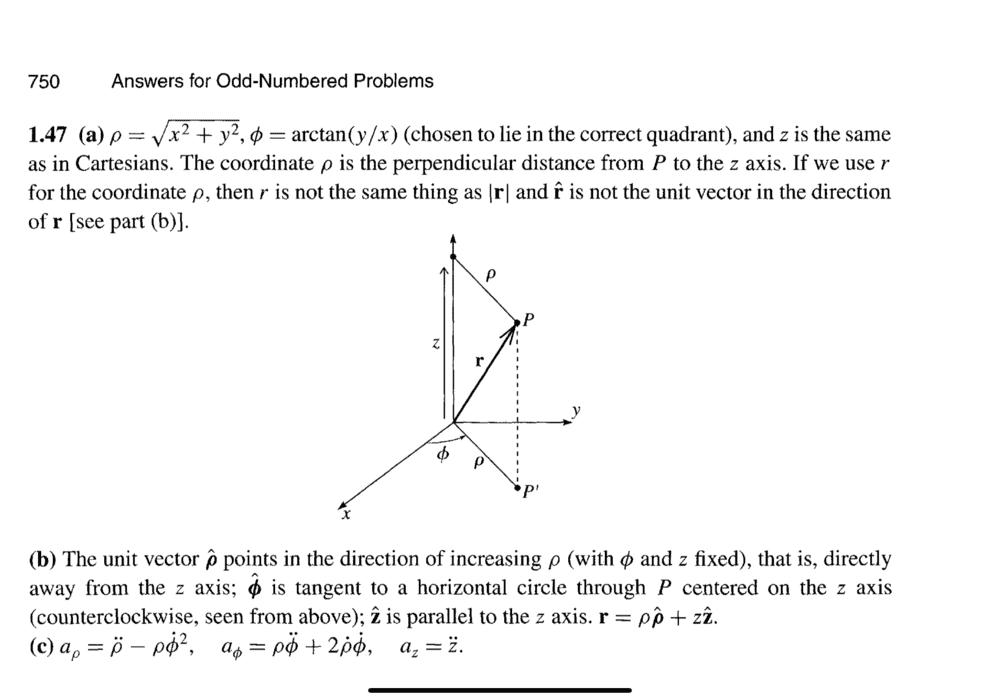
I don’t understand this solution. My problem is: in a vector space with n dimensions there are n basis vectors. In the case of cylindrical coordinates there have to be 3 basis vectors: z,p,phi. (How this basis vectors are defined, is listed in Wikipedia)Every vector in a vector space can be built from a minimum of 3 basis vectors. So we also need all three basis vectors to construct r. But why are we using online two of them? r could possibly point in any direction?
I appreciate every help! Thank you!
