yonese
- 15
- 1
[Mentor Note -- Thread moved from the technical forums, so no Homework Template is shown]
Hi all,
Recently I 'did' (a virtual lab) a drag laboraty experiment that used a wind tunnel to measure drag coefficient of 3 different shapes (cylinder, airfoil, triangular prism) and I'm not convinced the graph I've plotted is right.
The aim of the experiment was to obtain the drag coefficients for each body, for a range of air flow rates. When the fan in the wind tunnel is on and the valve is open, air impacts the object installed in the test section and a drag force is generated on the object. The objective of the experiment is to measure this drag force at different valve openings (i.e. different flow speed).
From this experiment, I've plotted a graph of each shape's drag coefficient vs Reynold's number (See below). The characteristic length were the same for the cylinder and prism, and the airfoil had a slightly larger value. I had expected the airfoil to have a smaller drag coefficient as it is streamlined and I expected the prism to have the highest drag, both of which are shown in the graph, so that's fine. However, something doesn't seem right. Firstly, the airfoil has such a large range of Reynold's number compared to its other shapes. Secondly, the prism's Reynold's number has a minimum of 7400, which means it's in the transitional state rather than turbulent like the other two shapes.
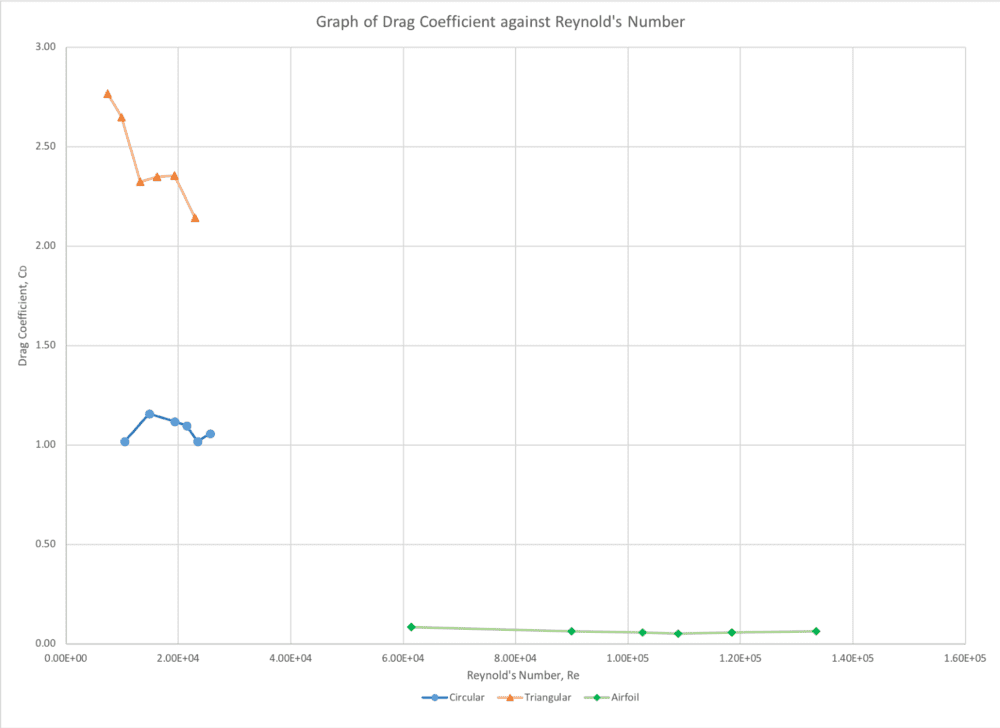
I have included the actual values below, if it is any useful.
The average drag coefficients were 1.08, 2.43 and 0.063 for the cylinder, triangular and airfoil prisms, respectively. I compared these to an external literatures which had values 1.20, 2.0 and 0.045 respectively. So, I wouldn't say I'm too far off but something about the graph doesn't seem right.
I'm also not sure whether I should be using the average drag coefficient when comparing it to other resources, or if I use the fully open valve values.
Thanks in advance.
Hi all,
Recently I 'did' (a virtual lab) a drag laboraty experiment that used a wind tunnel to measure drag coefficient of 3 different shapes (cylinder, airfoil, triangular prism) and I'm not convinced the graph I've plotted is right.
The aim of the experiment was to obtain the drag coefficients for each body, for a range of air flow rates. When the fan in the wind tunnel is on and the valve is open, air impacts the object installed in the test section and a drag force is generated on the object. The objective of the experiment is to measure this drag force at different valve openings (i.e. different flow speed).
From this experiment, I've plotted a graph of each shape's drag coefficient vs Reynold's number (See below). The characteristic length were the same for the cylinder and prism, and the airfoil had a slightly larger value. I had expected the airfoil to have a smaller drag coefficient as it is streamlined and I expected the prism to have the highest drag, both of which are shown in the graph, so that's fine. However, something doesn't seem right. Firstly, the airfoil has such a large range of Reynold's number compared to its other shapes. Secondly, the prism's Reynold's number has a minimum of 7400, which means it's in the transitional state rather than turbulent like the other two shapes.
I have included the actual values below, if it is any useful.
| Reynold's Number, Re | |||
| Valve pos. (mm) | Cylinder | Triangular Prism | Airfoil |
| 1 (Fully opened valve) | 2.57E+04 | 2.29E+04 | 1.33E+05 |
| 0.9 | 2.34E+04 | 1.93E+04 | 1.18E+05 |
| 0.7 | 2.15E+04 | 1.62E+04 | 1.09E+05 |
| 0.5 | 1.93E+04 | 1.32E+04 | 1.03E+05 |
| 0.3 | 1.48E+04 | 9.93E+03 | 9.00E+04 |
| 0.1 | 1.05E+04 | 7.40E+03 | 6.15E+04 |
| 0 (Closed valve) | 0 | 0 | 0 |
The average drag coefficients were 1.08, 2.43 and 0.063 for the cylinder, triangular and airfoil prisms, respectively. I compared these to an external literatures which had values 1.20, 2.0 and 0.045 respectively. So, I wouldn't say I'm too far off but something about the graph doesn't seem right.
I'm also not sure whether I should be using the average drag coefficient when comparing it to other resources, or if I use the fully open valve values.
Thanks in advance.
Last edited by a moderator: