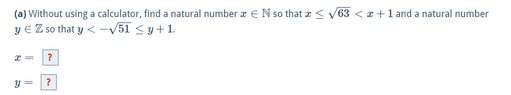Rationalising Surds: Get the Answers You Need - Daniel

[danielw]

Hi All

This is my question.

View attachment 5871

I don't know how to begin working on it.

I already tried simplifying the first part to: $$ \sqrt{63} = \sqrt{7 \cdot 3^3}=\sqrt{7}\sqrt{3^2}=3\sqrt{7}$$

But this doesn't get me closer to answering the first part of the question, and I think the same technique will apply to the second part. I would be grateful for some guidance!

Thanks.

Daniel

 

[MarkFL]

To find $x$, consider:

$$x^2\le63<(x+1)^2$$

So, think of the perfect squares that will fit the bill here:

$$7^2\le63<8^2$$

Thus, $x=7$...can you now find $y$?

 

[danielw]

To find $x$, consider:

$$x^2\le63<(x+1)^2$$

So, think of the perfect squares that will fit the bill here:

$$7^2\le63<8^2$$

Thus, $x=7$...can you now find $y$?

So since $$y^2=-(51)$$, $$y$$ is between 7 ($$7^2=49$$) and 8 ($$8^2=64$$), $$y=7$$?

 

[MarkFL]

The question for finding $y$ has an error in it...we are looking for an integer rather than a natural number. Natural numbers are denoted by $\mathbb{N}$ whereas integers are denoted by $\mathbb{Z}$.

We observe that $y$ must be a negative number (do you see why?), and so we can write:

$$(y+1)^2\le51<y^2$$

Since all 3 values are negative, we change the direction of the inequality when squaring.

And if we write:

$$7^2\le51<8^2$$

We may then write:

$$y^2=8^2$$

And we take the negative root here, to obtain:

$$y=-8$$

Does this make sense?