- #1
larocket83
- 1
- 0
I'm pretty rusty on my statics. I'm wondering how I could determine the reaction at four points arranged in a rectangle, with a force acting in the x direction at a distance away from the plate I have shown in this graphic. I seem to remember the term statically indeterminate, I think this applies, but is there any way to figure out the forces that each of these corners see? (Assume the points are fixed to the floor.)
Thanks in advance.
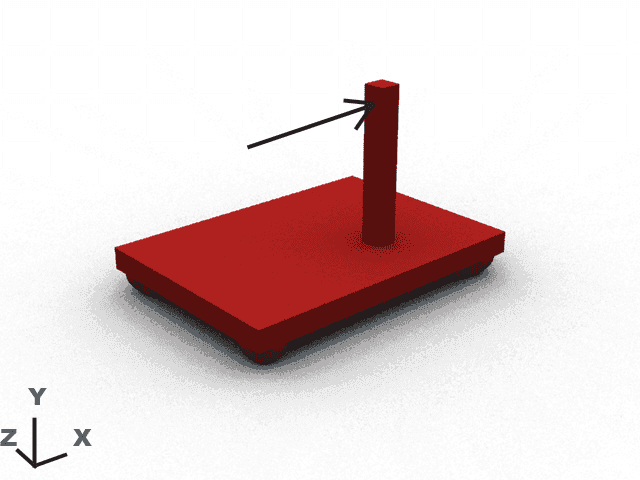
Thanks in advance.