Reaction forces in a Static system

[jen]

Homework Statement

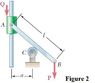
Rod AB is attached to a collar at A and rests against a roller at C.
a = 107mm,
l = 642mm,
P = 110kN and
Q = 30kN, determine:
The reaction at A (by the vertical rod) (Unit: kN)
The magnitude of the reaction at C (Unit: kN)

Homework Equations

This is my first ever post and I'm not sure how to attach an image i have one but it doesn't have a URL can you just copy and paste images?

For now ill try and explain it in words.
So imagine if you will a smooth vertical rod with a collar that can slide up and down. attached to the collar is a rod(AB) the point where the rod meets the collar is point A and the end of the Rod is point B. The rod goes down and to the right from point A resting midway on a roller point C. The total length of the rod is 642 mm. The roller is 107 mm to the right of the vertical rod that is to say the horizontal distance between point A and point C is 107 mm. At point A there is a force of 30kN down and at point B there is a force of 110kN down

The Attempt at a Solution

The first thing I did was draw a free body diagram it was a diagonal line going from point A down and to the right to point B. I drew the two know forces both down at points A and B. At point A I put a reaction force horizontal to the left and at point C I put a reaction force perpendicular to the rod AB. I then split the reaction at C into horizontal and vertical components. As this system is static the sum of the forces in the x and y direction as well as the sum of the moments is 0 so I calculated that the vertical component of the reaction at C must be 110+30=140kN and that the horizontal component must be equal to the reaction at point A. My problem now is that i can't solve anything else mainly because i can't figure out what the angle of the rod is. I have tried calculating the moment around point A and point C both give me equations i can't solve without the aid of a graphics calculator and even then i get multiple answers. I have been nutting over this for almost a week and I'm starting to think there might not be enough information to resolve it. I know that if i knew the angle of the rod or the point along the rod where C was then i could solve it. please help me.

 

[SteamKing]

Yes, you can upload image files and other files from your computer to PF. Just go to the advanced tab and select the paper clip icon, which opens a dialog box asking you for the files to upload.

A picture would go a long way to describing this problem.

 

[jen]

thanks

 

[SteamKing]

I think the way to attack this problem is to observe that when equilibrium is achieved, the sum of the moments about C must be zero. The equilibrium angle the bar AB makes with the horizontal is going to depend on the values of the forces Q and P.

 

[jen]

i tried to do that but i can't get it to come out.
this is how i did it
lets call the horizontal reaction at A ra and the angle between the vertical bar and the rod AB angle c
then the sum of the moments about C is
Mc= 30*.107+ra*.107/sin(c)-110*(.624*sin(c)-.107)

and ra is 140*sin(c)

therfore
Mc=30*.107+(140*sin(c))*(.107/sin(c))-110*(.642*sin(c)-.107)=0
but when i solve this i get some really weird stuff is the logic around what I'm doing right here

 

[SteamKing]

It's not clear what you mean by 'really weird stuff' when you solve for angle c. Sasquatch? Chupacabra? Yeti? Can you provide more detail?

 

[jen]

basically I'm looking for a single answer for c but i get a sin wave and don't know where to go from there.

 

[jen]

Do you think you could try and solve it with pen and paper and tell me if you can do it

 

[SteamKing]

For your equation, there will be one basic value of angle 'c' which makes the equation true. Since you have trig functions, which are periodic, c + 2kπ, where k is any integer, will also be a solution.
You can use trial and error or make a plot of the equation to solve it.