MHB Rebekah's question at Yahoo Answers involving inverse trigonometric functions

[MarkFL]

Here is the question:

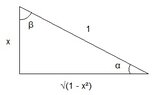
Given 0<x<orequalto 1, determine the value of inversine (x) + inversetan (squareroot(1-x^2)/x)?

Here is a link to the question:

Given 0<x<orequalto 1, determine the value of inversine (x) + inversetan (squareroot(1-x^2)/x)? - Yahoo! Answers

I have posted a link there to this topic so the OP may find my response.

 

[MarkFL]

Hello Rebekah,

We are given to evaulate:

$\displaystyle \sin^{-1}(x)+\tan^{-1}\left(\frac{\sqrt{1-x^2}}{x} \right)$

where $0<x\le1$

Let's draw a diagram of a right triangle where:

$\displaystyle \alpha=\sin^{-1}(x)\text{ and }\beta=\tan^{-1}\left(\frac{\sqrt{1-x^2}}{x} \right)$:

View attachment 616

Now, it is easy to see that $\alpha$ and $\beta$ are complementary, hence:

$\displaystyle \alpha+\beta=\sin^{-1}(x)+\tan^{-1}\left(\frac{\sqrt{1-x^2}}{x} \right)=\frac{\pi}{2}$