Reduction of order for Second Order Differential Equation

[member 731016]

Homework Statement

Please see below. I am trying to find the reduction of order for a second order ODE using an alternative method than shown in the textbook.

Relevant Equations

Ansatz $v(t) = e^{rt}$

For this,

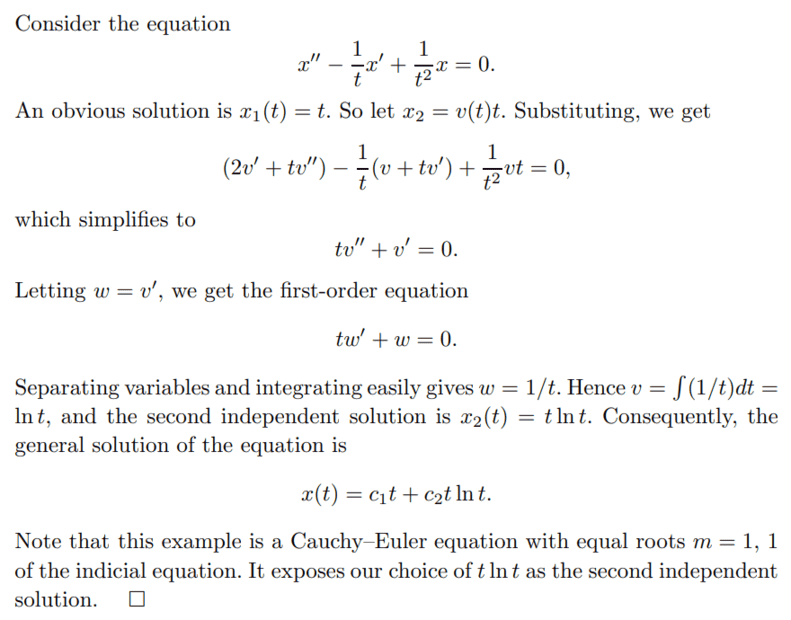
I tried solving the differential equation using an alternative method. My alternative method starts at

$tv^{''} + v^{'} = 0$
I substitute $v(t) = e^{rt}$ into the equation getting,
$tr^2e^{rt} + re^{rt} = 0$
$e^{rt}[tr^2 + r] = 0$
$e^{rt} = 0$ or $tr^2 + r = 0$
Note that $e^{rt} ≠ 0$
$tr^2 + r = 0$
$r(tr + 1) = 0$
$r = 0$ or $r = -\frac{1}{t}$

Thus, $v_1 = e^0 = 1$ and $v_2 = e^{-1} = \frac{1}{e}$

Note that $v_1 = 1$ is a trivial solution since it is just $x_2 = t = x_1$, however, for $v_2$, we get $x_2 = \frac{t}{e}$.

However, $x_2 = \frac{t}{e}$ is just another multiple of $x_1$. Is it possible to get $t\log_e(t)$ using my method?

Thanks.

Any help greatly appreciated - Chiral.

 

[Frabjous]

You are assuming v(t)=e^rt to try to get the solution v(t)=ln(t). Do you see the problem?

 

[Orodruin]

Your method is invalid. You assume $e^{rt}$ is a solution with constant $r$ and then arrive at $r = -1/t$. By coincidence $r=0$ makes your ansatz coincide with one of the solutions, but when you find non-constant $r$ you should realize that your ansatz did not work.

Why is this the case? The ansatz $e^{rt}$ is a good ansatz for ODEs with constant coefficients. Your ODE does not have constant coefficients. Instead, it is of Caucy-Euler form.