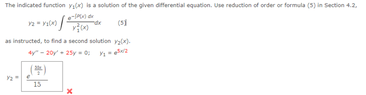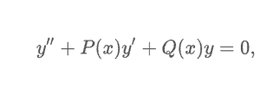Reduction of Order Problem for Differential Equations Class

[M87TJC]

Homework Statement

Find second solution for differential equation using reduction of order (see first image)

Relevant Equations

equation labeled (5) in first image in addition to equation 1

Problem statement:

Second order linear differential equation in standard from

Reasoning:

 

[M87TJC]

I just looked back at the original problem, and I realized that I did not put the equation into standard form. If I divide the equation by 4 and repeat the same process I get the correct answer.