Related rates calculus problem about water tank

In summary, we are asked to find the amount of water that comes out in each of the 100 holes in a rectangular water tank with a base length of 200 cm, given that the measurement of the water's height in the tank may have an error of ± 1 cm. This can be solved using differential equations.
  • #1
jaychay
58
0
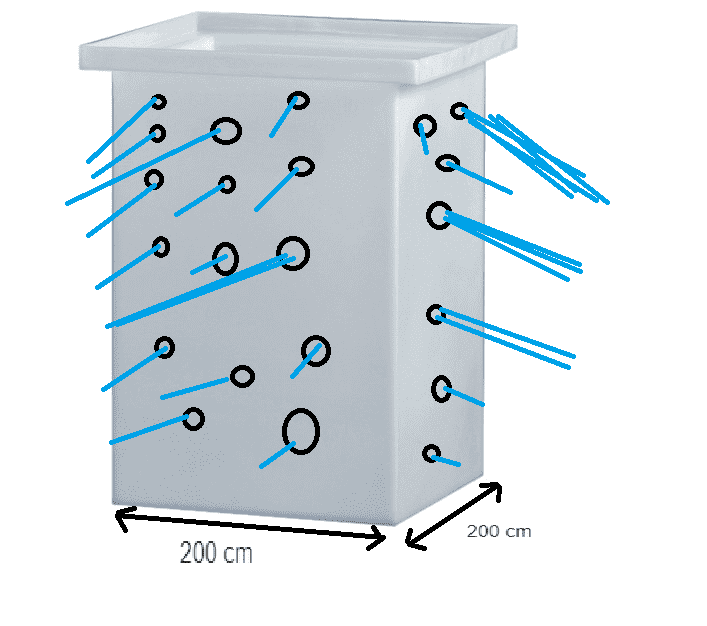
Consider the rectangular water tank, at the base the length is the same for 200 cm. There are 100 holes for water to come out which each hole have the same flow rate. Find the amount of water that come out in each hole by using differential when we know that there is an error in the measurement of the height of the water in the tank is not exceed ± 1 cm. ?

tank.png
 
Last edited by a moderator:
Physics news on Phys.org
  • #2
Your problem seems to be incomplete. Is there more information?
 
  • #3
Klaas van Aarsen said:
Your problem seems to be incomplete. Is there more information?
The problem is completed and I can now find the answer to this problem
By the way, thank you very much for helping me with the problems many times Mr.Klaas van Aarsen :)
 

FAQ: Related rates calculus problem about water tank

What is a related rates calculus problem about a water tank?

A related rates calculus problem about a water tank involves finding the rate of change of a quantity, such as the water level in the tank, as it relates to other changing quantities, such as the rate of water flow into the tank.

How do you set up a related rates calculus problem about a water tank?

To set up a related rates calculus problem about a water tank, you will need to identify all the changing quantities involved, such as the water level, the rate of water flow, and the dimensions of the tank. Then, you can use the chain rule to express the rate of change of one quantity in terms of the others.

What are some common real-life applications of related rates calculus problems about water tanks?

Some common real-life applications of related rates calculus problems about water tanks include determining the rate at which a swimming pool is being filled or drained, calculating the rate at which water is being pumped into or out of a storage tank, and finding the rate at which water is leaking from a container.

What are the key steps to solving a related rates calculus problem about a water tank?

The key steps to solving a related rates calculus problem about a water tank are: 1) Identify the changing quantities involved; 2) Write an equation that relates these quantities; 3) Differentiate both sides of the equation with respect to time; 4) Substitute in the given values and solve for the desired rate of change.

What are some tips for solving related rates calculus problems about water tanks?

Some tips for solving related rates calculus problems about water tanks include: 1) Clearly labeling all given values and unknown quantities; 2) Drawing a diagram to visualize the problem; 3) Using the chain rule correctly; 4) Checking your answer for reasonableness; and 5) Practicing with different types of related rates problems to improve your problem-solving skills.

Similar threads

Related rates calculus problem about a water tank
Replies
1
Views
2K
B Pumping water upwards into a large water tank
Replies
3
Views
1K
Fluid dynamics problem: Equalize the volume of water in tanks at differing heights
2
Replies
50
Views
6K
MHB Need help on related rates problem
Replies
1
Views
1K
How Can Fault Detection Be Modeled in a Water Tank System?
Replies
7
Views
2K
Tank Mass Balance Calculation for Designing w/ Automatic Float Switch
Replies
1
Views
2K
Engineering Water Flow from Pressurized Tank through Heat Exchanger
2
Replies
56
Views
4K
MHB How Fast Does the Water Level Rise in a Conical Tank?
Replies
1
Views
1K
Filling a tank flow rate problem
Replies
2
Views
2K
MHB Derivation Application in Differential Calculus, verification question problem.
Replies
4
Views
2K

Hot Threads

Recent Insights

Back
Top