Relative intensities of fine-structure components in an alkali

[hicetnunc]

Homework Statement

An emission line in the spectrum of an alkali has three fine-structure components corresponding to the transitions $^2\text{P}_{3/2} - ^2\text{D}_{3/2}$, $^2\text{P}_{3/2} - ^2\text{D}_{5/2}$ and $^2\text{P}_{1/2} - ^2\text{D}_{3/2}$. These components have intensities $a$, $b$ and $c$, respectively, that are in the ratio $1:9:5$. Show that these satisfy the rule that the sum of the intensities of the transitions to, or from, a given level is proportional to its statistical weight ($2J+1$).

Relevant Equations

None.

Hi. I'm really stuck with this problem and would appreciate some help.

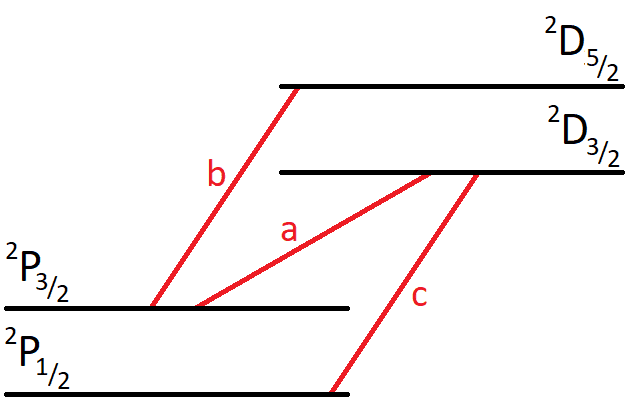
For example, if i take the total intensity from the $^2\text{P}_{3/2}$ level, i get $a+b$. Since $b$ is 9 times larger than $a$, i get that the total intensity is $10a$. This should then be proportional to the statistical weight $2J+1=4$, so $10a = 4q$, where $q$ is a proportionality constant. Then $q=2.5a$. But if I then consider the $^2\text{D}_{3/2}$ level, I get that its total intensity is $a+c=6a$ and has statistical weight 4. Then the proportionality constant would be $q=\frac{6a}{4}=1.5a$. This doesn't seem right since I get different proportionality constants.

How should I handle this problem?

 

[mjc123]

You can only compare the P levels with each other, or the D levels with each other, not P with D. This works if you consider that for the P levels, (a+b):c =10:5 = 4:2, while for the D levels b:(a+c) = 9:6 = 6:4. But the total number of states in the P and D levels are different (6 and 10 respectively), so the proportionality constants must be different.

However, you can do it if you normalise the statistical weights so that their sum for the P or D levels is 1. So the weight for ²P_1/2 = 2/(2+4) = 1/3, for ²P_3/2 is 2/3, ²D_3/2 2/5, and ²D_5/2 3/5. Then you get q = 15a for both P and D.

 

[hicetnunc]

Alright, that cleared it up! Thanks a lot!