Relativistic correction to Coulomb Potential in SQED

[Seriva]

Homework Statement

Obtain the relativictic lower order correction to the Coulombian potential

Relevant Equations

Those ones that are in the appended photo

I have derived the Coulombian potential as an effective potential between two spinless charged particle taking the non-relativitic approach on the scattering amplitude obtained in terms of the Feynman rules in SQED.
The scattering amplitudes are:

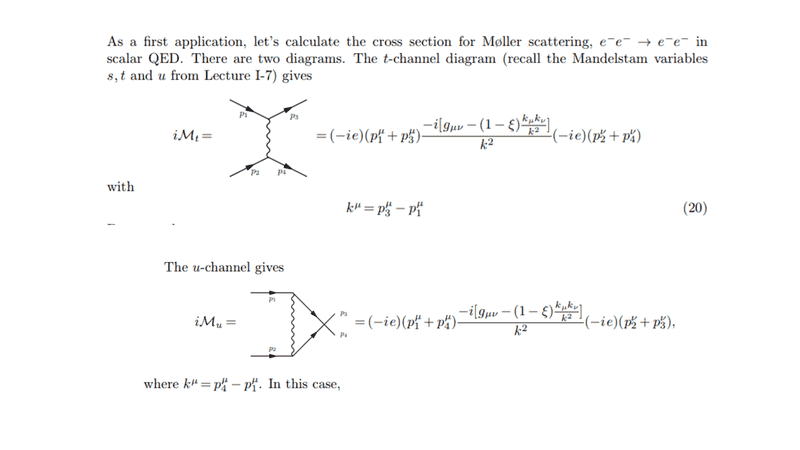
I'm using the gauge in which xi = 1.
How could I obtain the relativistic lower order correction of the Coulombian potential using those amplitudes??

 

[NateD]

In order to obtain the relativistic lower order correction of the Coulombian potential, one needs to use the scattering amplitudes and calculate the corrections due to the higher order terms that appear in the interaction due to relativistic effects. This can be done by computing the Feynman diagrams which contain these higher order terms. The diagrams with the leading order relativistic corrections will then provide the appropriate correction to the Coulombian potential.