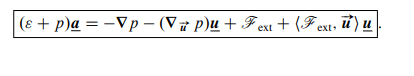Relativistic Hydrodynamics Eqn for Perfect Fluid: Insight Needed

[Bruno Cardin]

TL;DR Summary

I'm struggling with understanding the meaning of an extra "term" in the motion equations for a relativistic fluid.. wether it's in SR or GR. More info below.

Hello. Could anyone help me with some insight in an extra term appearing in the motion equations of a relativistic fluid? I say extra term, because it's not present on the motion for a test particle, as it follows:

Let's propose Minkowski space-time, the motion equations for a fluid with zero pressure, with four-velocity U, mass density ¶ and subject to an external force density f is:

¶ a= f + <f,U> U (with a being the four-acceleration). This is called the relativistic Euler equation and can be easely deduced from the energy momentum conservation of the tensor T generated by said force.

While the same equation, when written for a test particle submitted to the same force , would follows the usual equation m.a=F , written in four-vectors.

What is that extra term <f,U> U ?

 

[Orodruin]

It subtracts the heat-like force, which is proportional to the 4-momentum and therefore increases the energy density rather than changing the 4-velocity.

Also, please see the LaTeX guidelines for writing equations.

 

[vanhees71]

A treatment using the action principle can be found here:

https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf Sect. 3.5.3.

 

[Bruno Cardin]

It subtracts the heat-like force, which is proportional to the 4-momentum and therefore increases the energy density rather than changing the 4-velocity.

Also, please see the LaTeX guidelines for writing equations.

Thanks for the reply.

I kind of understand better now, but there's still something bugging me. So, basically, I'm working with charged dust, which is described by energy-momentum tensor:

$$ T^{\alpha \beta}= \rho U^{\alpha} U^{\beta} + E^{\alpha \beta}$$

where U is the four velocity, $\rho$ is the fluid's rest mass density and E is the electromagnetic field energy-momentum tensor. Applying the equation $T^{\alpha \beta};_{\beta} =0$ we reach the equation:

$$\rho U^{\beta}U^{\alpha};_{\beta}=-E^{\alpha \beta};_{\beta}- (here the already mentioned term)$$

$-E^{\alpha \beta};_{\beta}$ is by definition the Lorentz force, and the extra term is the product of the Lorentz force with the four velocity, times the four velocity. This equation has 4 components (1 for each $\alpha$), so basically that extra term is a vector.

I get from your answer that what it does is increase $\rho$ ? Sorry for bothering.

 

[vanhees71]

I don't know, what you mean by "extra term". In the dust model there are no thermal contributions (no heat) and the only stress is the Maxwell stress tensor of the em. field, which is included on the right-hand side.

What looks like four equations, are in fact only three, because indeed the right-hand side is
$$-{E^{\alpha \beta}}_{;\beta}=\rho_q F^{\alpha \beta} U_{\beta},$$
where $\rho_q$ is the charge density in the local rest frame of the fluid cell (a scalar quantity as is your mass density $\rho$). Since $U_{\alpha} U^{\alpha}=1$ you have $U_{\alpha} ({U^{\alpha}}_{\;\beta})=0$, and this is consistent with the right-hand side, because $F^{\alpha \beta}=-F^{\beta \alpha}$. So there are indeed only three independent equations of motion as it must be.

 

[Bruno Cardin]

I don't know, what you mean by "extra term". In the dust model there are no thermal contributions (no heat) and the only stress is the Maxwell stress tensor of the em. field, which is included on the right-hand side.

What looks like four equations, are in fact only three, because indeed the right-hand side is
$$-{E^{\alpha \beta}}_{;\beta}=\rho_q F^{\alpha \beta} U_{\beta},$$
where $\rho_q$ is the charge density in the local rest frame of the fluid cell (a scalar quantity as is your mass density $\rho$). Since $U_{\alpha} U^{\alpha}=1$ you have $U_{\alpha} ({U^{\alpha}}_{\;\beta})=0$, and this is consistent with the right-hand side, because $F^{\alpha \beta}=-F^{\beta \alpha}$. So there are indeed only three independent equations of motion as it must be.

Thanks for the reply. What I mean with "extra term" is that when you have a free particle (let's say a test particle of mass m) in the presence of an e.m field that generates a tensor E, then the equation of motion for said particle is $m a= F$ where a is it's four acceleration and F is the Lorentz Force, whereas for a fluid, the equation is:

$$\rho a= -E^{\alpha \beta}_{;\beta} - ( E^{\mu \beta}_{;\beta} U_{\mu}) U^{\alpha}$$

And given the fact that $-E^{\alpha \beta}_{;\beta}$ is defined as the Lorentz force density, $f^{\alpha}$, then we have:

$$\rho a= f^{\alpha} + (f^{\mu} U_{\mu}) U^{\alpha}$$

which is the analogue to the point-like particle, but has another force. The test particle is only submitted to the Lorentz force F, where the fluid is submitted to the Lorentz force density f and the dot product between said density and the four velocity, which I called "extra term", $(f^{\mu} U_{\mu}) U^{\alpha}$. I don't know what that term does or what does it mean.

 

[vanhees71]

Where do you get this equation from? I simply get (for SRT in standard Minkowski coordinates; for GR, just put covariant derivatives instead of partial derivatives)
$$\rho_m u^{\mu} \partial_{\mu} u^{\nu}=\rho_q F^{\nu \mu} u_{\mu},$$
as it should be.

For a single particle the equation of course reads
$$m \mathrm{d}_{\tau} u^{\nu} = q F^{\nu \mu} u_{\mu},$$
where
$$u^{\mu}=\mathrm{d}_{\tau} x^{\mu},$$
with $\tau$ the proper time of the particle.

In the continuum-mechanical fluid equation of course $\mathrm{D}_{\tau}=u^{\mu} \partial_{\mu}$ is the "material proper-time dervivative".

For more on the model of a charged-dust fluid, see

https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf (Sect. 4.4).

 

[Bruno Cardin]

Where do you get this equation from? I simply get (for SRT in standard Minkowski coordinates; for GR, just put covariant derivatives instead of partial derivatives)
$$\rho_m u^{\mu} \partial_{\mu} u^{\nu}=\rho_q F^{\nu \mu} u_{\mu},$$
as it should be.

For a single particle the equation of course reads
$$m \mathrm{d}_{\tau} u^{\nu} = q F^{\nu \mu} u_{\mu},$$
where
$$u^{\mu}=\mathrm{d}_{\tau} x^{\mu},$$
with $\tau$ the proper time of the particle.

In the continuum-mechanical fluid equation of course $\mathrm{D}_{\tau}=u^{\mu} \partial_{\mu}$ is the "material proper-time dervivative".

For more on the model of a charged-dust fluid, see

https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf (Sect. 4.4).

This is how I get that equation, in GR with covariant derivatives (but it's the same in Minkowski space-time):

We have charged dust, so the system is described by the energy-momentum tensor:

$$ T^{\alpha \beta} = \rho U^{\alpha} U^{\beta} + E^{\alpha \beta} $$

With E being the e.m energy-momentum tensor. Let's clarify now that $-E^{\alpha \beta};_{\beta}$ is by definition the Lorentz force density, $f^{\alpha}= \sigma F^{\alpha}_{\beta} U^{\beta}$ with $\sigma$ being the charge density.

This system obbeys the laws of conservation $T^{\alpha \beta} ;_{\beta}=0$, so if we write that, we get:

$$
0=\rho;_{\beta}U^{\alpha}U^{\beta}+\rho U^{\alpha};_{\beta}U^{\beta} +\rho U^{\alpha} U^{\beta};_{\beta} + E^{\alpha \beta};_{\beta}$$

And using the identity for the Lorentz force density, we get:

$$0= \rho;_{\beta}U^{\alpha}U^{\beta}+\rho U^{\alpha};_{\beta}U^{\beta} +\rho U^{\alpha} U^{\beta};_{\beta} - f^{\alpha}$$

This is a coordinate equation (one for each $\alpha$ , so we can write it as a full vector equation as:

$$\overline{0}=\rho;_{\beta}U^{\beta} \overline{U}+\rho U^{\beta};_{\beta} \overline{U} + \rho \overline{a} - \overline{f} $$

where $\overline{a}$ is the four-acceleration of the fluid (We have replaced it for the expression in coordinates $U^{\beta}U^{\alpha};_{\beta}$).

Now we can contract the equation with the operator $P=I+ \overline{U}\otimes \widetilde{U}$ which is still equal to the null vector $\overline{0}$ on the left, and on the right we get the expression usint the fact that $P( \overline{U})=\overline{0}$ and $P( \overline{a})= \overline{a}$ :

$$\overline{0}= \rho \overline{a}-P(\overline{f})$$

So:

$$ \rho \overline{a} = P(\overline{f})$$

Which, back to coordinate notation, translates to the relativistic Euler equation for a fluid:

$$ \rho U^{\beta}U^{\alpha};_{\beta}= P(\overline{f}) ^{\alpha} $$

Now, $P(\overline{f})= \overline{f}+ <\overline{f},\overline{U}> \overline{U}$

So, finally:

$$ \rho U^{\beta}U^{\alpha};_{\beta}=f ^{\alpha} + <\overline{f},\overline{U}>U^{\alpha} $$

That's where the "extra" term comes from.

 

[vanhees71]

You forgot the continuity equation, i.e.,
$$(\rho \U^{\mu})_{;\mu}=0,$$
i.e.,
$$(\rho U^{\alpha} U^{\beta})_{;\alpha} = (\rho U^{\alpha})_{;\alpha} + U^{\alpha} \rho {U^{\beta}}_{;\alpha}.$$
Plugging this into the energy balance equation, you get
$$U^{\alpha} \rho {U^{\beta}}_{;\alpha}=-{E^{\alpha \beta}}_{\;\beta} = f^{\beta} = \sigma F^{\beta \alpha} U_{\alpha}.$$
I still don't see, where your extra term should come from, and it's also not what you expect from equation of motion for point particles.

 

[Bruno Cardin]

You forgot the continuity equation, i.e.,
$$(\rho \U^{\mu})_{;\mu}=0,$$
i.e.,
$$(\rho U^{\alpha} U^{\beta})_{;\alpha} = (\rho U^{\alpha})_{;\alpha} + U^{\alpha} \rho {U^{\beta}}_{;\alpha}.$$
Plugging this into the energy balance equation, you get
$$U^{\alpha} \rho {U^{\beta}}_{;\alpha}=-{E^{\alpha \beta}}_{\;\beta} = f^{\beta} = \sigma F^{\beta \alpha} U_{\alpha}.$$
I still don't see, where your extra term should come from, and it's also not what you expect from equation of motion for point particles.

Actually, the first guy was right (look at the first reply). It is a heat-like force.

$(\rho \U^{\mu})_{;\mu}=0,$ is not neccesarely true, if there is a change in the internal energy of the fluid (not mass creation, but internal energy, so that $\rho=\rho_{0} + \epsilon$ .

Look again at this equation coming from the energy conservation:

$$0=(\rho U^{\beta});_{\beta}U^{\alpha} + \rho U^{\beta}U^{\alpha};_{\beta} - f^{\alpha}$$

(where this time I didn't separate the first term in two). Now let's contract it with the four-velocity one-form (U_{\alpha}) , then the term with the acceleration vanishes, and we have a -1 with the first term:

$$0= - (\rho U^{\beta});_{\beta} - (f^{\alpha}U_{\alpha})$$

So $(f^{\alpha}U_{\alpha})= - (\rho U^{\beta});_{\beta}$

This is because the four- acceleration always sattisfies <a,U>=0, so if the force as a component tangent to the four-velocity, then it can't produce acceleration.. it changes the internal energy instead (and its proportional to the four-momentum, like the first guy said).

Plugging that back to the last equation on my previous post, we get:

$$\rho U^{\beta}U^{\alpha};_{\beta} + (\rho U^{\beta});_{\beta} = f^{\alpha}$$

The part of the force tangent to the four-velocity produces changes in the internal energy of the fluid, while the other part produces acceleration.

For the Lorentz force, however, $(f^{\alpha}U_{\alpha})$ is always zero (just read it in my E.M notes), if U is the four-velocity of the fluid that generates the field of course. So for this particular case, it vanishes, but it is a regular term in other cases (check equation 5.15 of the attached file and follow the analogue with an extra force instead of the pressure P. This is also done in the book "Gourgoulhon special relativity" , equation 21.49, which I couldn't attach because it was too big, but I'll leave the screenshot).

Anyways, thanks for the help.

 

[vanhees71]

Yes, but you discussed the dust model, which by assumption doesn't have taken into account interactions between the particles making up the fluid. For that you need another model. The most simple is the perfect fluid, where
$$T^{\mu \nu} = (u+P) u^{\mu \nu} -P g^{\mu \nu},$$
where $u$ is the density of the inner energy (in the local fluid rest frame) and $P$ the pressure. Then of course you get additional terms in the equations of motion describing the forces on the fluid element due to the pressure due to the fluid around it, as it should be.

You find this also in my writeup on SR (Sect. 3.5.3)

https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf

For the GR case, see

L. Rezzolla and O. Zanotti, Relativistic hydrodynamics,
Oxford University Press, Oxford (2013),
https://dx.doi.org/10.1093/acprof:oso/9780198528906.001.0001