B Relativity of Measures: A vs B Frame & Light Source S

[AlMetis]

A frame “A” of three perpendicular axis (x, y, z) and an identical frame “B”, with an observer at rest in each, are moving toward each other at 1/2c on their common x axis.
Centered between A and B on x is a light source S.
At the time t1 when A, B and S coincide on x, S emits a light flash.
Given the symmetry of motion between A, B and S, are the paths observed by A and B a mirror of each other?
If not, please explain.

 

[Ibix]

A frame “A” of three perpendicular axis (x, y, z) and an identical frame “B”, with an observer at rest in each,

That's not really what's meant by a frame, but that doesn't really matter here.

moving toward each other at 1/2c on their common x axis.

This is more problematic. Your diagram implies both are doing 0.25c in the frame where their speeds are equal and opposite, but this does not mean either is doing 0.5c with respect to any object in your scenario.

Given the symmetry of motion between A, B and S, are the paths observed by A and B a mirror of each other?

What do you mean by "paths"? Paths of what?

And since this looks like homework, why don't you give your answer and justification first?

 

[FactChecker]

The answer depends on what you assume and what you want to conclude. You say nothing about whether these are inertial reference frames or not. For all we know, the entire set-up might be whizzing around, accelerating, and spinning. Mathematical reference frames can do that.
I assume that you meant them to be inertial reference frames. Then yes, there is symmetry. Otherwise, there is not necessarily physical symmetry. With acceleration, the behavior of the light could be different (not symmetric) for observers A and B.

 

[AlMetis]

I think this means the speed between A and B is 0.5c, because they are both moving toward S with speed 0.25c

I think this is true. A photon going straight up from S would appear to angle toward B and vise a versa because A and B are going in opposite directions.

 

[Ibix]

I think this means the speed between A and B is 0.5c, because they are both moving toward S with speed 0.25c

Then you need to look up relativistic velocity addition. Each one sees the other doing 8c/17, or about 0.47c.

I think this is true. A photon going straight up from S would appear to angle toward B and vise a versa because A and B are going in opposite directions.

Which observer would see this?

Also, a word to the wise, don't talk about photons in relativity. Talk about light pulses. Photons are much more complicated things and it's good not to refer to classical light pulses as photons. Then you will end up less confused when you study actual photons in quantum field theory.

 

[AlMetis]

Is it more accurate to say a light pulse perpendicular to x as seen from S, would appear at an angle toward B when seen from A and the same angle toward A when seen from B?

 

[AlMetis]

FactChecker, yes these are inertial frames of reference.

 

[Ibix]

Assuming A and B have passed the source and are moving away from it, yes.

 

[AlMetis]

From the inertial frame “E”, also on the common x axis with A, B and S, an observer measures the motion of S at 0.125 c along x toward B, the motion of A at 0.375c along x toward B, and the motion of B at 0.125 c along x toward S.
At the time t1 A, B, S and E coincide on x.
E remains at the center of the light pulse while A, B and S continue in their motion relative to E.
Given the asymmetry of the motion between A, B and S relative to E, at t1, would a light pulse viewed from E moving perpendicular to x, appear at an angled toward B when seen from A and the same angle toward A when seen from B?
If so, please explain.

 

[Nugatory]

If so, please explain.

Assuming that all the speeds are stated relative to the origin of the same inertial frame (so no need to mess with relativistic velocity addition)….
Assuming that we’re considering the light source is something like a laser, sending a flash out on a straight-line path (as opposed to the expanding sphere of light we’d get by switching on a light bulb)….
Assuming that in that frame the laser was aimed straight up, perpendicular to the x-axis….

A will find that the path of the laser light is not perpendicular to the x-axis. Instead it is at some non-zero angle $\theta$ from the perpendicular. (I’m not going to bother calculating it because the exact numerical value is uninteresting, the point is that it is non-zero).
B will find that the path of the laser light is not perpendicular to the x-axis. It will be at an angle $-\theta$ to the perpendicular. This is clear from the symmetry of the setup and is just a repeat of the discussion up through post #8.
E will find that the path of the laser light is straight up, perpendicular to the x-axis. That’s part of the specification of the problem, it’s the third assumption above.

All three agree about where the light beam is actually going. The difference in their descriptions of the path is because they have different notions of ”perpendicular”. It’s similar to the trajectory of a bouncing ball on the deck of a ship - vertical relative to the deck if we’re on the ship, angled relative to the deck if we’re on the shore watching the ship moving past us.

Your setup you is one mirror away from being a light clock that is at rest relative to E, so you may want to Google for “light clock” and look at some of our older threads on the subject.

 

[AlMetis]

A light clock does make this a clearer picture, thank you.

It appears in the first example A and B would see what you said $\theta$ and $-\theta$ which I assume is a mirror on x across y.
But the second example puts A further along +x than B is along -x.
Why doesn’t this mean A sees a different angle that what B sees?

 

[Nugatory]

But the second example puts A further along +x than B is along -x.
Why doesn’t this mean A sees a different angle that what B sees?

I'm sorry, I wasn't looking carefully and didn't notice that you had selected different speeds in the second example.

 

[Ibix]

From the inertial frame “E”, also on the common x axis with A, B and S, an observer measures the motion of S at 0.125 c along x toward B, the motion of A at 0.375c along x toward B, and the motion of B at 0.125 c along x toward S.

These numbers are inconsistent with your initial setup. You appear to have used Galilean velocity transformation to E's rest frame, not relativistic.

Assuming that the intent is that E and S measure each other to be doing $c/8$ then E would measure A to be doing $4c/11$ and B to be doing $4c/31$. On the other hand, if the intent is that E measure S and B to be doing the same speed then it would need to be doing $(4-\sqrt{15})c$ relative to S and would measure A to be doing $(4\sqrt{15}-17)c/(\sqrt{15}-8)$.

 

[AlMetis]

The second example is not a relativistic transformation of the first, each is the same motion defined in Galilean measures relative to S in the first example and relative to E in the second example.Whether the speeds are Galilean, or relativistic measures (because the observations of A and B are either symmetric, or not), the light pulse observed from A and B are different in each example?

 

[Ibix]

The second example is not a relativistic transformation of the first, each is the same motion defined in Galilean measures relative to S in the first example and relative to E in the second example.

Ok. Probably worth stating when you are starting a new example, especially if you reuse numbers and labels. It seems to have caught out both Nugatory and me for different reasons.

Whether the speeds are Galilean, or relativistic measures (because the observations of A and B are either symmetric, or not), the light pulse observed from A and B are different in each example?

They would be symmetric in the first (A, B, S) example and not in the second (A, B, S, E).

Where are you going with all this? It's fairly straightforward to do the Lorentz transforms and determine all of this.

 

[AlMetis]

Sorry for the confusion.

I’m asking why they are different.

How is the symmetry of the observations of a light pulse by two inertial frames (A and B) broken simply by the knowledge of the motion relative to another inertial frame E?

 

[Ibix]

How is the symmetry of the observations of a light pulse by two inertial frames (A and B) broken simply by the knowledge of the motion relative to another inertial frame E?

It isn't. In one case you emitted a light pulse perpendicular to the x axis as seen by S, who sees A and B moving with equal speed. In the second case it was emitted perpendicular to the x axis as seen by E, who sees A moving faster than B. So this version of the scenario does not have mirror symmetry in any frame. The first one does. You broke the symmetry by specifying an asymmetric scenario.

 

[AlMetis]

Yes I did, I am confusing the angle of emission with the path observerd.

In each example the light pulse is emitted perpendicular to x, which is to say the direction, or pointing of the source is perpendicular to x and x is common to all observers. The angle of the path traced by the light pulse between t1 and t2 is determined by the motion of the observer relative to the pulse.

I think what is confusing is that the first example does not give enough information to know the path observed by A and B. The second example shows A, B and S are moving, but without the inertial frame E, we don’t know they are.

 

[PeroK]

I think what is confusing is that the first example does not give enough information to know the path observed by A and B. The second example shows A, B and S are moving, but without the inertial frame E, we don’t know they are.

None of this makes any sense to me, I'm sorry to say. I'm struggling to see the purpose of this thread?

 

[Ibix]

I think what is confusing is that the first example does not give enough information to know the path observed by A and B.

Yes it does, at least as modified by #6 where you clarified that the light pulse is emitted perpendicular to the frame velocities as seen by S.

The second example shows A, B and S are moving, but without the inertial frame E, we don’t know they are.

No, you can always pick one frame and choose to call it a rest frame. You chose E there, but you could have chosen any of the other three, or some other frame that doesn't have a physical manifestation in your scenario. The difference between your scenarios is entirely due to your differing choices of setup; in both scenarios at most one of the frames can be chosen to be at rest and all the others are moving. No analysis can possibly show otherwise.

 

[AlMetis]

I understand that the position of rest is relative, but the choice of an observer at one location should not change what is observed by another observer at another location.

I will use your suggestion of a light clock.
When the emitter at the bottom of the clock is pointing at the mirror at the top of the clock, the light pulse will NOT strike the mirror if the mirror moves after emission. We are talking about a pulse that travels in a straight line in the direction the emitter is pointed.
Whether the pulse strikes the mirror or not is not something determined by an observer’s choice of reference frame, even though whether the mirror moves or not is “exclusively” determined by the observer’s choice of reference frame. So an observer can choose a frame that explains the empirical evidence, and they can choose a frame that contradicts the empirical evidence.
We could say the light pulse scenario is just a tool for discussion and the reality is light is a wave so the light will strike the mirror even when the mirror moves after emission. That makes more sense, but the light pulse example makes it clear that even using the wave model we have to admit the distance from emitter to mirror is greater when the mirror moves after emission.

 

[Sagittarius A-Star]

I will use your suggestion of a light clock.
When the emitter at the bottom of the clock is pointing at the mirror at the top of the clock, the light pulse will NOT strike the mirror if the mirror moves after emission.

That's wrong. If the angle of the light pulse trajectory with the x-axis is 90 degree in the rest frame of the light-clock, then it is not 90 degree in the other frame of reference. This difference of the angle in two frames is called "aberration".

 

[Ibix]

the choice of an observer at one location should not change what is observed by another observer at another location.

Indeed. Nobody has said otherwise and a physical model that predicted that would be rapidly discarded.

When the emitter at the bottom of the clock is pointing at the mirror at the top of the clock, the light pulse will NOT strike the mirror if the mirror moves after emission.

If you mean a light clock that accelerates perpendicular to its length then I agree, and all observers will agree. If you mean a light clock viewed from a frame where it is in steady motion then no, the light pulse will always be found on a line between emitter and mirror and all frames will agree on this.

We are talking about a pulse that travels in a straight line in the direction the emitter is pointed.

No - as @Sagittarius A-Star comments, you appear not to understand aberration. It's extremely common for motions that are perpendicular in one frame not to be perpendicular in others. The most prosaic is rain on a car window. The car moves horizontally and the rain falls vertically, yet the rain makes slanted drops on the side windows of the car. This shows that, in the rest frame of the car, the rain isnot falling vertically.

Whether the pulse strikes the mirror or not is not something determined by an observer’s choice of reference frame,

True.

So an observer [...] can choose a frame that contradicts the empirical evidence.

No they cannot.

We could say the light pulse scenario is just a tool for discussion and the reality is light is a wave so the light will strike the mirror even when the mirror moves after emission. That makes more sense, but the light pulse example makes it clear that even using the wave model we have to admit the distance from emitter to mirror is greater when the mirror moves after emission.

This seems to be you looking for a way out of a trap you built for yourself by not following the point about aberration.

 

[PeroK]

When the emitter at the bottom of the clock is pointing at the mirror at the top of the clock, the light pulse will NOT strike the mirror if the mirror moves after emission. We are talking about a pulse that travels in a straight line in the direction the emitter is pointed.

If that were true, it would apply to all objects, not just light ways. E.g. imagine you are in a moving car and you throw an object out of the side window in a straight line perpendicular to the side of the car (from your reference frame). To an observer on the ground, the object moves at an angle relative to the side of the car.

There is no contradiction here. Instead, it's your misconception that somehow the relative direction of motion must be the same in both frames (which is physically impossible).

There seem to be many students who make this mistake. It may boil down to it being difficult to imagine the same scenario in two different reference frames.

In the above example, imagine you fire the object using a length of tube pointing out the car. To an observer on the ground, the object will still move at an angle (because the tube is moving). In fact, you can consider the motion of the object as it moves down the tube. To the observer in the car, it is moving perpendicular to the side of ther car. But, to the observer on the ground, it is moving at an angle. To maintain your assertion, then the object must break out of the tube in the ground frame to move perpendicular to the car - which is physically impossible.

 

[Ibix]

It may boil down to it being difficult to imagine the same scenario in two different reference frame.

I think it comes down to this: if I hold my arm out to the side and walk forward my arm is perpendicular to my direction of motion and/or the direction of motion of the floor in all frames. But if an ant walks down my arm, it is not moving perpendicular to my/the floor's motion in most frames, despite walking on my perpendicular arm.

That may seem paradoxical, but if so then you (general you, not @PeroK) are mixing up your frames of reference and forgetting to keep track of what is moving in which frame.

 

[PeroK]

I think it comes down to this: if I hold my arm out to the side and walk forward my arm is perpendicular to my direction of motion and/or the direction of motion of the floor in all frames. But if an ant walks down my arm, it is not moving perpendicular to my/the floor's motion in most frames, despite walking on my perpendicular arm.

That may seem paradoxical, but if so then you (general you, not @PeroK) are mixing up your frames of reference and forgetting to keep track of what is moving in which frame.

That's the perfect example!

 

[DrGreg]

My 2015 animation to illustrate aberration:

(from https://www.physicsforums.com/threads/how-does-light-slide-sideways.804112/post-5048516)

 

[vanhees71]

That's wrong. If the angle of the light pulse trajectory with the x-axis is 90 degree in the rest frame of the light-clock, then it is not 90 degree in the other frame of reference. This difference of the angle in two frames is called "aberration".

Aberration is explained in Sect. 4.3 on the Doppler effect of light in

https://itp.uni-frankfurt.de/~hees/pf-faq/srt.pdf

 

[AlMetis]

@PeroK, “Objects” acquire momentum from their source (cars), light does not.
As DrGreg’s animation illustrates, the equilateral triangle (source - mirror- source) is the path of the light pulse. It is not what is measured by an observer in the train(aberration), but it is the physical path the pulse must take to meet the mirror when the mirror is no longer “physically” where it was at the time of emission.

If that was not the case there would be no need to reconcile two “different” lengths measured by an observer on the platform with an observer in the train (and at very hight speeds invoke the Lorentz transformation). We would simply say, one of the observers is wrong.

When there is an identical source on the platform that emits the pulse when the source on the train aligns with it on the track (x axis), the observations/measurements would not change for either observer from those they made when the pulse was emitted from the source on the train. For all intents and purposes the observer on the train would not have any way to measure, or reason to even suspect the pulse was not emitted from the source on the train.
This demonstrates the position of the source at emission relative to the position of the mirror at reflection is what determines the length the light pulse travels from source to reflection. This length is a “measurement” not common to both observers, but it is common and a LONGER measure for all observers at rest with the source (platform) and it is common and a SHORTER measure for all observers at rest with the train.

 

[PeroK]

@PeroK, “Objects” acquire momentum from their source (cars), light does not.

Yes, it does. It must, otherwise (as you have discovered), the motion of light is physically completely impossible. Imagine light moving down the centre of a hollow tube (in vacuum). It must move down the centre of that hollow tube in all frames (just like any object). It can't move inside the tube in one frame and outside the tube in another frame where the tube is moving.

Like I said, a lot of students share your misconception. The simple fact is that light cannot possibly behave the way you demand, so you must have misunderstood something!

 

[PeroK]

PS it's the speed of light that's invariant. Not its momentum vector.

 

[Ibix]

“Objects” acquire momentum from their source (cars), light does not.

Yes it does. You may be mistaking the (true) statement that the speed of light is independent of its source for the (false) one that the velocity of light is independent of its source. As PeroK has already pointed out, your (mis)conception of how light behaves is internally inconsistent, which is why you are struggling with it.

 

[robphy]

PS it's the speed of light that's invariant. Not its momentum vector.

Indeed, blue light has larger components for its 4-momentum vector compared to red light. But both blue light and red light travel at the same speed.

 

[Sagittarius A-Star]

Whether the pulse strikes the mirror or not is not something determined by an observer’s choice of reference frame

Exactly! Therefore, if you agree, that the light-pulse strikes the mirror in the rest-frame of the light-clock, then you must also agree, that the light-pulse strikes the mirror in the other reference-frame.

 

[Ibix]

In fact, why don't we do some maths? Let's say an object at rest at the origin emits a pulse of light in the $+y$ direction at $t=0$. The coordinates of the light pulse at time $t$ are therefore $x=0, y=ct$. Plug in the Lorentz transforms:$$\begin{eqnarray*}
t'&=&\gamma(t-vx/c^2)\\
&=&\gamma t\\
x'&=&\gamma(x-vt)\\
&=&-\gamma vt\\
&=&-vt'\\
y'&=&y\\
&=&ct\\
&=&ct'/\gamma
\end{eqnarray*}$$So we can see that relativity says that the light pulse's coordinates in a frame where the source is moving are $x'=-vt$ and $y'=ct'/\gamma$. The speed is still $c$: $x'^2+y'^2=(v^2+c^2/\gamma^2)t'^2=c^2t'^2$ so the pulse is distance $ct'$ from the origin at time $t'$. But its velocity has acquired an $x$ component so is not perpendicular to the $x$ axis in this frame.

These are two different descriptions of the same thing.

 

[AlMetis]

Exactly! Therefore, if you agree, that the light-pulse strikes the mirror in the rest-frame of the light-clock, then you must also agree, that the light-pulse strikes the mirror in the other reference-frame.

Yes I do agree. I never said otherwise.

The point of that example was that the choice of frame determines whether the mirror moves or not. That a moving target is struck by the same light pulse that strikes the non-moving target suggest there is a distinction in relative measures that is irrelevant to the physical events.

 

[AlMetis]

This length is a “measurement” not common to both observers, but it is common and a LONGER measure for all observers at rest with the source (platform) and it is common and a SHORTER measure for all observers at rest with the train.

This does not mean the observer on the train is wrong, or the observer on the platform is wrong. It it does mean time is running slower for one of these two observers.

Can anyone explain which it is?

 

[Nugatory]

This does not mean the observer on the train is wrong, or the observer on the platform is wrong. It it does mean time is running slower for one of these two observers.

Can anyone explain which it is?

In the case of uniform inertial movement (which is what we have here, as opposed to the completely different and unrelated twin paradox situations) both observers find that time is running slower for the other observer, both are correct, the situation is completely symmetrical, and there is no meaningful way in which we can say that time is running slower for one or the other.

 

[Sagittarius A-Star]

Yes I do agree. I never said otherwise.

You did say otherwise:

When the emitter at the bottom of the clock is pointing at the mirror at the top of the clock, the light pulse will NOT strike the mirror if the mirror moves after emission. We are talking about a pulse that travels in a straight line in the direction the emitter is pointed.
...
We could say the light pulse scenario is just a tool for discussion and the reality is light is a wave so the light will strike the mirror even when the mirror moves after emission.

SR can treat the particle model and wave model of light equally good.

 

[AlMetis]

the situation is completely symmetrical, and there is no meaningful way in which we can say that time is running slower for one or the other.

Is the symmetry broken if we know which measures a shorter light path?

 

[AlMetis]

You did say otherwise:

You’re taking what I said out of context.
If the light pulse does not hit the mirror, it will not be seen to hit the mirror in any frame of reference.
If it does hit the mirror, it will be seen to hit it in every frame of reference.
It will not hit the mirror if the mirror moves, but whether the mirror moves or not depends on the frame of reference chosen. This is still my original question now posed in three different scenarios.

 

[Ibix]

It will not hit the mirror if the mirror moves, but whether the mirror moves or not depends on the frame of reference chosen. This is still my original question now posed in three different scenarios.

This has been answered several times. If the pulse hits the mirror in the mirror rest frame then it will hit in the frame where the mirror is moving. Your argument that the pulse will not hit in a frame where the mirror is moving is not correct.

 

[Sagittarius A-Star]

If the light pulse does not hit the mirror, it will not be seen to hit the mirror in any frame of reference.
If it does hit the mirror, it will be seen to hit it in every frame of reference.

... is correct and contradicts to:

It will not hit the mirror if the mirror moves, but whether the mirror moves or not depends on the frame of reference chosen.

... which is wrong. See:
https://www.einstein-online.info/en/spotlight/light-clocks-time-dilation/

 

[Nugatory]

Is the symmetry broken if we know which measures a shorter light path?

No.
An easy way of seeing this is to provide two light clocks, one at rest relative to each observer. Both observers will consider the path of the light beam in the clock that is at rest relative to them to be "shorter" and the path of the light beam for the other clock to be "longer". Both will consider the path of the light in the clock that is at rest relative to them to be perpendicular to the x-axis and the path of the light in the other clock to be angled relative to the x-axis. Anything either says about one light clock, the other will be able to say about the other light clock, so there is no violation of symmetry anywhere.

It is worth noting that they both agree about the paths through spacetime that both light beams follow - that is, they agree about which points in spacetime the light passes through. They also agree about the lengths of these paths in spacetime. They disagree about what "perpendicular to the x-axis" means, because that notion is inherently frame-dependent.

 

[Sagittarius A-Star]

@PeroK, “Objects” acquire momentum from their source (cars), light does not.

Do you agree, that the light-pulse has in the (primed) rest-frame of the light-clock the following momentum in y-direction?
$p'_y = \frac{E'}{c^2}u'_y = \frac{E'}{c^2} c = \frac{hf'}{c}$

 

[vanhees71]

You did say otherwise:

SR can treat the particle model and wave model of light equally good.

At this level of discussion, please forget anything you think to know about "photons". I don't understand, why we still teach the totally wrong picture of Einstein's conception of 1905. Note that Einstein himself admitted as late as 1954 that he doesn't consider to have understood himself the "nature of radiation". What's for sure is that the naive photon picture of 1905 is completely wrong. Light (i.e., electromagnetic waves) have in no sense the properties of a localizable particle. According to modern QED, and that's what's indeed describet the "nature of radiation", i.e., the electromagnetic interaction, correctly, photons do not even have a proper position observable.

You should think about photons as being quanta of the free electromagnetic field, and their behavior is thus best "visualized" as the minimal portion of electromagnetic field energy and momentum of a given frequency and wave vector that can be emitted and absorbed by matter. In this sense Planck's photon picture was closer to modern QED than Einstein's.

 

[Sagittarius A-Star]

What's for sure is that the naive photon picture of 1905 is completely wrong.

Then you must also admit, that the classical wave picture is completely wrong. It predicts i.e. wrongly the pattern from a double-slit experiment with only a few photons.

But this discussion is not about the double-slit experiment. The relativistic Doppler/aberration formula can be derived correctly with both, the classical particle model and classical wave model of light. It should be possible to discuss SR, a classical theory, without invoking QED.

 

[vanhees71]

Well, that's why we have to quantize the electromagnetic field, i.e., there are phenomena which cannot be described by classical Maxwell theory.

As I said, for the Doppler effect you don't need photons. So stick to classical electrodynamics. It's a pretty simple calculation with a Lorentz transformation of the wave-four-vector.

 

[AlMetis]

This has been answered several times. If the pulse hits the mirror in the mirror rest frame then it will hit in the frame where the mirror is moving. Your argument that the pulse will not hit in a frame where the mirror is moving is not correct.

It has been stated a number of times and the math that repeats those statements has been well documented. But neither explain why.As I have said before, if the pulse is seen from one frame to hit the mirror, it will be seem from all frames to hit the mirror, if it misses in one frame it will miss in all frames.
That is not a guess, or a hypothesis, or a wish. It is absolutely clear, empirical fact.
I am not questioning that. I am questioning why that is contradicted in the examples I gave.
The responses have all been something similar to:
“it is not contradicted in your examples if you interpret your examples as we say you should.”
I can accept that if you just explain “why”. Telling me what to “believe” is not teaching me science.Here is another example that I think is detailed enough that any mistake/s I have made should be ealily explained.
When I am next to and at rest with the sun, I am at rest with where the light leaves the sun at any instant. A minute later I can still be at rest with the sun, but if I am, I am moving roughly 828,000 km/hr away from where the light left the sun a minute ago.
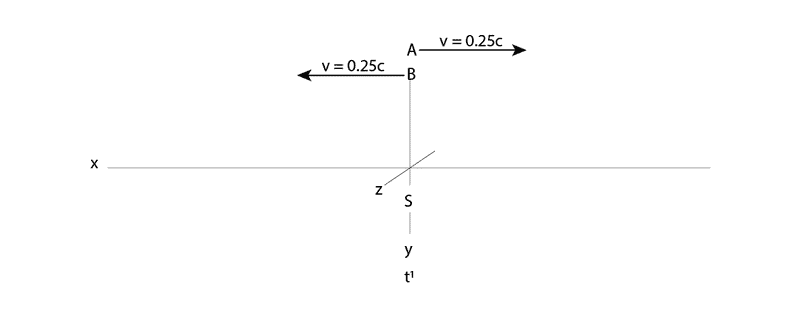
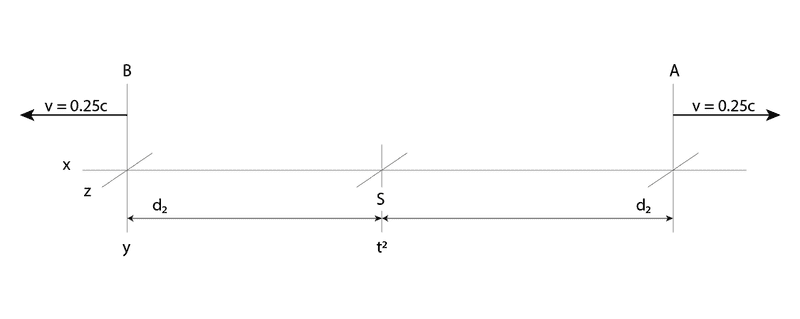
If I don’t know, or have no way to measure this motion of the sun/me relative to where the light was emitted from it a minute ago, that does not change the path of that light relative to any frame of reference. If that light hits the earth, every frame will see it hit the earth. The motion that determines whether that light hits the earth is not the motion of any frame relative to the earth, or sun. It is the motion of the earth relative to where the light was emitted. The earth is either on a collision path with the light, or not. The light does not change its direction to chase the earth. When A and B see S remain centered between them, you and I will predict the path of the light pulse emitted from S will be symmetric as observed by A and B. When E sees A, B and S moving along a common axis (x) they will all be moving relative to where the light is emitted from S at any instant. Unlike me at rest with the sun, E remains where the light was emitted. With this “new” information, you and I will claim the path observed by A and B will NOT be symmetrical.

Why does what E measures change what you and I predict will be seen by A and B if the relative motion of A, B and S is all that determines the path of the light observed by A and B and that relative motion does not change?

 

[Ibix]

But neither explain why.

PeroK pointed out the reason on the last page, but you replied:

“Objects” acquire momentum from their source (cars), light does not.

The problem with your reasoning is thinking that light does not acquire momentum from its source. If you accept that it does then all your problems go away (and you don't violate momentum conservation). The self-contradictory mess you are in trying to think otherwise should tell you that you should be considering what we've said.

If conservation of momentum is not enough of an explanation for you, what would be?