- #1
Oz123
- 29
- 0
Ok, so here's an example from David Morin's book that I seriously don't understand:
Two clocks are positioned at the ends of a train of length L (as measured in its own frame). They are synchronized in the train frame. The train travels past you at speed v. It turns out that if you observe the clocks at simultaneous times in your frame, you will see the rear clock showing a higher reading than the front clock (see attached image). By how much?
Ok, so his solution is quite vague, he says that the time delay for the light going to the left relative to the light going to the right with respect to the train's frame is lv/c^2, that is clear. But why is it equal to the time delay of the clocks with respect to the outside observer?
Why can't it just be like this:
Supposed we do place the light in the middle of the train, so that the time it takes to travel for the left and right lights be t1=l/2(c+v) and t2=l/2(c-v) respectively. Now we suppose that the clock hands will move if the light reaches at each end, so in the train's frame, both light reaches at the same time, therefore the hands will tick at the same time. And for the outside observer, there will be a time difference of t1-t2, so that's the same time difference between the movements of the hands. So we get t1-t2=vl/(c^2-v^2) which is not the same as lv/c^2, since it has an extra factor of the square of the Lorentz factor...Why is this other thought experiment wrong (well, it gives a different answer) when it seems to be right?
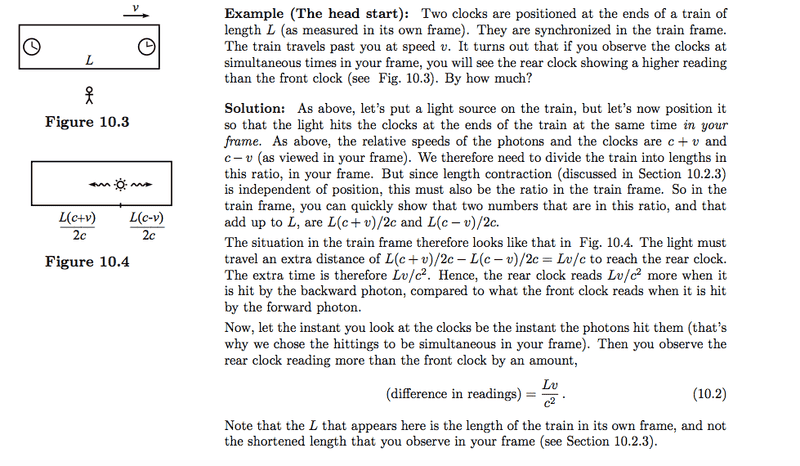
Two clocks are positioned at the ends of a train of length L (as measured in its own frame). They are synchronized in the train frame. The train travels past you at speed v. It turns out that if you observe the clocks at simultaneous times in your frame, you will see the rear clock showing a higher reading than the front clock (see attached image). By how much?
Ok, so his solution is quite vague, he says that the time delay for the light going to the left relative to the light going to the right with respect to the train's frame is lv/c^2, that is clear. But why is it equal to the time delay of the clocks with respect to the outside observer?
Why can't it just be like this:
Supposed we do place the light in the middle of the train, so that the time it takes to travel for the left and right lights be t1=l/2(c+v) and t2=l/2(c-v) respectively. Now we suppose that the clock hands will move if the light reaches at each end, so in the train's frame, both light reaches at the same time, therefore the hands will tick at the same time. And for the outside observer, there will be a time difference of t1-t2, so that's the same time difference between the movements of the hands. So we get t1-t2=vl/(c^2-v^2) which is not the same as lv/c^2, since it has an extra factor of the square of the Lorentz factor...Why is this other thought experiment wrong (well, it gives a different answer) when it seems to be right?
Last edited: