A Renormalizability conditions for a real scalar field in d dimensions

[JD_PM]

TL;DR Summary

I want to understand how the $n$ powers of $\phi$ are limited (in a renormalized theory) depending on the $d$ number of dimensions we consider as well as how to "render" our theory renormalizable for higher dimensions

I am studying the real scalar field theory in $d$ spacetime dimensions as beautifully presented by M. Srednicki QFT's draft book, chapter 18 (actually, for the sake of simplicity, let us include polynomial interactions of degree less than or equal to 6 only)

\begin{equation*}
\mathcal{L} = −\frac 1 2 Z_{\phi}\partial_{\mu} \phi \partial^{\mu} \phi - \frac 1 2 Z_m m^2 \phi^2 - \sum_{n \geq 3}^6 Z_n \frac{\lambda_n}{n!} \phi^n
\end{equation*}

We see that

\begin{equation*}
[\mathcal{L}] = d, \quad [\partial_{\mu}]=1, \quad [Z]=0, \quad \Rightarrow \quad [\phi] = \frac 1 2(d-2), \quad [m] = 1, \quad [\lambda_n] = d- \frac 1 2 n(d-2)
\end{equation*}

Where we noted that $[\phi^n] = \frac 1 2 n (d-2)$.

In order to have the whole picture, we need to work with the so called superficial degree of divergence, which is defined as the powers of momentum in the numerator minus the powers of momentum in the denominator. If $D \geq 0$ the diagram diverges, otherwise it does not. As an example, take the Saturn diagram for $\phi^4$ and obtain the Feynman amplitude. You'll see that it diverges as $D=8-6>0$.

I understand (after studying example 32.4 of the enlightening book by Lancaster & Blundell: QFT for the gifted amateur; btw I wish I have encountered this book years ago, it is beautiful!) that $D$ is given by the following formulas

$$D=dL-I$$

$$D=d-E$$

Where $L$, $I$ and $E$ stand for the number of loops, internal lines and external lines respectively.

OK, but Srednicki does not present $D$ in any of the above forms. He argues the superficial degree of divergence as follows (he uses as notation $g_n$ instead of $\lambda_n$).

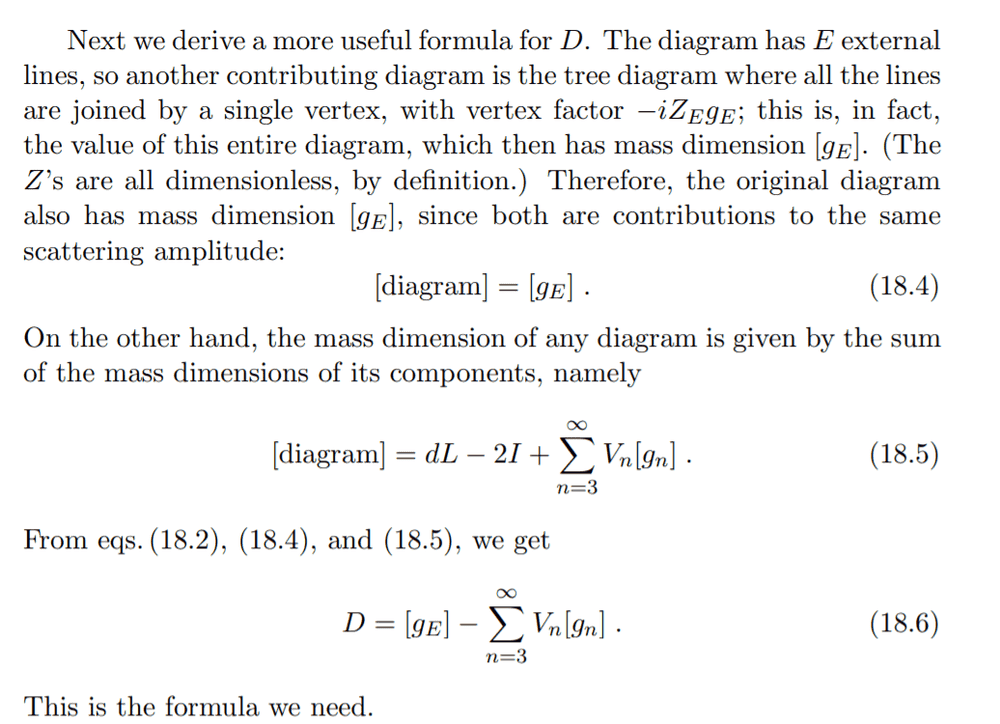
Based on (18.6) he argues that given any $[\lambda_n]<0$, our diagram diverges as $D>0$ (where he assumed that the dimension of the tree diagram $[g_E] > 0$ always). So we conclude that any theory with negative coupling constant is non-renormalizable.

Once we have made such conclusion and recalling $[\lambda_n] = d- \frac 1 2 n(d-2)$, we are ready to check how the $n$ powers of $\phi$ are limited depending on the $d$ number of dimensions we consider.

\begin{equation}
[\lambda_n]<0 \iff n> \frac{2d}{d-2} \tag{*}
\end{equation}

Questions

1) Why does he assume that the dimension of the tree diagram is $[g_E] > 0$ always? He states that "a theory is nonrenormalizable if any coefficient of any term in the lagrangian has negative mass dimension" but he does so after assuming $[g_E] > 0$, so I am confused.

2) What is the maximal value of $d$ for which this theory is renormalizable? Our model has terms up to $n=6$. Based on $(*)$, I would say that $d=3$. So if we wish to have $\phi^6$ power terms in a renormalizable theory, we need to work in $3$ dimensions.

3) Is it possible to kind of "render" our theory renormalizable for higher dimensions (say for $d=4,5,6,7,8,9,10$)? I guess that the answer is yes, by means of introducing polynomial potential terms (with integer powers of $n$). However I am not sure how this "render machinery" works (are these polynomial potential terms "counterterms"?). Might you please shed some light on it?

Thank you

 

[JD_PM]

Alright, I acknowledge I went a bit off on a tangent here . Please check this more on-point version and let me reformulate the questions

• How to check whether our theory is renormalizable when $d=2$ or not? Our formula $n> \frac{2d}{d-2}$ breaks down for such a case.

• Let's say we want to find renormalizable theories for higher dimensions. Based on $(*)$, for $d=4$ we see we would need to drop $\phi^5$ and $\phi^6$ terms. For $d=5,6$ we see we would need to drop $\phi^4$, $\phi^5$ and $\phi^6$. For $d=7,8,9,10,..,10^6,...$ (I did not check further than $d=10^6$ but it seems that the relation holds for further dimensions) we see we would need to drop $\phi^3$, $\phi^4$, $\phi^5$ and $\phi^6$. Does this mean that the simplest scalar field theory $\mathcal{L} = \frac 1 2 \partial_{\mu} \phi \partial^{\mu} \phi - \frac 1 2 m^2 \phi^2$ is renormalizable for all dimensions?

 

[vanhees71]

Have a look at Sect. 5.5 in the following manuscript

https://itp.uni-frankfurt.de/~hees/publ/lect.pdf

where the power counting is done for $\phi^4$ theory. You can easily generalize this to theories with higher powers and vertices with derivative couplings.