Rényi entropy becomes von Neumann entropy

[Lapidus]

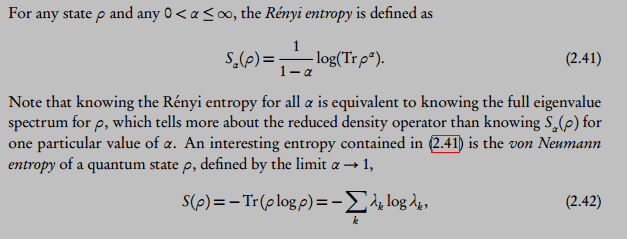
In holographic entanglement entropy notes like here, they let alpha go to one in (2.41) and get (2.42). But (2.41) goes towards infinity, when doing that! Can someone explain how alpha --> 1 will make (2.41) into (2.42)? Thank you!

 

[ShayanJ]

You should keep in mind that $Tr \rho=1$. So $\displaystyle \lim_{\alpha \to 1} \log(Tr \rho^\alpha)=0$. So $\displaystyle \lim_{\alpha \to 1}S_\alpha(\rho)=\frac 0 0$ and is indeterminate. To calculate it, you need to use L'Hopital's rule and differentiate the numerator and denominator w.r.t. $\alpha$ and then take the limit.