Replacing Variables in Integration

[Amad27]

I have asked the same question on math stackexchange under the moniker "anonymous," since I do not wish to be known there. I will try my luck here.$$I = \int_{-\infty}^{\infty} e^{-x^2} dx$$

I don't understand, we say:

$$I = \int_{-\infty}^{\infty} e^{-x^2} dx$$

Then we say:

$$I = \int_{-\infty}^{\infty} e^{-t^2} dt$$

I want to see how this process works?

We consider

$$f(x) = e^{-x^2}$$

Right?

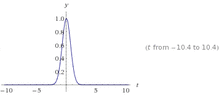
View attachment 3784

Then we say that:

$$f(t) = e^{-t^2}$$

View attachment 3785

Right? Ideally we are replacing x with t correct? Then we say: where R is the whole real axis.

$$I^2 = \int_{R} \int_{R} e^{-(t^2 + x^2)} dtdx$$

We had two different functions, one of variable $t$ and one of variable $x$. They are both "free variables," but when you convert it into a 3D system.

Arent you implicitly, relation $x$ and $t$ now? How are we making a relation? In terms of the geometrical aspect?
How does this work?

Thanks

 

[Ackbach]

$x$ and $t$ are independent variables. I actually prefer to use $x$ and $y$, because then it's a bit easier to think of this in terms of a two-dimensional integral. The proof goes like this:
\begin{align*}
I&=\int_{-\infty}^{\infty}e^{-x^2} \, dx \\
&=\int_{-\infty}^{\infty}e^{-y^2} \, dy \quad x \; \text{and} \; y \;
\text{are dummy variables} \\
I^2&=\int_{-\infty}^{\infty}e^{-x^2} \, dx \cdot
\int_{-\infty}^{\infty}e^{-y^2} \, dy \\
&=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-x^2} \cdot e^{-y^2} \, dx
\, dy \\
&=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-(x^2+y^2)} \, dx \, dy \\
&=\int_{0}^{2\pi}\int_{0}^{\infty}e^{-r^2} r \, dr \, d\theta \quad
\text{switching to polar coordinates}. \\
\end{align*}
Now you can substitute either $u=-r^2$ or $u=e^{-r^2}$ to finish. You get that $I^2=\pi$, so $I=\sqrt{\pi}$.

Does this answer your question?

 

[Amad27]

$x$ and $t$ are independent variables. I actually prefer to use $x$ and $y$, because then it's a bit easier to think of this in terms of a two-dimensional integral. The proof goes like this:
\begin{align*}
I&=\int_{-\infty}^{\infty}e^{-x^2} \, dx \\
&=\int_{-\infty}^{\infty}e^{-y^2} \, dy \quad x \; \text{and} \; y \;
\text{are dummy variables} \\
I^2&=\int_{-\infty}^{\infty}e^{-x^2} \, dx \cdot
\int_{-\infty}^{\infty}e^{-y^2} \, dy \\
&=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-x^2} \cdot e^{-y^2} \, dx
\, dy \\
&=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-(x^2+y^2)} \, dx \, dy \\
&=\int_{0}^{2\pi}\int_{0}^{\infty}e^{-r^2} r \, dr \, d\theta \quad
\text{switching to polar coordinates}. \\
\end{align*}
Now you can substitute either $u=-r^2$ or $u=e^{-r^2}$ to finish. You get that $I^2=\pi$, so $I=\sqrt{\pi}$.

Does this answer your question?

Hello @Ackbach, thanks for the reply. A slight question.

This is the issue:

$$I=\int_{-\infty}^{\infty}e^{-x^2} \, dx$$

What you are doing is:

let $x = y \implies dx = dy$ Then

$$I = \int_{-\infty}^{\infty}e^{-y^2} \, dy$$

But when you combine it:

$$I = \int_{R}\int_{R} e^{-(x^2 + y^2)} dxdy$$

This is really:

$$I = \int_{R}\int_{R} e^{-2x^2} (dx)^2$$

Applying $x^2 + y^2 = r^2$ requires $x$ to be perpendicular to $y$.

Which means it requires one to be the "range part," and one to be the "domain part." Doesn't it? Due to the Pythagorean theorem?

QUESTION #2

We defined in the beginning,

$$h(x) = e^{-x^2}$$

$$\therefore, h(y) = e^{-y^2}$$

But so $x$ and $y$ are colinear, lie in the same line. $y = x$ is not necessary. But still, when you make this 3D, $x$ and $y$ cannot have different axes can they?

 

[Ackbach]

Hello @Ackbach, thanks for the reply. A slight question.

This is the issue:

$$I=\int_{-\infty}^{\infty}e^{-x^2} \, dx$$

What you are doing is:

let $x = y \implies dx = dy$ Then

$$I = \int_{-\infty}^{\infty}e^{-y^2} \, dy$$

Right.

But when you combine it:

$$I = \int_{R}\int_{R} e^{-(x^2 + y^2)} \, dx \, dy$$

This is really:

$$I = \int_{R}\int_{R} e^{-2x^2} (dx)^2$$

The problem with doing this is that it violates the independence of $x$ and $y$. $x=y$ is really not safe to say here. An analogy would be vector addition. You can't add magnitudes of vectors to get the magnitude of the resultant vector, right? (That is, you can't do that unless both vectors are pointing in the same direction.) $|\vec{a}+\vec{b}|\not=|\vec{a}|+|\vec{b}|$ in general. In fact, $|\vec{a}+\vec{b}|\le |\vec{a}|+|\vec{b}|$, the Triangle Inequality.

Applying $x^2 + y^2 = r^2$ requires $x$ to be perpendicular to $y$.

Right.

Which means it requires one to be the "range part," and one to be the "domain part." Doesn't it?

No, it doesn't. You need to increase the number of dimensions you're thinking in. The domain is not the real line. It is the $xy$ plane, and the function $e^{-(x^2+y^2)}$ gives you a range in the $z$ direction. That is, you need to be thinking about a function $z=f(x,y)$ of two variables, not the usual Calculus I or II idea of a function of one variable $y=f(x)$. Why must this be? Because the theorem that let's you do
$$\int_{-\infty}^{\infty}e^{-x^2} \, dx \cdot
\int_{-\infty}^{\infty}e^{-y^2} \, dy=
\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-(x^2+y^2)} \, dx \, dy$$
assumes that $x$ and $y$ are independent.

Due to the Pythagorean theorem?

Not sure the Pythagorean Theorem would apply here, except in the transition to polar coordinates.

QUESTION #2

We defined in the beginning,

$$h(x) = e^{-x^2}$$

$$\therefore, h(y) = e^{-y^2}$$

But so $x$ and $y$ are colinear, lie in the same line.

They are not collinear. For one thing, $x$ and $y$ are each variables, not vectors or lines. For another, the independence of $x$ and $y$ means you can think of the analogy of two perpendicular directions to aid in calculations, as opposed to some kind of dependence between $x$ and $y$.

$y = x$ is not necessary.

It is not just not necessary: it is necessarily not so!

But still, when you make this 3D, $x$ and $y$ cannot have different axes can they?

They must, actually, because they are independent.

 

[Amad27]

Right.
The problem with doing this is that it violates the independence of $x$ and $y$. $x=y$ is really not safe to say here. An analogy would be vector addition. You can't add magnitudes of vectors to get the magnitude of the resultant vector, right? (That is, you can't do that unless both vectors are pointing in the same direction.) $|\vec{a}+\vec{b}|\not=|\vec{a}|+|\vec{b}|$ in general. In fact, $|\vec{a}+\vec{b}|\le |\vec{a}|+|\vec{b}|$, the Triangle Inequality.
Right.
No, it doesn't. You need to increase the number of dimensions you're thinking in. The domain is not the real line. It is the $xy$ plane, and the function $e^{-(x^2+y^2)}$ gives you a range in the $z$ direction. That is, you need to be thinking about a function $z=f(x,y)$ of two variables, not the usual Calculus I or II idea of a function of one variable $y=f(x)$. Why must this be? Because the theorem that let's you do
$$\int_{-\infty}^{\infty}e^{-x^2} \, dx \cdot
\int_{-\infty}^{\infty}e^{-y^2} \, dy=
\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-(x^2+y^2)} \, dx \, dy$$
assumes that $x$ and $y$ are independent.
Not sure the Pythagorean Theorem would apply here, except in the transition to polar coordinates.
They are not collinear. For one thing, $x$ and $y$ are each variables, not vectors or lines. For another, the independence of $x$ and $y$ means you can think of the analogy of two perpendicular directions to aid in calculations, as opposed to some kind of dependence between $x$ and $y$.
It is not just not necessary: it is necessarily not so!
They must, actually, because they are independent.

Hi Ackbach, The biggest question: so the definition of a variable in general is that, it can be replaced if it is replaced EVERYWHERE?

So all variables are dummy variables in theory?

From the beginning (of your post), so really we are not considering $x=y$ because that would create future problems?Okay, so let me start thinking in 3 Dimensions, which is quite difficult.

When you said:

Because the theorem that let's you do
$$\int_{-\infty}^{\infty}e^{-x^2} \, dx \cdot
\int_{-\infty}^{\infty}e^{-y^2} \, dy=
\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}e^{-(x^2+y^2)} \, dx \, dy$$
assumes that $x$ and $y$ are independent.

Which theorem is this? Fubini's?

For me personally it feels simply weird, converting $2D$ functions into $3D$ functions.

I am severely confused,

Are we considering just one function $f$ for which we have:

$$f(x) = e^{-x^2}$$
$$f(y)=e^{-y^2}$$

Or consider two different functions $f, g$?

Ifso, why?Also, you said $x$ and $y$ weren't collinear.

I said that because $x$ and $y$ both lie on the horizontal axis of the 2D plane?

I might be missing out on this.

From the **beginning** are you already consider a multivariable function?

$f(x, y) = e^{-x^2}$

$g(x,y) = e^{-y^2}$

Considering two separate ALREADY multivariable functions from the beginning?

I think that is what the method is doing, I believe.

Please tell me if I am right.

Also then how do you derive $x^2 + y^2 = r^2$?? Thanks!