- #1
aaas
- 8
- 0
Homework Statement
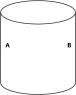
A circular disk of radius r and thickness d is made of material with resistivity p . Show that the resistance between points a and b (Fig. attached)is independent of r and is given by R=πp /2d
Homework Equations
R= p L / A
where L and A are length and section area respectively .
The Attempt at a Solution
I tried to use differential equations , but I always end up with ∞ or some indeterminate forms .