ResourcesHelp|Close Moment of a Force: Scalar Formulation

[sami23]

Homework Statement

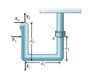
A pipe is anchored to a wall at point A. During the pipe's installation, several forces are applied to the pipe at different locations. If F₁ = 14.7 lb, F₂ = 18.5 lb, F₃ = 12.6 lb, F₄ = 10.9 lb, d₁ = 0.400 ft, d₂ = 0.800 ft, and d₃ = 0.800 ft, what is M_RA, the net moment about point A due to these forces? Assume that moments acting counterclockwise about point A are positive whereas moments acting clockwise are negative.

Homework Equations

M_RA = $$\Sigma$$Fd

The Attempt at a Solution

M_RA = F₁d₂ - F₂d₂ + F₃(d₃ - d₁) - F₄d₃

not sure about the direction (using the right-hand rule)

 

[sami23]

Help!