Resultant force and centre of pressure in a tank

[Guillem_dlc]

Homework Statement

The tank in the figure contains water and a uniform pressure $p_a$ acts on its surface. We wish to determine the resultant force $F$, and its centre of pressure $y_c-y_G$, on a flat gate of base $3\, \textrm{m}$ and height $1,5\, \textrm{m}$. The damper is located on a wall of the tank with an inclination of $30$ degrees with respect to the horizontal. The upper base of the damper is located at $3\, \textrm{m}$ depth. Consider the following cases: a) $p_a=p_{\textrm{atm}}$ and b) $p_a=125\, \textrm{kPa}$.

Relevant Equations

$I_{xx}=\iint y^2\, dA$

Figure:

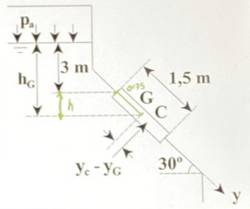
a) CASE A $\rightarrow p_a=101300\, \textrm{Pa}$
$$F_{\textrm{res}}?,\,\, y_c-y_{cg}=y_{cp}$$
We find $h_{cg}\rightarrow h_{cg}=3+h$
$$h=0,75\cdot \sin (30)=0,375\, \textrm{m}\rightarrow h_{cg}=3,375\, \textrm{m}$$
$$p_{cG}=\rho_{H2O}gh_{cg}=33108,75\, \textrm{Pa}$$
We calculate $A=1,5\cdot 3=4,5\, \textrm{m}^2$
$$\boxed{F=p_{CG}\cdot A=148989,375\, \textrm{N}}$$
$$I_{xx}=\iint y^2\, dA=\int_0^{1,5}\int_0^3 y^2\, dxdy=\int_0^{1,5}y^2\int_0^3 dxdy=3\int_0^{1,5}y^2\, dy=$$
$$=3\left[ \dfrac{y^3}{3}\right]_0^{1,5}=3,375$$
$$y_{CP}=-\dfrac{\rho_{H2O}g\sin \theta I_{xx}}{F_{CG}}=0,111$$
I don't get the $I_{xx}$ right and I don't know why? Is it calculated in this way?
$$I_{xx}=\iint y^2\, dA$$
It should give $\dfrac{b\cdot L^3}{12}$.

 

[erobz]

You are supposed to be looking for the effective force acting on the gate, and it’s applied location(the center of pressure). The gate is in static equilibrium. The moment of inertia of the gate is not going to be a factor, it is not a dynamics problem.

Isolate the gate, assume it’s being held closed. Sketch the pressure distribution acting over its interior for the different gauge pressures acting over surface $a$. Use that to determine the effective force on the gates interior, and it’s location using the proper formulas for those quantities.

 

[erobz]

The Second Moment of Area (Not Moment of Inertia - Thanks @Lnewqban) is a factor! I'm sorry...I forgot it was in the formula for $y_{cp}$. Anyhow, you don't have to do the integral, you have it written in the last part of the OP.

 

[Lnewqban]

In this case, the given relevant equation refers to the second moment of area.

Please, see:
https://en.m.wikipedia.org/wiki/Second_moment_of_area#Rectangle_with_centroid_at_the_origin

Note that the center of pressure, and the resultant single-vector force, are located off the geometrical center of the damper, due to the inequal distribution of unitary pressures and forces from top to bottom.

 

[Guillem_dlc]

With the integral I have not done this in the end?

 

[erobz]

For part (a) your force looks correct.

What they are asking you to find is:

$$ y_{cp} = \bar{y} + \frac{ \bar{I} }{ \bar{y} A} $$

$$ ( y_{cp} - \bar{y} ) = \frac{ \bar{I} }{ \bar{y} A} $$

$$y_{CP}=-\dfrac{\rho_{H2O}g\sin \theta I_{xx}}{F_{CG}}=0,111$$

Where does the negative sign come from, and where did it go?

That expression is not equal to $y_{cp}$ (see above).

If you are going to use the Force and the density to calculate the area of the plate $A$ (which unnecessary and introduces computational error), label it correctly as $F$. Its not $F_{CG}$.

I feel like you have made a mess of things trying to be overly clever with substitutions. I think its correct (other than the minus sign and the labeling), but you have not made it easy to check.

 

[erobz]

You are correct, that whatever you are going to do after that should result in (for the rectangle):

$$I_{xx} = \frac{1}{12}bh^3$$

 

[Guillem_dlc]

Where does the negative sign come from, and where did it go?

As $y_{cp}$ is a measure of the centre of gravity, I have given the result as an absolute value.

But maybe I should have left the sign to indicate where it goes.

For example, when you want to find the height of the centre of pressures then I do $h_{cp}=h_{cg}+y_{cp}$ with the sign I have been given from the formula so I know if it is higher or lower, but otherwise I put it in absolute value.

 

[erobz]

The actual question you have is what you've done incorrectly calculating the second moment of area of a rectangular plate?

For starters the limits of integration are wrong. Put coordinate system through the centroid of the area. What are the limits of integration with respect to those axes?

 

[erobz]

As $y_{cp}$ is a measure of the centre of gravity, I have given the result as an absolute value.

$y_{cp}$ is the center of pressure, not the center of gravity. The depths are measured as positive downward from the surface. $( y_{cp} - \bar{y}) > 0$ for these types of problems. No need for absolute values.

 

[Guillem_dlc]

The actual question you have is what you've done incorrectly calculating the second moment of area of a rectangular plate?

For starters the limits of integration are wrong. Put coordinate system through the centroid of the area. What are the limits of integration with respect to those axes?

$-0,75$ to $0,75$ and $-1,5$ to $1,5$?

 

[erobz]

$-0,75$ to $0,75$ and $-1,5$ to $1,5$?

ok, just leave it in parameters $h$ and $b$ for now. What are the limits for the integration w.r.t. $y$ in terms of $h$, and $x$ in terms of $b$ respectively?

 

[Guillem_dlc]

ok, just leave it in parameters $h$ and $b$ for now. What are the limits for the integration w.r.t. $y$ in terms of $h$, and $x$ in terms of $b$ respectively?

$-h/2$ to $h/2$ and $-b/2$ to $b/2$

 

[erobz]

Good. You can do that integration or (highly recommended), using the arguments of symmetry make life easier.

$$ I_{xx} = \int_{-b/2}^{b/2} \int_{-h/2}^{h/2} y^2 dy \, dx = 2 \int_{0}^{b/2} \int_{0}^{h/2} y^2 dy \, dx $$

 

[Guillem_dlc]

Good. You can do that integration or (highly recommended), using the arguments of symmetry make life easier.

$$ I_{xx} = \int_{-b/2}^{b/2} \int_{-h/2}^{h/2} y^2 dy \, dx = 2 \int_{0}^{b/2} \int_{0}^{h/2} y^2 dy \, dx $$

So if I have a circle I will have to switch to polars, right?

 

[erobz]

So if I have a circle I will have to switch to polars, right?

I don't know that you have to... probably would be a good idea.

 

[haruspex]

So if I have a circle I will have to switch to polars, right?

The problem is equivalent to finding the x coordinate of the mass centre of a vertical cylinder with a horizontal base but with a top sloped at some angle.
$\int_{-r}^r(a x+b)\sqrt{r^2-x^2}x.dx$
$\int_{-\pi}^\pi(ar\cos(\theta)+b)r\sin(\theta)r\cos(\theta).(-r)\sin(\theta)d\theta$