Review my solution: Trigonometry proof

[SweatingBear]

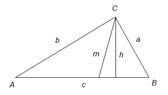
For a triangle with sides $$a$$, $$b$$, $$c$$ and angle $$C$$, where the angle $$C$$ subtends the side $$c$$, show that

$$c \geqslant (a+b) \sin \left( \frac C2 \right)$$
_______

Let us stipulate $$0^\circ < C < 180^\circ$$ and of course $$a,b,c > 0$$. Consequently, $$0^\circ < \frac C2 \leqslant 90^\circ \implies 0 < \sin^2 \left( \frac C2 \right) \leqslant 1$$ (we include $$90^\circ$$ for the angle $$\frac C2$$ in order to account for right triangles).

Law of cosines yield

$$c^2 = a^2 + b^2 - 2ab \cos ( C )$$

Using $$\cos (C) \equiv 1 - 2\sin^2 \left( \frac C2 \right)$$, we can write

$$c^2 = a^2 + b^2 - 2ab + 4ab\sin^2 \left( \frac C2 \right)$$

Now since $$0 < \sin^2 \left( \frac C2 \right) \leqslant 1$$, $$a^2 + b^2 - 2ab + 4ab\sin^2 \left( \frac C2 \right)$$ will either have to equal $$a^2 + b^2 - 2ab + 4ab$$ or be greater than it. Thus

$$c^2 \geqslant a^2 + b^2 - 2ab + 4ab = a^2 + 2ab + b^2 = (a+b)^2 $$

A similar argument can be made for $$(a+b)^2$$ versus $$(a+b)^2 \sin^2 \left( \frac C2 \right)$$: $$(a+b)^2$$ will either have to equal or be greater than the latter expression, due to the values $$\sin^2 \left( \frac C2 \right)$$ can assume. Therefore

$$c^2 \geqslant (a+b)^2 \geqslant (a+b)^2 \sin^2 \left( \frac C2 \right) $$

We can finally conclude

$$c \geqslant (a+b) \sin \left( \frac C2 \right)$$

Thoughts?

 

[MarkFL]

I see nothing wrong with your proof. This is how I would prove it. Please refer to the following diagram:

View attachment 1085

I would postulate concerning the angle bisector $m$ of $\angle C$ and the altitude $h$, we must have:

(1) $$m\ge h$$

That is, the shortest distance between a point and a line is the perpendicular distance.

Now, the area $T$ of the triangle may be written in these ways:

$$T=\frac{1}{2}ch=\frac{1}{2}m(a+b)\sin\left(\frac{C}{2} \right)$$

Note: I have made use of the formulas (for a general triangle):

$$T=\frac{1}{2}bh$$

$$T=\frac{1}{2}ab\sin(C)$$

Thus, we have:

$$ch=m(a+b)\sin\left(\frac{C}{2} \right)$$

And from (1), we therefore conclude:

$$c\ge (a+b)\sin\left(\frac{C}{2} \right)$$

 

[SweatingBear]

I see nothing wrong with your proof. This is how I would prove it. Please refer to the following diagram:

View attachment 1085

I would postulate concerning the angle bisector $m$ of $\angle C$ and the altitude $h$, we must have:

(1) $$m\ge h$$

That is, the shortest distance between a point and a line is the perpendicular distance.

Now, the area $T$ of the triangle may be written in these ways:

$$T=\frac{1}{2}ch=\frac{1}{2}m(a+b)\sin\left(\frac{C}{2} \right)$$

Note: I have made use of the formulas (for a general triangle):

$$T=\frac{1}{2}bh$$

$$T=\frac{1}{2}ab\sin(C)$$

Thus, we have:

$$ch=m(a+b)\sin\left(\frac{C}{2} \right)$$

And from (1), we therefore conclude:

$$c\ge (a+b)\sin\left(\frac{C}{2} \right)$$

Thank for the feedback and the excellent alternative solution!

 

[Deveno]

A good rule of thumb in mathematics I live by is this:

If something is true, you should be able to provide two proofs, and one of them should have a picture. This is a perfect example of what I mean.