Rewriting a given action via integration by parts

[JD_PM]

Homework Statement

Given the action

\begin{equation*}
S=\int d^4 x \left[ \frac{a^2}{2}\left(\dot \phi^2 -(\nabla \phi)^2\right)-a^4V(\phi) \right]
\end{equation*}

Where the dot denotes differentiation with respect to the conformal time $\eta$.



Show that by introducing small fluctuations to the scalar field $\phi$.


\begin{equation*}
\phi = \phi_0 (\eta) + \delta \phi (\eta, \vec x)
\end{equation*}


as well as defining $\chi := a\delta \phi$, the action becomes


\begin{equation*}

S = S[\phi_0] + \frac{1}{2} \int d^4 x \left( \dot \chi^2 - (\nabla \chi)^2 + \frac{\ddot a}{a} \chi^2 \right)

\end{equation*}

Relevant Equations

N/A

I simply plugged \phi = \phi_0 (\eta) + \delta \phi (\eta, \vec x) into the given action to get

\begin{align}
S &= \int d^4 x \left[ \frac{a^2}{2}\left(\dot \phi^2 -(\nabla \phi)^2\right)-a^4V(\phi) \right] \nonumber \\
&= \int d^4 x \left[ \frac{a^2}{2}\left(\dot \phi_0^2 + (\delta \dot \phi)^2 + 2\dot \phi_0 \delta \dot \phi- (\nabla (\delta \phi))^2 \right)-a^4V(\phi_0) -a^4V(\delta \phi) \right] \\
&= \int d^4 x \left[ \underbrace{\frac{a^2}{2} \dot \phi_0^2 + a^2\dot \phi_0 \delta \dot \phi -a^4V(\phi_0)}_{S[\phi_0]} + \frac{a^2}{2}(\delta \dot \phi)^2 - \frac{a^2}{2}(\nabla (\delta \phi))^2 -a^4V(\delta \phi) \right]
\end{align}
However, I am stuck in how to show that

\begin{equation*}
\int d^4 x \left[ \frac{a^2}{2}(\delta \dot \phi)^2 - \frac{a^2}{2}(\nabla (\delta \phi))^2 -a^4V(\delta \phi) \right] = \frac{1}{2} \int d^4 x \left( \dot \chi^2 - (\nabla \chi)^2 + \frac{\ddot a}{a} \chi^2 \right)
\end{equation*}

I am convinced we'll have to use integration by parts and let the surface term vanish but I do not see how to do so...

Any help is appreciated.

Thank you

This doubt emerged while studying the lecture notes of my course. I attach the relevant pages

 

[etotheipi]

Hey JD, did you ever figure out how to get the answer? I was just trying to do it now but got stuck as well. So far I'd just written $V(\phi) = V(\phi_0) + \delta \phi V'(\phi_0)$ and then\begin{align*}
S &= S[\phi_0] + \int d^4 x \left[ \frac{a^2}{2} \left( 2\dot{\phi}_0 \delta \dot{\phi} + \delta \dot{\phi}^2 -2 \nabla \phi_0 \cdot \nabla \delta \phi - (\nabla \delta \phi)^2 \right) - a^4 \delta \phi V'(\phi_0)\right] \\

&= S[\phi_0] + \int d^4 x [ a^2 \dot{\phi}_0 \left( \frac{\dot{\chi}}{a} - \frac{\dot{a} \chi}{a^2} \right) + \frac{a^2}{2} \left(\frac{\dot{\chi}^2}{a^2} - \frac{2\dot{a} \dot{\chi} \chi}{a^3} + \frac{\dot{a}^2 \chi^2}{a^4} \right) -a \nabla \phi_0 \cdot \nabla \chi \\

&\quad \quad \quad \quad \quad - \frac{1}{2} (\nabla \chi)^2 -a^4 \delta \phi V'(\phi_0)] \\

&= S[\phi_0] + \frac{1}{2} d^4 x \left[ \dot{\chi}^2 - (\nabla \chi)^2 + \underbrace{2a \dot{\phi}_0 \dot{\chi} + 2a \chi \ddot{\phi}_0 - \frac{2\dot{a} \dot{\chi} \chi}{a} + \frac{\dot{a}^2 \chi^2}{a^2} - 2a \nabla \phi_0 \cdot \nabla \chi} \right]
\end{align*}
in the last line I used that $-a^2 V'(\phi_0) = \ddot{\phi}_0 + \frac{2\dot{a}}{a} \dot{\phi}_0$. But I'm at a loss about how to proceed

 

[JD_PM]

Hey James! Nice to see you taking the problem

\begin{align*}
S &= S[\phi_0] + \int d^4 x \left[ \frac{a^2}{2} \left( 2\dot{\phi}_0 \delta \dot{\phi} + \delta \dot{\phi}^2 -2 \nabla \phi_0 \cdot \nabla \delta \phi - (\nabla \delta \phi)^2 \right) - a^4 \delta \phi V'(\phi_0)\right] \\
\end{align*}

Notice that we are dealing with a homogeneous scalar field i.e. $\partial_i \phi = 0$ so the term $2 \nabla \phi_0 \cdot \nabla \delta \phi$ drops. Hence you'll agree with me that we start off by

\begin{equation*}
S=\int d^4 x \left( \underbrace{\frac{a^2}{2} \dot \phi_0^2 - a^4V(\phi_0)}_{= S[\phi_0]} + \frac{a^2}{2}(\delta \dot \phi)^2 - \frac{a^2}{2}(\nabla \delta \phi)^2 +a^2\dot \phi_0 \delta \dot \phi - a^4 \delta \phi V'(\phi_0) \right)
\end{equation*}

To advance, you will need to work out the term $\delta \phi$ based on change of variables (39) in the attached pic. If it goes smooth you will find

\begin{align*}
S &= S[\phi_0] \nonumber \\
&+ \int d^4 \vec x \left[\frac{1}{2} \left( \dot \chi^2 - 2\frac{\dot a \chi \dot \chi}{a} + \frac{\dot a^2}{a^2} \chi^2 \right) -\frac 1 2 (\nabla \chi)^2 + a^2 \dot \phi_0 \delta \dot \phi - a^4\delta \phi V'(\phi_0)\right]
\end{align*}

Once we get here we will carry on

 

[etotheipi]

Yes these results are in agreement if $2 \nabla \phi_0 \cdot \nabla \delta \phi = 0$, because if you substitute this term\begin{align*}
a^2 \dot{\phi}_0 \delta \dot{\phi} - a^4 \delta \phi V'(\phi) &= a \dot{\chi} \dot{\phi}_0 - \dot{a} \dot{\phi}_0 \chi + \left[ a^2 \delta \phi \ddot{\phi}_0 + 2a \dot{a} \dot{\phi}_0 \delta \phi \right] \\

&= a \dot{\chi} \dot{\phi}_0 - \dot{a} \dot{\phi}_0 \chi + \left[ a \chi \ddot{\phi}_0 + \dot{a} \dot{\phi}_0 \chi \right] \\

&= a \dot{\chi} \dot{\phi}_0 + a \chi \ddot{\phi}_0

\end{align*}in your final expression, then you end up with my expression at the end of #2.

 

[JD_PM]

Good! At this point we notice that we already have 2 of the 3 terms in the desired final form. All we need to do is simplify.

Try to integrate by parts (IBP¨) the $2\frac{\dot a \chi \dot \chi}{a}$ term. Your action should simplify slightly. Then make use of the E.O.M. (37). Finally you will have to use IBP once again to get the final simplified action :)

 

[etotheipi]

Write\begin{align*}

-\int d^3 x d\eta \frac{2\dot{a}\chi \dot{\chi}}{a} &= - \int d^3 x d\eta \frac{\dot{a}}{a} \frac{d}{d\eta} \left( \chi^2 \right) \\

&= -\int d^3 x d\eta \left[ \frac{d}{d\eta} \left( \frac{\dot{a}}{a} \chi^2 \right) - \chi^2 \left( \frac{a\ddot{a} - \dot{a}^2}{a^2} \right) \right] \\

&= \phantom{-} \int d^3 x d\eta \left( \frac{\ddot{a}}{a} \chi^2 - \frac{\dot{a}^2}{a^2} \chi^2 \right)

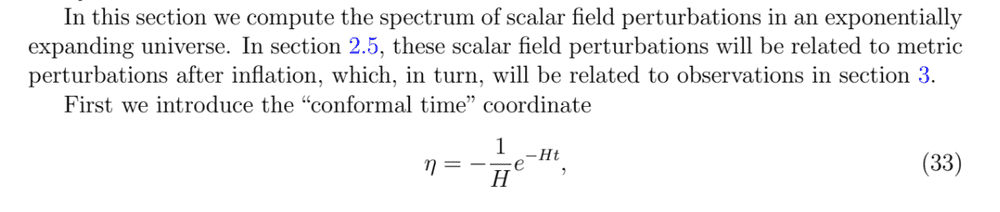
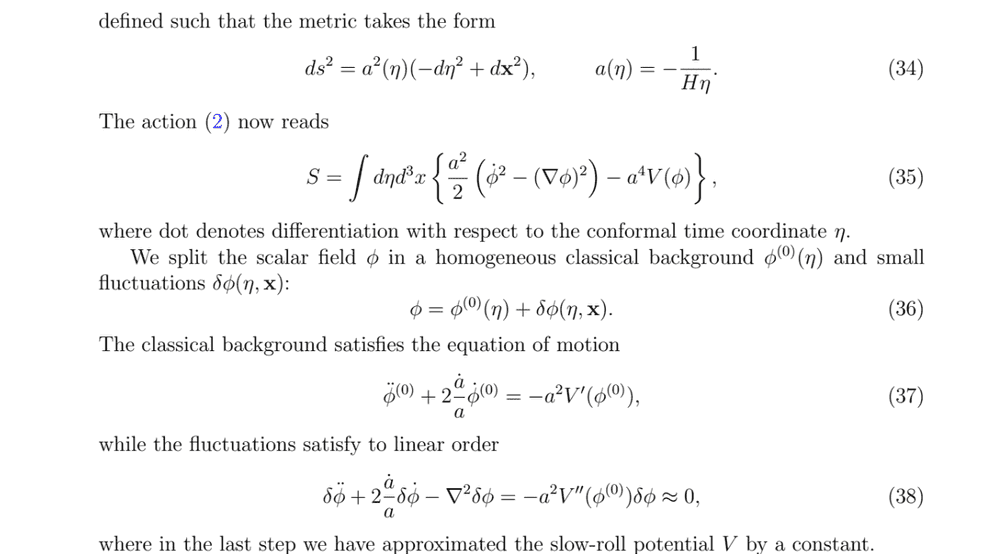
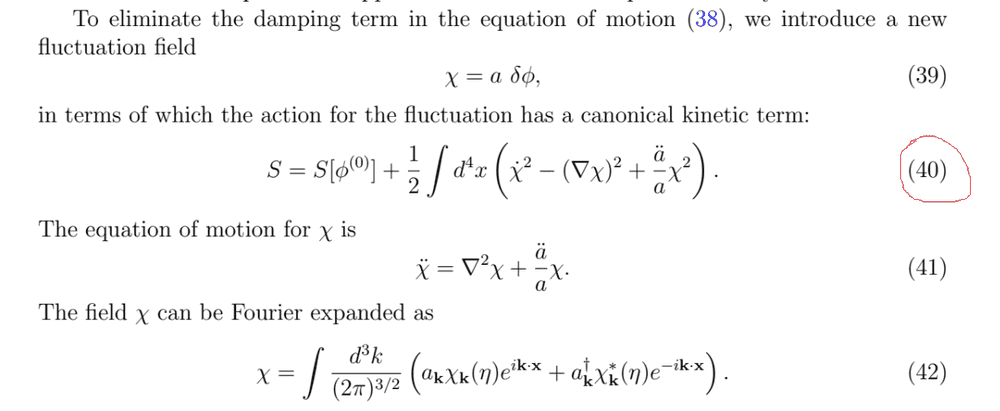
\end{align*}where the boundary term was thrown away. The expression becomes\begin{align*}

S = S[\phi_0] + \frac{1}{2} \int d^4 x \left[ \dot \chi^2 + \frac{\ddot a}{a} \chi^2 -(\nabla \chi)^2 + 2a^2 \dot \phi_0 \delta \dot \phi - 2a^4\delta \phi V'(\phi_0)\right]

\end{align*}Now use equation $(37)$ to re-write $2a^2 \ddot{\phi}_0 \delta \phi + 4a \dot{a} \dot{\phi}_0 \delta \phi = -2a^4 \delta \phi V'(\phi_0)$, such that\begin{align*}
\int d^4 x \left[ 2a^2 \dot \phi_0 \delta \dot \phi - 2a^4\delta \phi V'(\phi_0)\right] &= \int d^4 x \left[ 2a^2 \dot \phi_0 \delta \dot \phi +2a^2 \ddot{\phi}_0 \delta \phi + 4a \dot{a} \dot{\phi}_0 \delta \phi \right] \\

&= \int d^3 x d\eta \left[ \frac{d}{d\eta} \left( 2a^2 \dot{\phi}_0 \delta \phi \right)\right] \\

&=0

\end{align*}again neglecting the boundary term. Hence\begin{align*}
S = S[\phi_0] + \frac{1}{2} \int d^4 x \left[ \dot \chi^2 + \frac{\ddot a}{a} \chi^2 -(\nabla \chi)^2 \right]
\end{align*}