Rings Generated by Elements - Lovett, Example 5.2.1 .... ....

[Math Amateur]

I am reading Stephen Lovett's book, "Abstract Algebra: Structures and Applications" and am currently focused on Chapter 5 ...

I need some help with Example 5.2.1 in Section 5.2: Rings Generated by Elements ...

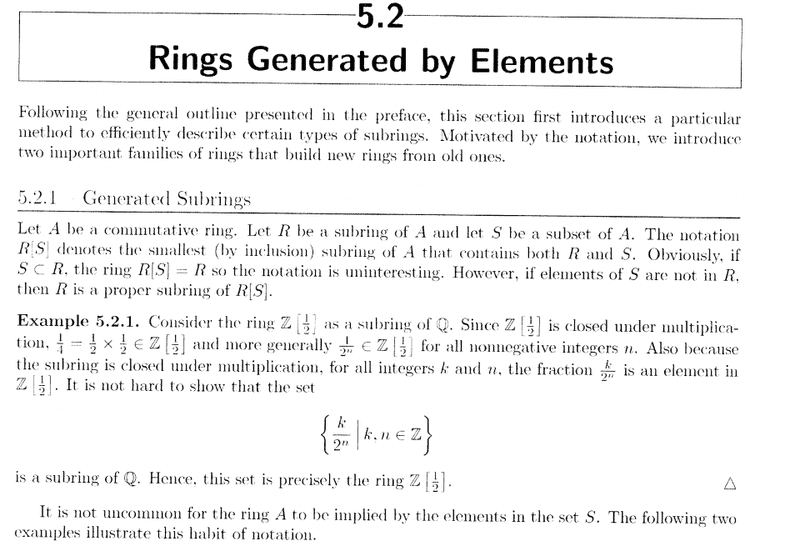
In the Introduction to Section 5.2.1 (see text above) Lovett writes:

" ... ... R[S] denotes the smallest (by inclusion) subring of $A$ that contains both $R$ and $S$ ... ... "Then, a bit later, in Example 5.2.1 concerning the ring $\mathbb{Z} [ \frac{1}{2} ]$ Lovett writes:" ... ... It is not hard to show that the set$\{ \frac{k}{ 2^n} \ | \ k.n \in \mathbb{Z} \}$is a subring of $\mathbb{Q}$. Hence, this set is precisely the ring $\mathbb{Z} [ \frac{1}{2} ]$ ... ... ... "BUT ...

How has Lovett actually shown that the set $\{ \frac{k}{ 2^n} \ | \ k.n \in \mathbb{Z} \}$ as a subring of \mathbb{Q} is actually (precisely in Lovett's words) the ring $\mathbb{Z} [ \frac{1}{2} ]$ ... ...

... ... according to his introduction which I quoted Lovett says that the ring $\mathbb{Z} [ \frac{1}{2} ]$ is the smallest (by inclusion) subring of $\mathbb{Q}$ that contains $\mathbb{Z}$ and $\frac{1}{2}$ ... ...Can someone please explain to me exactly how Lovett has demonstrated this ... ...

... and ... if Lovett has not clearly proved this can someone please demonstrate a proof ...Just one further clarification ... is Lovett here dealing with ring extensions ... ... ?

Hope someone can help ...

Peter

 

[FactChecker]

1) {k/2ⁿ | k,n∈ℤ} is a subring that contains ℤ and 1/2. Therefore, ℤ[1/2] ⊆ {k/2ⁿ | k,n∈ℤ}.
2) Pick any element k/2ⁿ, k,n∈ℤ. For ℤ[1/2] to be a closed ring under an obvious series of operations, it must contain that element. Therefore {k/2ⁿ | k,n∈ℤ} ⊆ ℤ[1/2].

 

[Math Amateur]

Thanks FactChecker ... I think I now follow ...

Appreciate your help ...

Peter