John Mcrain
- 435
- 28
Can you explain with example what mean rotation is absolute and linear motion is relative?
In windowless box you cant tell if box is rotating at turntable or accelerate in straight lineIbix said:I don't think "absolute" is particularly well defined in this context. But usually what is meant is that you can measure acceleration in a "closed box" type experiment, but not linear velocity. If I put you in a windowless lab mounted on a turntable you can immediately detect if the turntable is rotating or not, but not whether it's in linear motion or not - in fact, there's no real meaning to "in linear motion" without reference to some state of rest.
The underlying fact is that you can always detect if you are inertial or not. If you are rotating you are not moving inertially - or at least not all of you is.
Please, try this ride:John Mcrain said:In windowless box you cant tell if box is rotating at turntable or accelerate in straight line
Sure you can. The acceleration vectors point in different directions and/or have different magnitudes at different points in the rotating case but not in the linear case. With sufficiently precise measurements you could see this. If you can't do sufficiently precise measurements to spot the variation then you could get it wrong, yes.John Mcrain said:In windowless box you cant tell if box is rotating at turntable or accelerate in straight line
If box is not place at the center of constant turning table, all 4 walls of box has same accelerations vectors directions like box accelerate in straight line.Ibix said:Sure you can. The acceleration vectors point in different directions and/or have different magnitudes at different points in the rotating case but not in the linear case. With sufficiently precise measurements you could see this. If you can't do sufficiently precise measurements to spot the variation then you could get it wrong, yes.
This is wrong. Do you know the expression for centripetal force?John Mcrain said:If box is not place at the center of constant turning table, all 4 walls of box has same accelerations vectors directions like box accelerate in straight line.
But fluid flows in the circular canals of the ears. That detects rotation.John Mcrain said:Once when G simulator reach constant RPM, men in the chair dont know if he travel in cirlce or accelerate in straight line.
mxV2 / r , so at all 4 walls of box acceleration dircetions is toward center, but magnitude is different ...Ibix said:This is wrong. Do you know the expression for centripetal force?
You mean ears detects synchronous rotation of men?Baluncore said:But fluid flows in the circular canals of the ears. That detects rotation.
Yes. And "towards the center" isn't the same direction at different points on a wall, unless the wall happens to be aligned radially.John Mcrain said:mxV2 / r , so at all 4 walls of box acceleration dircetions is toward center, but magnitude is different ...
How would men in chair in G simulator know if he travel in circle or accelerate in straight line?Ibix said:Yes. And "towards the center" isn't the same direction at different points on a wall, unless the wall happens to be aligned radially.
If he tosses a ball forward and it appears to curve, he would know.John Mcrain said:How would men in chair in G simulator know that he is travel in circle and not accelerate in straight line?
Hang plumb lines and compare the angles of fall.John Mcrain said:How would men in chair in G simulator know if he travel in circle or accelerate in straight line?
In both case seat push his back.
Synchronous with what?John Mcrain said:You mean ears detects synchronous rotation of men?
If you put bucket with water close to wall,will be water behaves identical like accelerate in straight line car?Baluncore said:Synchronous with what?
You are assuming that a man is a solid, has no fluid content, and cannot move or turn his head.
What if he was forced against the wall by the rotation, body parallel with the axis of rotation. He then reverses the position of his head and feet. Would his ears not tell him that he was rotating in the other direction?
It depends on how large the bucket is compared to the radius of the centrifuge. The centrifugal force is not uniform like the inertial force in linear acceleration of the car.John Mcrain said:If you put bucket of water close to wall,will be water behaves identical like accelerate in straight line car?
The water surface in the bucket placed in the cabin of the G-simulator also forms a section of that U-shape. But if the bucket is small compared to the radius of the centrifuge this might not be noticeable, and just look like a tilted plane surface.John Mcrain said:But if bucket is set at the center of G-simulator,, water will take U-shape.
What casuse this U shape when bucket is at wall in Groom?From ground frame bucket with water set at the wall of G-room rotate around itslef because it change orientation.A.T. said:The water surface in the bucket placed in the cabin of the G-simulator also forms a section of that U-shape. But if the bucket is small compared to the radius of the centrifuge it might not be noticeable, and just look like a tilted plane surface.
The parabolic surface extends throughout the Groom. You can sample that curved surface with a bucket of water anywhere in the room.John Mcrain said:What casuse this U shape when bucket is at wall in Groom?
Yes.John Mcrain said:If we stop rotate G-room, will be water inside bucket continued to spin for some time?
The non-uniform centrifugal force field.John Mcrain said:What casuse this U shape when bucket is at wall in Groom?
What do you mean by "U shape"? Once the rotation axis is outside the bucket there will no longer be a dip in the middle of the bucket, sure, but the water height will still vary in a paraboloidal fashion - just a very off-center paraboloid. This is because the apparent gravity points to the center of rotation, which is at a slightly different angle to a straight wall at different points.John Mcrain said:What casuse this U shape when bucket is at wall in Groom?
I assume by apparent gravity you mean the combination of Earths gravity and centrifugal force. Its direction varies because the centrifugal force is non-uniform.Ibix said:What do you mean by "U shape"? Once the rotation axis is outside the bucket there will no longer be a dip in the middle of the bucket, sure, but the water height will still vary in a paraboloidal fashion - just a very off-center paraboloid. This is because the apparent gravity points to the center of rotation, which is at a slightly different angle to a straight wall at different points.
Yes, agreed. I was just wondering if OP somehow thinks that we think all buckets everywhere on a rotating disk will have a minimum surface height somewhere in the middle of each bucket ("a U shape"), rather than having a small (typically off-center) part of a shared paraboloid.A.T. said:I assume by apparent gravity you mean the combination of Earths gravity and centrifugal force. Its direction varies because the centrifugal force is non-uniform.
You mean like this?Ibix said:Yes, agreed. I was just wondering if OP somehow thinks that we think all buckets everywhere on a rotating disk will have a minimum surface height somewhere in the middle of each bucket ("a U shape"), rather than having a small (typically off-center) part of a shared paraboloid.
Yes.John Mcrain said:You mean like this?
It'll do a lot of sloshing around and quite possibly knock over the bucket. If it doesn't fall over the forces on the water from the decelerating bucket walls will move the rotation axis of the water to somewhere near the niddle of the bucket, yes.John Mcrain said:If we stop rotate G-room when bucket is at the wall, water in bucket will still spin some time.
No. Fill the room with water to a depth of a few centimetres and spin it up. The water will settle down into a paraboloid with a minimum at the rotation axis and no bulk motion of the water as seen from the room. Place a hollow cylinder, open at the ends, vertically in the water at some point. You've just made a filled bucket. Now drain all the water from everywhere except the bucket you just made. Why would the water level change? The forces on it never do.John Mcrain said:Does it mean we must add together effects of water rotation(U shape) plus effects (parabolid shape ) of water circle around Groom pivot point, when G-room is rotate?
Yes if the water is at rest in the rotating frame, it has some angular momentum around the center of the bucket.John Mcrain said:You mean like this?
View attachment 320213
If we stop rotate G-room when bucket is at the wall, water in bucket will still spin some time. That is proof that water also rotate about itself during G-room rotation.
You can (but don't have to) decompose the inertial forces like that. But the effect of the circular translation is not a parabolid shape, because the inerial froce field from the centripetal acceleration is uniform. It's a choice of reference frame origin:John Mcrain said:Does it mean we must add together effects of water rotation(U shape) plus effects (parabolid shape ) of water circle around Groom pivot point, when G-room is rotate?
in left case bucket is facing allways toward center (roation+circualr motion) and in right case bucket point for example allways to north(only circular motion)Ibix said:No.
How can centripetal acceleration be uniform, before you write centrifugal is non-uniform?A.T. said:Yes if the water is at rest in the rotating frame, it has some angular momentum around the center of the bucket.You can (but don't have to) decompose the inertial forces like that. But the effect of the circular translation is not a parabolid shape, because the inerial froce field from the centripetal acceleration is uniform. It's a choice of reference frame origin:
A) In the co-rotating frame with origin at the center of the room, you have the following inertial forces:
- Centrifugal force radially away from the room-center (due to rotation of the reference frame axes)
B) In the co-rotating frame with origin at the center of the bucket, you have the following inertial forces:
- Centrifugal force radially away from the bucket-center (due to rotation of the reference frame axes)
- Uniform inertial force parallel to the line connecting the room-center and the bucket-center (due to non-inertial translation of the frame origin)
The sum of the two fields in B must equal the centrifugal force in A. In both frames the water is at rest, so there are no Coriolis forces.
- Inertial centrifugal force due to rotation of the reference frame axes is non-uniformJohn Mcrain said:How can centripetal acceleration be uniform, before you write centrifugal is non-uniform?
Along radius centripetal and centrifugal force must change,so they are non-uniform?
But @Ibix write this : Fill the room with water to a depth of a few centimetres and spin it up. The water will settle down into a paraboloid with a minimum at the rotation axis and no bulk motion of the water as seen from the room.A.T. said:- Inertial force due to non-inertial translation of the reference frame origin is uniform
John Mcrain said:But @Ibix write this : Fill the room with water to a depth of a few centimetres and spin it up. The water will settle down into a paraboloid with a minimum at the rotation axis and no bulk motion of the water as seen from the room.
dont understand
Read the full post #31 again. In frame B the inertial force has two components, one is non-uniform and one is uniform. The total inertial force in frame B is non-uniform and the same as in frame A.John Mcrain said:@A.T.
Outside and inside edge of bucket dont have same velocity and they are not at same place of room radius.
How can that force be uniform?
So, in one case you've got a bucket on a large turntable and in the other a bucket on a small turntable that is on the large turntable, with spins synchronised so that the bucket maintains its orientation with respect to the lab? I wouldn't expect the behaviour to be the same, except in certain highly idealised cases, due to different frictional forces between the bucket and the water. I wasn't aware you were considering a turntable on a turntable before now.John Mcrain said:How can then water in bucket behaves the same in this two cases?
No I didnt consider turntable on turntable in orignal case, I add this example only to point out that rotation of water about itself ( synchronised spin) must have some effect to water shape not just bucket water that is cirlce around pivot point of Groom.Ibix said:I wasn't aware you were considering a turntable on a turntable before now.
I still dont understand why both effects are not non-uniform, why in frame of bucket ,cirlce motion around center of Groom is uniform?A.T. said:Read the full post #31 again. In frame B the inertial force has two components, one is non-uniform and one is uniform. The total inertial force in frame B is non-uniform and the same as in frame A.
If you don't get it then stick to frame A. I only mentioned B because you asked if one can decompose the inertial effects like that.
The uniform component is the most basic inertial force due to non-inertial translation of the coordinate system origin: F = -ma where a is the acceleration of the coordinate system origin relative to an inertial frame.John Mcrain said:I still dont understand why both effects are not non-uniform, why in frame of bucket ,cirlce motion around center of Groom is uniform?
The water surface tends towards an equipotential surface in the rest frame of the water.John Mcrain said:in left case bucket is facing allways toward center (roation+circualr motion) and in right case bucket point for example allways to north(only circular motion)
How can then water in bucket behaves the same in this two cases?
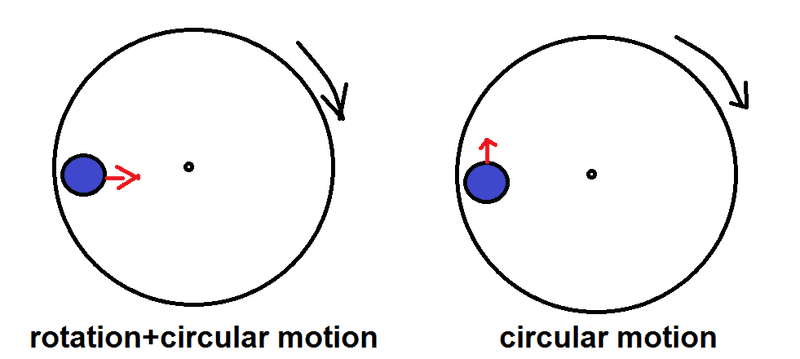
So in short,pure orbiting cause tilited flat surface(like in straight line acceleration-dragster) and when we add rotation around itself, then we get tilted curved surface?A.T. said:The water surface tends towards an equipotential surface in the rest frame of the water.
Left the equipotential surface in the rotating rest frame of the bucket & water is curved and part of a paraboloid centered on the turn table center.
Right the equipotential surface in the non-rotating but orbiting rest frame of the bucket & water is flat but tilted inwards.
The problem with the right case is that the equipotential surface is not static in the rest frame of the water & bucket, so the water has to flow to follow the equipotential surface. This will lag behind, and create friction with the walls. But ignoring those complications the water surface on the right tends towards a tilted flat plane.
Yes, for an idealized fluid that immediately adapts to a changing potential field.John Mcrain said:So in short,pure orbiting cause tilited flat surface(like in straight line acceleration-dragster) and when we add rotation around itself, then we get tilted curved surface?
In right case bucket rotate,change orientation in relation to G-room.When G-room stop rotate, water in bucket is calm,not spins.A.T. said:Yes, for an idealized fluid that immediately adapts to a changing potential field.
The two frames are not equivalent precisely because rotation is absolute.John Mcrain said:How can different frames get differnet results,isnt rotation absolute?
It's defined relative to inertial frames.John Mcrain said:Or why would rotation be defined to ground frame?
So I can say moon not change direction in relation to Earth,always same side point toward center of earth?A.T. said:The two frames are not equivalent precisely because rotation is absolute.
It's defined relative to inertial frames.
That is ambiguous / not clear.John Mcrain said:So I can say moon not change direction in relation to Earth,
That is correct.John Mcrain said:always same side point toward center of earth?