Rotational motion and finding the moment of inertia

[Bolter]

Homework Statement

Find the moment of inertia

Relevant Equations

PE = mgh
KE tran = 1/2mv^2

Here is the problem that I am finding difficult to answer

I had tried using conservation of energy to do this question

Where I know that the gravitational potential energy at the top of the slope equals to the sum of both the linear and rotational kinetic energy at the bottom of the slope.

Hence which is why I written down this, in where I then rearrange for I (moment of inertia). However upon doing this, I realize I have an unknown which is omega (angular velocity). How would I work out omega so I then find the moment of inertia of the ball?

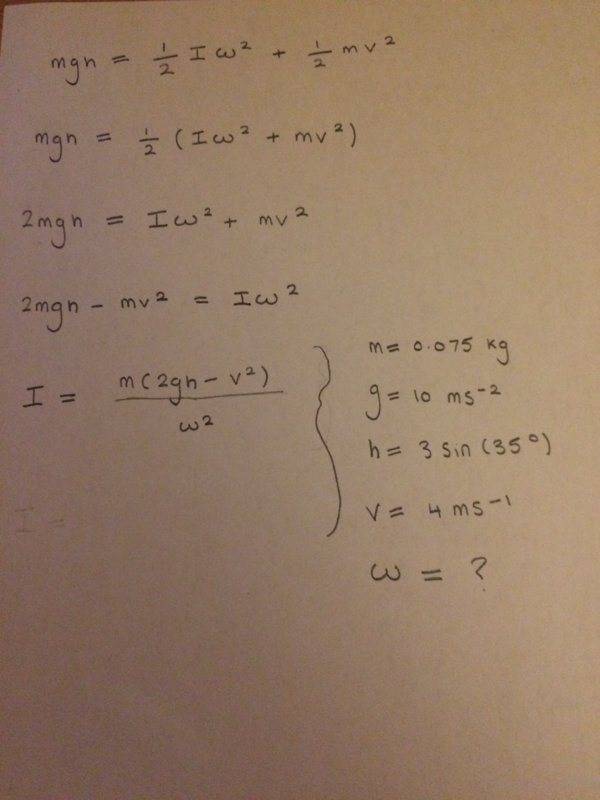
Thank you!

 

[BvU]

You make use of the given that 'the ball rolled' (i.e. it did not slip) !

 

[Bolter]

You make use of the given that 'the ball rolled' (i.e. it did not slip) !

So is finding angular velocity as simple as dividing the linear velocity by the ball's radius
I have done that to get my angular velocity to be 266.667 rad/s

Then plugging in all the values I need from before, I get I = 1.94 x 10^-5 kg/m^2 (3 sig figs)

Is this alright?

 

[haruspex]

So is finding angular velocity as simple as dividing the linear velocity by the ball's radius
I have done that to get my angular velocity to be 266.667 rad/s

Then plugging in all the values I need from before, I get I = 1.94 x 10^-5 kg/m^2 (3 sig figs)

View attachment 254367

Is this alright?

Looks right.

 

[Bolter]

Looks right.

Thanks for checking. Appreciate it!

 

[BvU]

I agree with your result. There is one snag, however:
For a massive sphere, $I = {2\over 5}\,mr^2$; for a hollow sphere, $I = {2\over 3}\,mr^2$. Most you can have is $I = mr^2$ (all the mass at distance $r$, like for a ring).

Our $I = 1.94 \ 10^{-5}$ kg m² would be 1.15 $mr^2\qquad$

I suspect an error by the exercise composer ...

 

[Bolter]

I agree with your result. There is one snag, however:
For a massive sphere, $I = {2\over 5}\,mr^2$; for a hollow sphere, $I = {2\over 3}\,mr^2$. Most you can have is $I = mr^2$ (all the mass at distance $r$, like for a ring).

Our $I = 1.94 \ 10^{-5}$ kg m² would be 1.15 $mr^2\qquad$

I suspect an error by the exercise composer ...

Ah yes that is true. I’ll definitely take note of that :)