- #1
nate13
- 1
- 0
I'm having problems with this:
Jed has two resistors, R1 = 120.0 ± 0.30 Ω and R2 = 65.6 ± 0.15 Ω. Give all answers to 2 significant digits.
(a) Calculate the RSS uncertainty for the resistance of these two resistors in series.
(b) Calculate the RSS uncertainty for the resistance of these two resistors in parallel.
(c) Compare the relative uncertainty (in percent) for the two cases.
This is what I've tried so far for part a, but i know it's wrong:
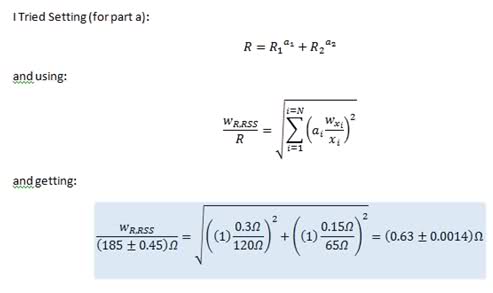
Any help is appreciated!
Jed has two resistors, R1 = 120.0 ± 0.30 Ω and R2 = 65.6 ± 0.15 Ω. Give all answers to 2 significant digits.
(a) Calculate the RSS uncertainty for the resistance of these two resistors in series.
(b) Calculate the RSS uncertainty for the resistance of these two resistors in parallel.
(c) Compare the relative uncertainty (in percent) for the two cases.
This is what I've tried so far for part a, but i know it's wrong:
Any help is appreciated!