S=u*t+a*t^2/2 VS ratio 1:2:3:5... t1/t10= ...

[Steels]

Homework Statement

Galileo Ratio? But why squeroot? what am i missing?

Relevant Equations

s=u*t+a*t^2/2 VS 1:2:3:... t1/t10=s1/s10?

Hello! I can do this task just using this formula s=u*t+a*t^2/2.
But just saw an example where someone used this Galileo toerem.
Here is task:
"An observer stands on the platform at the front edge of the first bogie of a stationary train. The train starts moving with uniform acceleration and the first bogie takes 5 seconds to cross the observer. If all the bogies of the train are of equal length and the gap between them is negligible, the time taken by the tength bogie to cross observer is?"
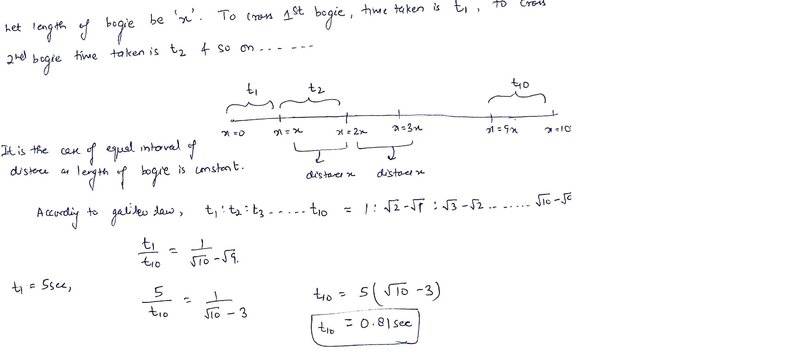
Now please, if you look at picture, you see its t1/t10=1/(sqrt(10)-sqrt(9))My question is, why its in squeroot? Where do we get x^2? so to get squeroot we need x^2! but from what? Why the lenght, it its meter it should be as meter, so where we get m^2? What am i doing wrong. Thanks for any explenation!

 

[BvU]

hllo @Steels ,

$\qquad$ !​

You have $$ s = {1\over 2}at^2$$so$$t = \sqrt{s\over 2a}$$and you are given that $t_1 = 5$ sec is the time for the first car to pass the observer.

If a car has length $l$, you know that $\sqrt{l\over 2a}= 5$ sec.

And the exercise asks for $\sqrt{10 {l\over 2a}} - \sqrt{9 {l\over 2a}} = \left (\sqrt{10 } - \sqrt{9} \right ) {l\over 2a}$ which, as you can see has the dimension of time.

The 10 and the 9 are numbers, no dimension.

$\$

 

[kuruman]

If a car has length $l$, you know that $\sqrt{l\over 2a}= 5$ sec.

Ahem, $$L=\dfrac{1}{2}at^2 \implies t^2=\dfrac{2L}{a} \implies t=\sqrt{\dfrac{2L}{a} }.$$ Also $\sqrt{\frac{L}{a}}$ has dimensions of time.

 

[BvU]

Oops, thank you !

 

[Steve4Physics]

... the time taken by the tength bogie to cross observer is?

Ten (or more) bogies? That’s a bogie wonderland! (If puzzled, give yourself a treat and click here.)

By the way - 'tenth' not 'tength'!

 

[Steels]

Muchas love for explanation! How could i miss that.
Thank you all for replys! Have a nice day to you all!

 

[BvU]

Hold it ! Had no idea what a bogie is; turns out the tenth bogie may well be at he end of the fifth railway car, not the tenth ! Unless we are talking Indian English ...

The term "car" is commonly used by itself in American English when a rail context is implicit. Indian English sometimes uses "bogie" in the same manner,[1]

(but then, who trusts google...)

The exercise becomes pretty impossible if bogie $\ne$ railcar ...

$\$

 

[Steels]

Hold it ! Had no idea what a bogie is; turns out the tenth bogie may well be at he end of the fifth railway car, not the tenth ! Unless we are talking Indian English ...

(but then, who trusts google...)

The exercise becomes pretty impossible if bogie $\ne$ railcar ...

$\$

No, it's a rail wagon= railcar. bogie is meant the same as wagon.

 

[BvU]

Ok, a bogie is a railcar -- keeps things manageable . But let's try to use English English for our communication ... wagon it is.

In response to a private conversation:

... i tried that later and i failed! ..
1st IMG _0370 picture, i got same result with just using physics method.

But in 2nd IMG_0371picture is what you sent me this morning! That mathematic ratio stuff.
And i am wondering about that part, where does that go!
Please, take a look at pictures, especially at 0371. what am i doing wrong by doing all that algebra stuff? Maybe wrong shortening?

IMG_0370:

IMG_0371:

$\$

We want to do this in the actual thread, not in private conversation: that way, others can correct any mistakes (which has already occurred at least once !)​

​

Also, we should type our equations using $\LaTeX$ for legibility​

​

Re IMG_0371:
The exercise asks for

the time taken by the tength bogie to cross observer

So that is

the time from $t=0$ until the tenth wagon has passed the observer (let's call this $t_{10}$)​

minus

the time from $t=0$ until the ninth wagon has passed the observer (let's call this $t_{9}$)​

(Note that $t_{10}$ is defined differently here: with these definitions, the answer to the exercise is $t_{10}-t_9$ !)

And we were given

the time from $t=0$ until the first wagon has passed the observer (let's call this $t_{1}$, 5 seconds)​

Let me correct my mistake(s) in #2

If a car has length $l,$ you know that $\sqrt{l\over 2a}= 5$ sec.

(using lower case $l$ was one, $l\over 2a$ instead of $2l\over a$ the other and kuruman caught both !)

If one wagon has length $L$, we can write$$
\begin{align*} t_1 &= \sqrt{\dfrac{2}{a} \,L} \\ t_{10} &= \sqrt{\dfrac{2}{a} \,10 L} \\ t_9 &= \sqrt{\dfrac{2}{a} \,9L} \end{align*}
$$so that our exercise answer becomes $$
\begin{align*} t_{10}-t_9 &=\sqrt{\dfrac{2}{a} \,10 L} \ - \sqrt{\dfrac{2}{a} \,9 L} \\
&=\sqrt{\dfrac{2}{a} \, L}\ \sqrt {10\;\vphantom{\dfrac{1}{1}}} \ - \sqrt{\dfrac{2}{a} \, L} \ \sqrt {9\;\vphantom{\dfrac{1}{1}}} \\
&= \sqrt{\dfrac{2}{a} \, L} \ \left (\sqrt {10\;} - \sqrt {9\;} \right ) \\
&=t_1 \left (\sqrt {10} - \sqrt 9 \right ) \end{align*} $$

Does this clarify things a little ?

$\$