Scale factors in spherical coordinates

Thread starter Est120
Start date May 29, 2021
Tags

Coordinates Factors Scale Spherical Spherical coordinates

In summary, scale factors in spherical coordinates are values that are multiplied to coordinate values to transform them from one coordinate system to another. There are three scale factors in spherical coordinates: r, θ, and φ, which correspond to the changes in radial distance, polar angle, and azimuthal angle. These scale factors can be calculated using specific equations and are important in understanding the relationship between different coordinate systems and determining volume elements. They are also affected by changes in coordinates, resulting in different transformations. Scale factors can be negative if the coordinate system is flipped or reflected, but their absolute values remain the same and represent the rates of change for each coordinate direction.

May 29, 2021

Est120

Homework Statement: how they get that result

Relevant Equations: Geometric relations

how they got that value for the scale factors h?

Physics news on Phys.org

May 29, 2021

dRic2

Gold Member

https://learn.lboro.ac.uk/archive/o...n2008/Workbook28/28_3_orthog_cvlnr_coords.pdf

see bottom of third page

1. What are scale factors in spherical coordinates?

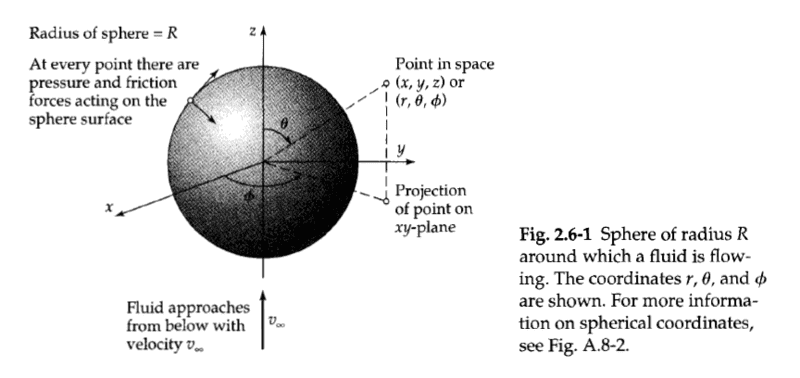
Scale factors in spherical coordinates are used to convert between different coordinate systems and represent the changes in distances and angles. They are also known as metric coefficients or Jacobian determinants.

2. How are scale factors calculated in spherical coordinates?

The scale factors in spherical coordinates are calculated using the partial derivatives of the spherical coordinates with respect to the Cartesian coordinates. The scale factors for radius, polar angle, and azimuthal angle are given by 1, r, and r sin(theta), respectively.

3. What is the significance of scale factors in spherical coordinates?

Scale factors in spherical coordinates play a crucial role in various fields of science and engineering, such as physics, geology, and astronomy. They help in understanding the relationships between different coordinate systems and in solving complex problems involving transformations between them.

4. How do scale factors affect the measurement of distances in spherical coordinates?

The scale factors in spherical coordinates affect the measurement of distances by accounting for the changes in the coordinate system. As the distance increases, the scale factors also increase, resulting in a larger measurement than in the original coordinate system.

5. Can scale factors be negative in spherical coordinates?

Yes, scale factors can be negative in spherical coordinates. This happens when the coordinate system is flipped or inverted, resulting in a change in the direction of the coordinate axes. However, the absolute value of the scale factor remains the same, and it is the sign that changes.